数据结构初阶：二叉树（二）

二叉树链式结构的实现

前置说明

在学习二叉树的基本操作前，需先要创建一棵二叉树，然后才能学习其相关的基本操作。由于现在对二叉树结构掌握还不够深入，为了降低学习成本，此处手动快速创建一棵简单的二叉树，快速进入二叉树操作学习，等二叉树结构了解的差不多时，我们反过头再来研究二叉树真正的创建方式。 
typedef int BTDataType;
typedef struct BinaryTreeNode
{BTDataType data;struct BinaryTreeNode* left;struct BinaryTreeNode* right;
}TreeNode;TreeNode* BuyTreeNode(int x)
{TreeNode* node = (TreeNode*)malloc(sizeof(TreeNode));assert(node);node->data = x;node->left = NULL;node->right = NULL;return node;
}TreeNode* CreateTree()
{TreeNode* node1 = BuyTreeNode(1);TreeNode* node2 = BuyTreeNode(2);TreeNode* node3 = BuyTreeNode(3);TreeNode* node4 = BuyTreeNode(4);TreeNode* node5 = BuyTreeNode(5);TreeNode* node6 = BuyTreeNode(6);TreeNode* node7 = BuyTreeNode(7);node1->left = node2;node1->right = node4;node2->left = node3;node4->left = node5;node4->right = node6;node5->right = node7;return node1;
}
注意：上述代码并不是创建二叉树的方式，真正创建二叉树方式后面详解重点讲解。
再看二叉树基本操作前，再回顾下二叉树的概念， 二叉树是：
1. 空树
2. 非空：根节点，根节点的左子树、根节点的右子树组成的。
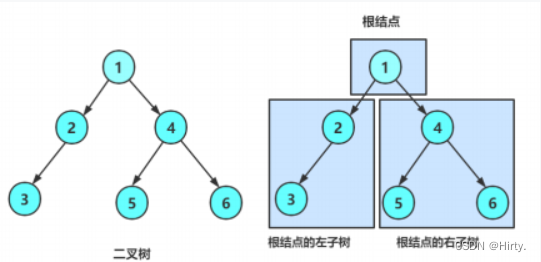
从概念中可以看出，二叉树定义是递归式的，因此后面基本操作中基本都是按照该概念实现的。

二叉树的遍历

前序、中序以及后序遍历

学习二叉树结构，最简单的方式就是遍历。所谓 二叉树遍历 (Traversal) 是按照某种特定的规则，依次对二叉 树中的节点进行相应的操作，并且每个节点只操作一次 。访问结点所做的操作依赖于具体的应用问题。 遍历是二叉树上最重要的运算之一，也是二叉树上进行其它运算的基础。
按照规则，二叉树的遍历有： 前序 / 中序 / 后序的递归结构遍历 ：
1. 前序遍历 (Preorder Traversal 亦称先序遍历 )—— 访问根结点的操作发生在遍历其左右子树之前。 （根 左子树 右子树）
2. 中序遍历 (Inorder Traversal)——访问根结点的操作发生在遍历其左右子树之中（间）。
（左子树 根 右子树）
3. 后序遍历 (Postorder Traversal)—— 访问根结点的操作发生在遍历其左右子树之后。
（左子树 右子树 根）
由于被访问的结点必是某子树的根， 所以 N(Node ）、 L(Left subtree ）和 R(Right subtree ）又可解释为 根、根的左子树和根的右子树 。 NLR 、 LNR 和 LRN 分别又称为先根遍历、中根遍历和后根遍历。 
// 二叉树前序遍历 
void PrevOrder(TreeNode* root)
{if (root == NULL){printf("N ");return;}printf("%d ", root->data);PrevOrder(root->left);PrevOrder(root->right);
}
// 二叉树中序遍历
void InOrder(TreeNode* root)
{if (root == NULL){printf("N ");return;}InOrder(root->left);printf("%d ", root->data);InOrder(root->right);
}
// 二叉树后序遍历
void PostOrder(TreeNode* root)
{if (root == NULL){printf("N ");return;}PostOrder(root->left);PostOrder(root->right);printf("%d ", root->data);
}
下面分析前序递归遍历，中序与后序图解类似：
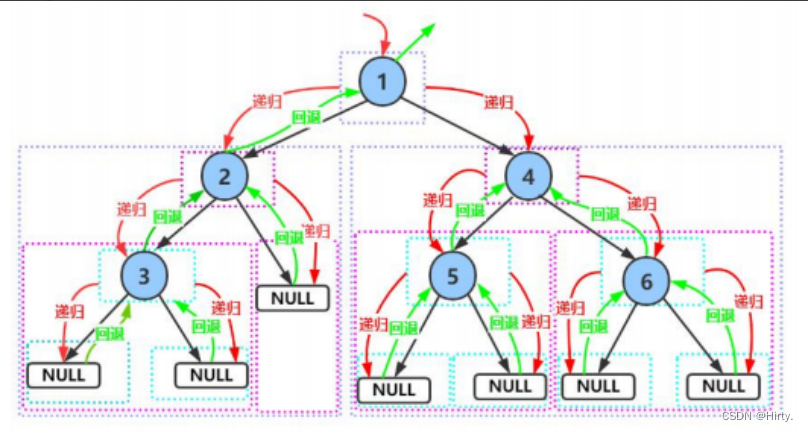
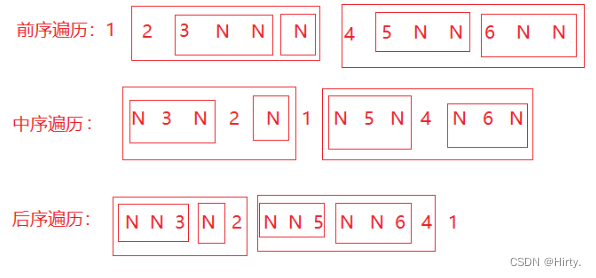
前序遍历结果： 1 2 3 4 5 6
中序遍历结果： 3 2 1 5 4 6
后序遍历结果： 3 2 5 6 4 1

层序遍历

层序遍历 ：除了先序遍历、中序遍历、后序遍历外，还可以对二叉树进行层序遍历。设二叉树的根节点所在层数为1 ，层序遍历就是从所在二叉树的根节点出发，首先访问第一层的树根节点，然后从左到右访问第 2 层上的节点，接着是第三层的节点，以此类推，自上而下，自左至右逐层访问树的结点的过程就是层序遍历。
原理：上一层出会依次带入下一层
队列：先进先出
一行打印完数据： 
void LevelOrder(TreeNode* root)
{Queue q;QueueInit(&q);if (root)QueuePush(&q, root);while (!QueueEmpty(&q)){TreeNode* front = QueueFront(&q);QueuePop(&q);printf("%d ", front->data);if (front->left)QueuePush(&q, front->left);if (front->right)QueuePush(&q, front->right);}}printf("\n");QueueDestroy(&q);
}
一层一层打印数据： 
void LevelOrder(TreeNode* root)
{Queue q;QueueInit(&q);if (root)QueuePush(&q, root);int levelSize = 1;while (!QueueEmpty(&q)){// 一层一层出数据while (levelSize--){TreeNode* front = QueueFront(&q);QueuePop(&q);printf("%d ", front->data);if (front->left)QueuePush(&q, front->left);if (front->right)QueuePush(&q, front->right);}printf("\n");levelSize = QueueSize(&q);}printf("\n");QueueDestroy(&q);
}

判断二叉树是否是完全二叉树：

// 判断二叉树是否是完全二叉树
bool TreeComplete(TreeNode* root)
{Queue q;QueueInit(&q);if (root)QueuePush(&q, root);int levelSize = 1;while (!QueueEmpty(&q)){TreeNode* front = QueueFront(&q);QueuePop(&q);if (front == NULL)break;QueuePush(&q, front->left);QueuePush(&q, front->right);}// 前面遇到空以后，后面还有非空就不是完全二叉树while (!QueueEmpty(&q)){TreeNode* front = QueueFront(&q);QueuePop(&q);if (front){QueueDestroy(&q);return false;}}QueueDestroy(&q);return true;
}

节点个数以及高度等

二叉树节点个数：

思路：分治子问题：左子树节点个数+右子树节点个数+1
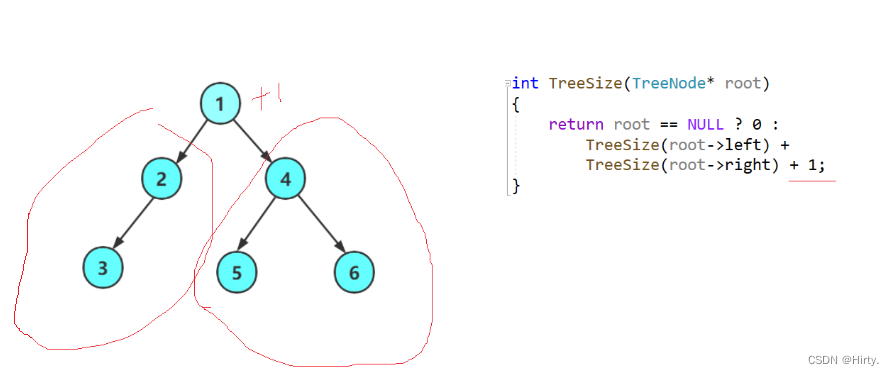
代码： 
// 二叉树节点个数
int TreeSize(TreeNode* root)
{return root == NULL ? 0 : TreeSize(root->left) + TreeSize(root->right) + 1;
}
二叉树叶子节点个数：
思路：
代码： 
// 叶子节点的个数
int TreeLeafSize(TreeNode* root)
{// 空 返回0if (root == NULL)return 0;// 不是空，是叶子 返回1if (root->left == NULL&& root->right == NULL)return 1;// 不是空 也不是叶子  分治=左右子树叶子之和return TreeLeafSize(root->left) +TreeLeafSize(root->right);
}

二叉树的高度：

思路;

代码： 
//int TreeHeight(TreeNode* root)
//{
//	if (root == NULL)
//		return 0;
//	int leftHeight = TreeHeight(root->left);
//	int rightHeight = TreeHeight(root->right);
//
//	return leftHeight > rightHeight ? leftHeight + 1 : rightHeight + 1;
//}int TreeHeight(TreeNode* root)
{if (root == NULL)return 0;return fmax(TreeHeight(root->left), TreeHeight(root->right)) + 1;
}

二叉树第k层节点个数

思路：

代码：

int TreeLevelK(TreeNode* root, int k)
{assert(k > 0);if (root == NULL)return 0;if (k == 1)return 1;return TreeLevelK(root->left, k-1)+ TreeLevelK(root->right, k-1);
}

二叉树查找值为x的结点：

// 二叉树查找值为x的结点
TreeNode* TreeFind(TreeNode* root, BTDataType x)
{if (root == NULL) //空树return NULL;if (root->data == x)return root;TreeNode* ret1 = TreeFind(root->left, x);if (ret1)return ret1;TreeNode* ret2 = TreeFind(root->right, x);if (ret2)return ret2;return NULL;
}

二叉树的创建和销毁

二叉树遍历_牛客题霸_牛客网 (nowcoder.com)
通过前序遍历的数组 "ABD##E#H##CF##G##" 构建二叉树： 
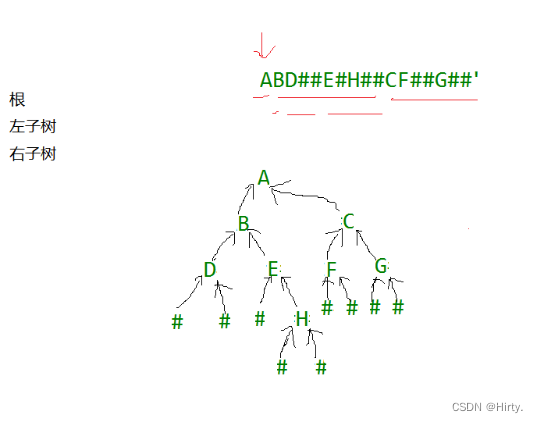
// 通过前序遍历的数组"ABD##E#H##CF##G##"构建二叉树
TreeNode* TreeCreate(char* a, int* pi)
{if (a[*pi] == '#'){(*pi)++;return NULL;}TreeNode* root = (TreeNode*)malloc(sizeof(TreeNode));if (root == NULL){perror("malloc fail");exit(-1);}root->data = a[(*pi)++];root->left = TreeCreate(a, pi);root->right = TreeCreate(a, pi);return root;
}

二叉树销毁 :

后序遍历

void DestroyTree(TreeNode* root)
{if (root == NULL)return;DestroyTree(root->left);DestroyTree(root->right);free(root);
}

二叉树的实现（对上诉总结）

Test.c


#include"Queue.h"typedef int BTDataType;
typedef struct BinaryTreeNode
{BTDataType data;struct BinaryTreeNode* left;struct BinaryTreeNode* right;
}TreeNode;TreeNode* BuyTreeNode(int x)
{TreeNode* node = (TreeNode*)malloc(sizeof(TreeNode));assert(node);node->data = x;node->left = NULL;node->right = NULL;return node;
}TreeNode* CreateTree()
{TreeNode* node1 = BuyTreeNode(1);TreeNode* node2 = BuyTreeNode(2);TreeNode* node3 = BuyTreeNode(3);TreeNode* node4 = BuyTreeNode(4);TreeNode* node5 = BuyTreeNode(5);TreeNode* node6 = BuyTreeNode(6);TreeNode* node7 = BuyTreeNode(7);node1->left = node2;node1->right = node4;node2->left = node3;node4->left = node5;node4->right = node6;node2->right = node7;return node1;
}void PrevOrder(TreeNode* root)
{if (root == NULL){printf("N ");return;}printf("%d ", root->data);PrevOrder(root->left);PrevOrder(root->right);
}void InOrder(TreeNode* root)
{if (root == NULL){printf("N ");return;}InOrder(root->left);printf("%d ", root->data);InOrder(root->right);
}void PostOrder(BTNode* root)
{if (root == NULL){printf("N ");return;}PostOrder(root->left);PostOrder(root->right);printf("%d ", root->data);
}
int TreeSize(TreeNode* root)
{return root == NULL ? 0 : TreeSize(root->left) +TreeSize(root->right) + 1;
}// 叶子节点的个数
int TreeLeafSize(TreeNode* root)
{// 空 返回0if (root == NULL)return 0;// 不是空，是叶子 返回1if (root->left == NULL&& root->right == NULL)return 1;// 不是空 也不是叶子  分治=左右子树叶子之和return TreeLeafSize(root->left) +TreeLeafSize(root->right);
}//int TreeHeight(TreeNode* root)
//{
//	if (root == NULL)
//		return 0;
//	int leftHeight = TreeHeight(root->left);
//	int rightHeight = TreeHeight(root->right);
//
//	return leftHeight > rightHeight ? leftHeight + 1 : rightHeight + 1;
//}int TreeHeight(TreeNode* root)
{if (root == NULL)return 0;return fmax(TreeHeight(root->left), TreeHeight(root->right)) + 1;
}int TreeLevelK(TreeNode* root, int k)
{assert(k > 0);if (root == NULL)return 0;if (k == 1)return 1;return TreeLevelK(root->left, k-1)+ TreeLevelK(root->right, k-1);
}// 二叉树查找值为x的结点
TreeNode* TreeFind(TreeNode* root, BTDataType x)
{if (root == NULL)return NULL;if (root->data == x)return root;TreeNode* ret1 = TreeFind(root->left, x);if (ret1)return ret1;TreeNode* ret2 = TreeFind(root->right, x);if (ret2)return ret2;return NULL;
}
// 通过前序遍历的数组"ABD##E#H##CF##G##"构建二叉树
TreeNode* TreeCreate(char* a, int* pi)
{if (a[*pi] == '#'){(*pi)++;return NULL;}TreeNode* root = (TreeNode*)malloc(sizeof(TreeNode));if (root == NULL){perror("malloc fail");exit(-1);}root->data = a[(*pi)++];root->left = TreeCreate(a, pi);root->right = TreeCreate(a, pi);return root;
}void DestroyTree(TreeNode* root)
{if (root == NULL)return;DestroyTree(root->left);DestroyTree(root->right);free(root);
}void LevelOrder(TreeNode* root)
{Queue q;QueueInit(&q);if (root)QueuePush(&q, root);int levelSize = 1;while (!QueueEmpty(&q)){// 一层一层出while (levelSize--){TreeNode* front = QueueFront(&q);QueuePop(&q);printf("%d ", front->data);if (front->left)QueuePush(&q, front->left);if (front->right)QueuePush(&q, front->right);}printf("\n");levelSize = QueueSize(&q);}printf("\n");QueueDestroy(&q);
}// 判断二叉树是否是完全二叉树
bool TreeComplete(TreeNode* root)
{Queue q;QueueInit(&q);if (root)QueuePush(&q, root);int levelSize = 1;while (!QueueEmpty(&q)){TreeNode* front = QueueFront(&q);QueuePop(&q);if (front == NULL)break;QueuePush(&q, front->left);QueuePush(&q, front->right);}// 前面遇到空以后，后面还有非空就不是完全二叉树while (!QueueEmpty(&q)){TreeNode* front = QueueFront(&q);QueuePop(&q);if (front){QueueDestroy(&q);return false;}}QueueDestroy(&q);return true;
}int main()
{TreeNode* root = CreateTree();PrevOrder(root);printf("\n");InOrder(root);printf("\n");/*size = 0;TreeSize(root);printf("TreeSize:%d\n", size);size = 0;TreeSize(root);printf("TreeSize:%d\n", size);size = 0;TreeSize(root);printf("TreeSize:%d\n", size);*/printf("TreeSize:%d\n", TreeSize(root));printf("TreeSize:%d\n", TreeSize(root));printf("TreeSize:%d\n", TreeSize(root));printf("TreeLeafSize:%d\n", TreeLeafSize(root));printf("TreeHeight:%d\n", TreeHeight(root));printf("TreeLevelK:%d\n", TreeLevelK(root, 4));TreeNode* ret = TreeFind(root, 5);printf("TreeFind:%p\n", ret);//ret->data++;printf("TreeComplete:%d\n", TreeComplete(root));//PrevOrder(root);//printf("\n");//LevelOrder(root);DestroyTree(root);root = NULL;return 0;
}

Queue.h

注意：int 改成 struct BinaryTreeNode* 

即typedef struct BinaryTreeNode* QDataType;

#pragma once
#include<stdio.h>
#include<assert.h>
#include<stdlib.h>
#include<stdbool.h>
#include<math.h>typedef struct BinaryTreeNode* QDataType;
typedef struct QueueNode
{QDataType val;struct QueueNode* next;
}QNode;typedef struct Queue
{QNode* phead;QNode* ptail;int size;
}Queue;void QueueInit(Queue* pq);
void QueueDestroy(Queue* pq);
void QueuePush(Queue* pq, QDataType x);
void QueuePop(Queue* pq);
QDataType QueueFront(Queue* pq);
QDataType QueueBack(Queue* pq);
bool QueueEmpty(Queue* pq);
int QueueSize(Queue* pq);

Queue.c

#include"Queue.h"void QueueInit(Queue* pq)
{assert(pq);pq->phead = pq->ptail = NULL;pq->size = 0;
}void QueueDestroy(Queue* pq)
{assert(pq);QNode* cur = pq->phead;while (cur){QNode* next = cur->next;free(cur);cur = next;}pq->phead = pq->ptail = NULL;pq->size = 0;
}void QueuePush(Queue* pq, QDataType x)
{assert(pq);QNode* newnode = (QNode*)malloc(sizeof(QNode));if (newnode == NULL){perror("malloc fail");return;}newnode->val = x;newnode->next = NULL;if (pq->ptail == NULL){pq->ptail = pq->phead = newnode;}else{pq->ptail->next = newnode;pq->ptail = newnode;}pq->size++;
}void QueuePop(Queue* pq)
{assert(pq);// assert(pq->phead);QNode* del = pq->phead;pq->phead = pq->phead->next;free(del);del = NULL;if (pq->phead == NULL)pq->ptail = NULL;pq->size--;
}QDataType QueueFront(Queue* pq)
{assert(pq);// assert(pq->phead);return pq->phead->val;
}QDataType QueueBack(Queue* pq)
{assert(pq);// assert(pq->ptail);return pq->ptail->val;
}bool QueueEmpty(Queue* pq)
{assert(pq);return pq->phead == NULL;
}int QueueSize(Queue* pq)
{assert(pq);return pq->size;
}

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：http://www.rhkb.cn/news/313375.html

如若内容造成侵权/违法违规/事实不符，请联系长河编程网进行投诉反馈email:809451989@qq.com，一经查实，立即删除！

相关文章

学习Rust的第5天：控制流

学习Rust的第5天：控制流

Control flow, as the name suggests controls the flow of the program, based on a condition. 控制流&#xff0c;顾名思义&#xff0c;根据条件控制程序的流。 If expression If表达式 An if expression is used when you want to execute a block of code if a condition …
阅读更多...
list基础知识

list基础知识

list 1.list 的定义和结构 list 是双向链表&#xff0c;是C的容器模板&#xff0c;其接收两个参数&#xff0c;即 list(a,b) 其中 a 表示指定容器中存储的数据类型&#xff0c;b 表示用于分配器内存的分配器类型&#xff0c;默认为 list <int>; list 的特点&#xff1a;…
阅读更多...
springboot中mongodb连接池配置-源码分析

springboot中mongodb连接池配置-源码分析

yml下spring.data.mongodb 以前mysql等在spring.xxx下配置&#xff0c;现在springboot新版本&#xff08;小编3.2.3&#xff09;在spring.data.xxx下了&#xff0c;如下所示&#xff0c;mongodb的配置在spring.data.mongodb下&#xff1a; 连接池相关参数配置-源码分析 拼接在…
阅读更多...
STM32应用开发——BH1750光照传感器详解

STM32应用开发——BH1750光照传感器详解

STM32应用开发——BH1750光照传感器详解 目录 STM32应用开发——BH1750光照传感器详解前言1 硬件介绍1.1 BH1750简介1.2 硬件接线 2 软件编程2.1 软件原理2.1.1 IIC设备地址2.1.2 IIC读写2.1.3 BH1750指令集2.1.4 BH1750工作流程2.1.5 BH1750测量模式 2.2 测试代码2.3 运行测试…
阅读更多...
学习了解大模型的四大缺陷

学习了解大模型的四大缺陷

由中国人工智能学会主办的第十三届吴文俊人工智能科学技术奖颁奖典礼暨2023中国人工智能产业年会于2024年4月14日闭幕。 会上&#xff0c;中国工程院院士、同济大学校长郑庆华认为&#xff0c;大模型已经成为当前人工智能的巅峰&#xff0c;大模型之所以强&#xff0c;是依托了…
阅读更多...
Java 设计模式系列：模板方法模式

Java 设计模式系列：模板方法模式

简介 模板方法模式是一种行为型设计模式&#xff0c;它定义一个操作中的算法骨架&#xff0c;将一些步骤推迟到子类中。模板方法模式使得子类可以不改变一个算法的结构&#xff0c;即可重定义该算法的某些特定步骤。 在模板方法模式中&#xff0c;抽象类中定义了一系列基本操…
阅读更多...
48.基于SpringBoot + Vue实现的前后端分离-雪具销售系统（项目 + 论文PPT）

48.基于SpringBoot + Vue实现的前后端分离-雪具销售系统（项目 + 论文PPT）

项目介绍 本站是一个B/S模式系统&#xff0c;采用SpringBoot Vue框架&#xff0c;MYSQL数据库设计开发&#xff0c;充分保证系统的稳定性。系统具有界面清晰、操作简单&#xff0c;功能齐全的特点&#xff0c;使得基于SpringBoot Vue技术的雪具销售系统设计与实现管理工作系统…
阅读更多...
精通技术写作：如何写出高质量技术文章？

精通技术写作：如何写出高质量技术文章？

CSDN 的朋友你们好&#xff0c;我是未来&#xff0c;今天给大家带来专栏【程序员博主教程&#xff08;完全指南&#xff09;】的第 7 篇文章“如何撰写高质量技术文章”。本文深入探讨了如何写好一篇技术文章。文章给出了好的技术文章的定义和分析&#xff0c;并提供了从选题、…
阅读更多...
MDK stm32怎么生成bin文件

MDK stm32怎么生成bin文件

第一种 D:\Keil_v5\ARM\ac5.6\bin\fromelf.exe --bin -o ../../Output/atk_f407.bin ../../Output/atk_f407.axf 空格解析 D:\Keil_v5\ARM\ac5.6\bin\fromelf.exe一个空格--bin一个空格-o两个空格../../Output/atk_f407.bin ../../Output/atk_f407.axf &#xff08;注意后…
阅读更多...
回归预测 | Matlab实现GWO-GPR灰狼算法优化高斯过程回归多变量回归预测

回归预测 | Matlab实现GWO-GPR灰狼算法优化高斯过程回归多变量回归预测

回归预测 | Matlab实现GWO-GPR灰狼算法优化高斯过程回归多变量回归预测 目录 回归预测 | Matlab实现GWO-GPR灰狼算法优化高斯过程回归多变量回归预测预测效果基本介绍程序设计参考资料 预测效果 基本介绍 Matlab实现GWO-GPR灰狼算法优化高斯过程回归多变量回归预测 1.Matlab实现…
阅读更多...
ASP.NET基于TCP协议的简单即时通信软件的设计与实现

ASP.NET基于TCP协议的简单即时通信软件的设计与实现

摘 要 即时通信(Instant Message)&#xff0c;由于其具有实时性、跨平台性、成本低、效率高等优点而受到广泛的使用。设计并实现一个能够处理多用户进行实时、安全的即时通信系统具有较强的现实意义。即时通信的底层通信是通过SOCKET套接字接口实现的。当前的主流UNIX系统和微…
阅读更多...
RocketMQ 之 IoT 消息解析：物联网需要什么样的消息技术？

RocketMQ 之 IoT 消息解析：物联网需要什么样的消息技术？

作者&#xff1a;林清山&#xff08;隆基&#xff09; 前言&#xff1a; 从初代开源消息队列崛起&#xff0c;到 PC 互联网、移动互联网爆发式发展&#xff0c;再到如今 IoT、云计算、云原生引领了新的技术趋势&#xff0c;消息中间件的发展已经走过了 30 多个年头。 目前&a…
阅读更多...
图神经网络推荐系统

图神经网络推荐系统

链接&#xff08;何向南教授&#xff09;&#xff1a;https://hexiangnan.github.io/papers/wsdm22-tutorial-proposal.pdf 摘要&#xff1a; 近年来&#xff0c;图神经网络(graph neural network, GNN)以其强大的结构化数据处理能力和对高阶信息的挖掘能力&#xff0c;成为许…
阅读更多...
【Go语言快速上手(二)】 分支与循环函数讲解

【Go语言快速上手(二)】 分支与循环函数讲解

&#x1f493;博主CSDN主页:杭电码农-NEO&#x1f493;   ⏩专栏分类:Go语言专栏⏪   &#x1f69a;代码仓库:NEO的学习日记&#x1f69a;   &#x1f339;关注我&#x1faf5;带你学习更多Go语言知识   &#x1f51d;&#x1f51d; Go快速上手 1. 前言2. 分支与循环2.1…
阅读更多...
libtorch+cuda12.0cmake编译失败“Failed to find nvToolsExt”解决办法

libtorch+cuda12.0cmake编译失败“Failed to find nvToolsExt”解决办法

博主已安装cuda12.0以及对应的libtorch。 这里可以直接通过安装cuda11.6&#xff08;cuda11.8也可以&#xff09;中的【Nsight NVTX】解决。 1、下载CUDA11.6 cuda下载链接 蓝色箭头根据电脑系统win10还是win11自行选择&#xff0c;其余均按图中所以选择。 2、安装 下载完…
阅读更多...
集合体系java

集合体系java

Collection:单列集合&#xff1a;每个元素只包含一个值 Collection集合存储的是地址 Collection的三种遍历方法如下 //迭代器是用来遍历集合的专用方式&#xff08;数组没有迭代器&#xff09;&#xff0c;在java中迭代器的代表是Iterator //boolean hasNext():询问当前位置…
阅读更多...
图书管理系统概述

图书管理系统概述

自友图书馆管理系统解决方案适用于中小学、大中专院校以及企事业单位中小型图书馆的自动化管理需求&#xff0c;其功能覆盖了图书馆自动化集成管理业务流程所包括的所有环节。《图书馆管理系统》首先应该按照我国图书馆行业通用CNMARC格式及《中图法第四版》行业标准开发而成,支…
阅读更多...
Swift Publisher 5 for mac：打造精美版面

Swift Publisher 5 for mac：打造精美版面

Swift Publisher 5 for mac&#xff1a;打造精美版面 Swift Publisher 5是一款专业的版面设计和编辑工具&#xff0c;为Mac用户提供了强大的设计功能和直观的操作界面。以下是关于Swift Publisher 5的功能介绍&#xff1a; 直观易用的界面&#xff1a;用户能够轻松地使用Swift …
阅读更多...
甘特图：项目管理者的必备神器，如何提高工作效率？

甘特图：项目管理者的必备神器，如何提高工作效率？

甘特图是什么&#xff1f;项目管理者大多都熟悉甘特图&#xff0c;它是一种直观展示项目计划执行过程的工具。通过条形图来显示项目、任务的时间安排&#xff0c;以及实际进度与计划进度的对比情况。 在我个人的项目管理实践中&#xff0c;甘特图确实帮助我提高了工作效率&am…
阅读更多...
goproxy 简单介绍 及一键安装脚本

goproxy 简单介绍 及一键安装脚本

goproxy 官网 https://goproxy.cn/ GoProxy 是一项用于 Go 模块的高性能代理服务&#xff0c;旨在为 Go 开发人员提供更快速、更可靠的模块下载体验。它提供以下主要功能&#xff1a; 全球分布式代理服务器: GoProxy 在全球多个地区部署了代理服务器&#xff0c;例如拉斯维加…
阅读更多...
最新文章
推荐文章

Copyright @ 2022~2023