代码随想录第十五天| ● 层序遍历 10 ● 226.翻转二叉树 ● 101.对称二叉树

文章目录

层序遍历
- 102. 二叉树的层序遍历
- - 思路一：递归
  - 思路二：层序遍历-迭代-借助队列
- 107. 二叉树的层序遍历 II
- - 思路：层序遍历后翻转数组result即可
- 199.二叉树的右视图
- - 思路：通过list数组储存每一层末尾值
- 637.二叉树的层平均值
- - 思路：通过list数组储存均值
- 429.N 叉树的层序遍历
- - 思路：依次判断并储存孩子节点
- 515.在每个树行中找最大值
- - 思路：每一层储存最大值Integer.MIN_VALUE
- 116.填充每个节点的下一个右侧节点指针
- - 思路
- 117.填充每个节点的下一个右侧节点指针II
- - 思路：【和前一题相同】
- 104.二叉树的最大深度
- - 思路：每层deep+1
- 111.二叉树的最小深度
- - 思路:
226.翻转二叉树
- 1.递归法DFS
- - 代码
- 2.层序遍历BFS
- - 代码：
101. 对称二叉树
- 思路：-使用后序遍历左右中
- - 递归法
  - 递归代码

层序遍历

层序遍历一个二叉树。就是从左到右一层一层的去遍历二叉树。这种遍历的方式和我们之前讲过的都不太一样。

需要借用一个辅助数据结构即队列来实现，队列先进先出，符合一层一层遍历的逻辑，而用栈先进后出适合模拟深度优先遍历也就是递归的逻辑。

而这种层序遍历方式就是图论中的广度优先遍历，只不过我们应用在二叉树上。

使用队列实现二叉树广度优先遍历，动画如在这里插入图片描述

102. 二叉树的层序遍历

在这里插入图片描述

思路一：递归

/*** Definition for a binary tree node.* public class TreeNode {*     int val;*     TreeNode left;*     TreeNode right;*     TreeNode() {}*     TreeNode(int val) { this.val = val; }*     TreeNode(int val, TreeNode left, TreeNode right) {*         this.val = val;*         this.left = left;*         this.right = right;*     }* }*/
class Solution {public List<List<Integer>> resList = new ArrayList<>();public List<List<Integer>> levelOrder(TreeNode root) {DFS(root,0);return resList;}//递归public void DFS(TreeNode root,Integer deep){if(root==null)return; //终止条件deep++;if(resList.size()<deep){//当层级增加时，list的Item也增加，利用list的索引值进行层级界定// 每一层一个item储存对应层的节点元素List<Integer> item = new ArrayList<>();resList.add(item);}resList.get(deep-1).add(root.val);//根据对应的层数储存节点DFS(root.left,deep);DFS(root.right,deep);}
}

思路二：层序遍历-迭代-借助队列

先建立【储存每一层的result数组】
【建立队列】保存每一层的树节点，方便取值。
将root放入队列中，队列不为空时进行循环。
建立每一层的item数组，储存数值
题解
在这里插入图片描述
代码：
注意： queue的size一定要提前固定，因为queue的大小是动态变化的

class Solution {public List<List<Integer>> levelOrder(TreeNode root) {Queue<TreeNode>queue = new LinkedList<>();List<List<Integer>> res = new ArrayList<>();if(root!=null){queue.add(root);//放入根节点}while(!queue.isEmpty()){// 新建一个临时列表 tmp ，用于存储当前层打印结果List<Integer> tmp=new ArrayList<>();// 队列放入每一层节点的个数// 循环次数为当前层节点数// // 这里一定要使用固定大小size，不要使用que.size()，因为que.size是不断变化的int len=queue.size();for(int i=len;i>0;i--){TreeNode node=queue.poll();tmp.add(node.val);if(node.left!=null){queue.add(node.left);}if(node.right!=null){queue.add(node.right);}}res.add(tmp);}return res;}
}

107. 二叉树的层序遍历 II

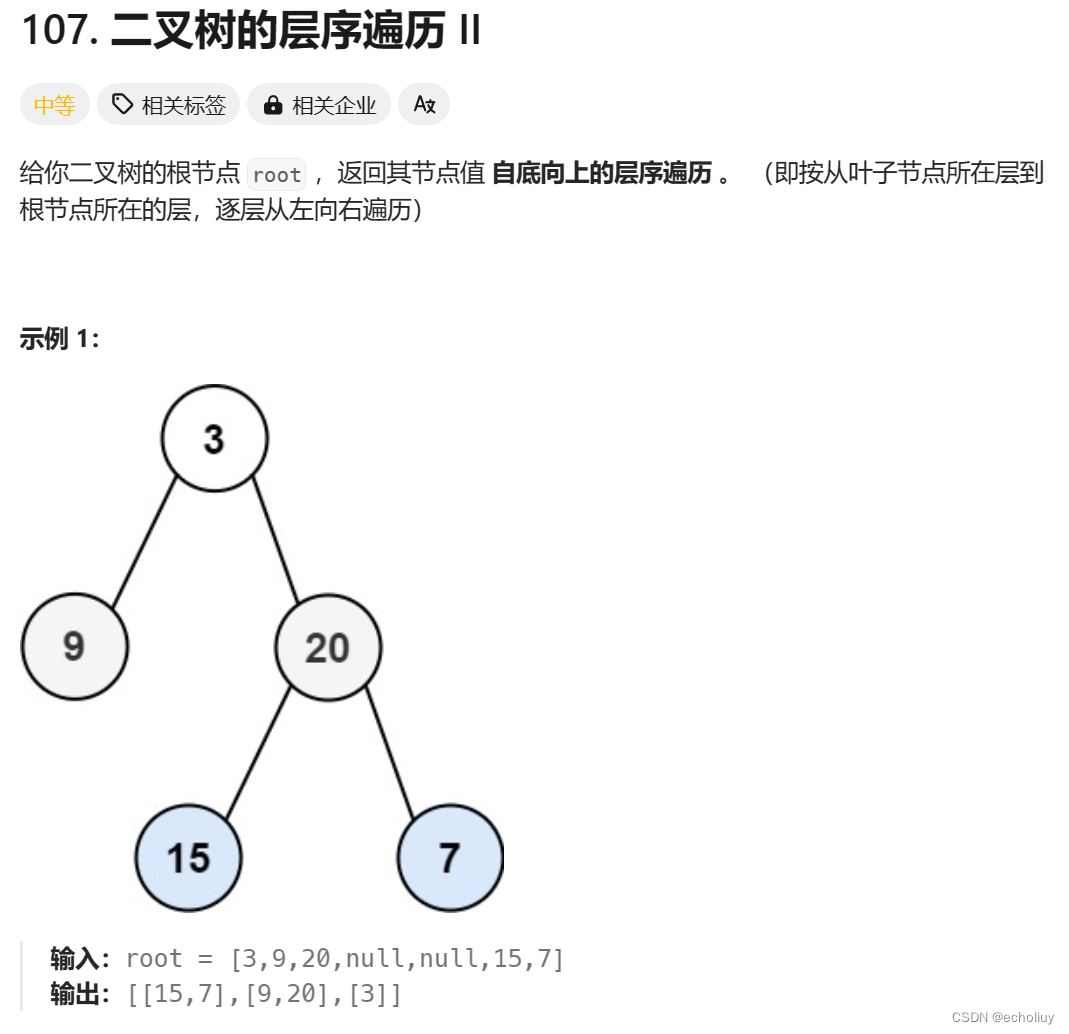
在这里插入图片描述

思路：层序遍历后翻转数组result即可

class Solution {public List<List<Integer>> levelOrderBottom(TreeNode root) {Queue<TreeNode> queue = new LinkedList<>();List<List<Integer>> res = new ArrayList<>();if(root!=null){queue.add(root);}while(!queue.isEmpty()){List<Integer> tmp = new ArrayList<>();int len = queue.size();for(int i=len;i>0;i--){TreeNode node = queue.poll();tmp.add(node.val);if(node.left!=null){queue.add(node.left);}if(node.right!=null){queue.add(node.right);}}res.add(tmp);}// return res;List<List<Integer>> res1 = new ArrayList<>();for(int i=res.size()-1;i>=0;i--){res1.add(res.get(i));}return res1;}
}

199.二叉树的右视图

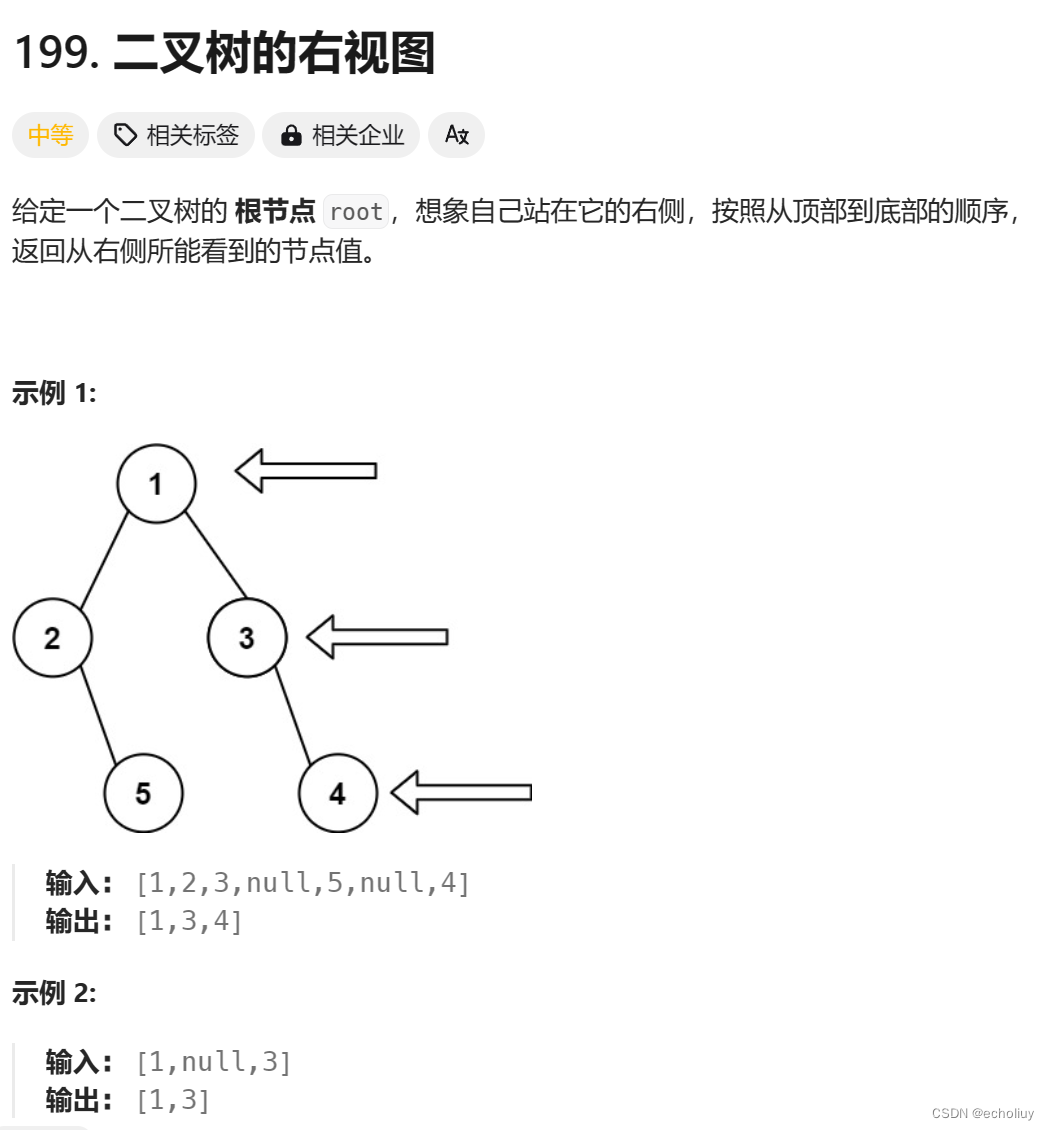
在这里插入图片描述

思路：通过list数组储存每一层末尾值

层序遍历的时候，判断是否遍历到单层的最后面的元素，如果是，就放进result数组中，随后返回result就可以了。

/*** Definition for a binary tree node.* public class TreeNode {*     int val;*     TreeNode left;*     TreeNode right;*     TreeNode() {}*     TreeNode(int val) { this.val = val; }*     TreeNode(int val, TreeNode left, TreeNode right) {*         this.val = val;*         this.left = left;*         this.right = right;*     }* }*/
class Solution {public List<Integer> rightSideView(TreeNode root) {// List<List<Integer>> res = new ArrayList<>();Queue<TreeNode> queue = new LinkedList<>();if(root!=null){queue.add(root);}List<Integer>list=new ArrayList<>();//储存最终结果while(!queue.isEmpty()){int len=queue.size();// List<Integer>temp=new ArrayList<>();for(int i=len;i>0;i--){TreeNode node=queue.poll();if(i==1){//浏览到数组的最后一个，储存list.add(node.val);}if(node.left!=null){queue.add(node.left);}if(node.right!=null){queue.add(node.right);}}}return list;}
}

637.二叉树的层平均值

在这里插入图片描述

思路：通过list数组储存均值

本题就是层序遍历的时候把一层求个总和在取一个均值。

/*** Definition for a binary tree node.* public class TreeNode {*     int val;*     TreeNode left;*     TreeNode right;*     TreeNode() {}*     TreeNode(int val) { this.val = val; }*     TreeNode(int val, TreeNode left, TreeNode right) {*         this.val = val;*         this.left = left;*         this.right = right;*     }* }*/
class Solution {public List<Double> averageOfLevels(TreeNode root) {Queue<TreeNode> queue = new LinkedList<>();List<Double> list = new ArrayList<>();if(root!=null){queue.add(root);}while(!queue.isEmpty()){// List<Integer> list = new ArrayList<>();int len = queue.size();double temp=0;for(int i=len;i>0;i--){TreeNode node = queue.poll();// list.add(node.val);temp+=node.val;if(node.left!=null){queue.add(node.left);}if(node.right!=null){queue.add(node.right);}}list.add(temp/len);}return list;}
}

429.N 叉树的层序遍历

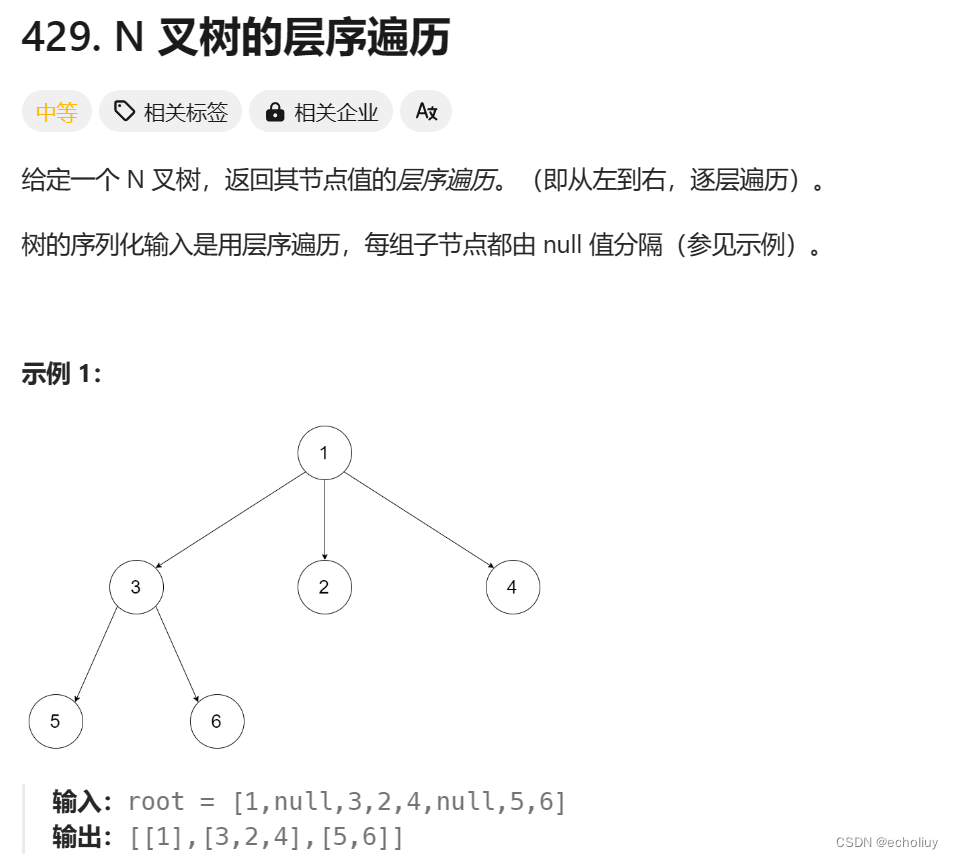
在这里插入图片描述

思路：依次判断并储存孩子节点

注意：

                // List<Node> children = node.children;// if(children==null||children.size()==0){//     continue;// }// for(Node child:children){或者 两种方法for(Node child:node.children){if(child!=null){queue.add(child);}}

/*
// Definition for a Node.
class Node {public int val;public List<Node> children;public Node() {}public Node(int _val) {val = _val;}public Node(int _val, List<Node> _children) {val = _val;children = _children;}
};
*/class Solution {public List<List<Integer>> levelOrder(Node root) {List<List<Integer>> res=new ArrayList<>();Queue<Node> queue =new LinkedList<>();if(root!=null){queue.add(root);}while(!queue.isEmpty()){List<Integer> item = new ArrayList<>();int len=queue.size();for(int i=0;i<len;i++){Node node=queue.poll();item.add(node.val);// List<Node> children = node.children;// if(children==null||children.size()==0){//     continue;// }for(Node child:node.children){if(child!=null){queue.add(child);}}}res.add(item);}return res;}
}

515.在每个树行中找最大值

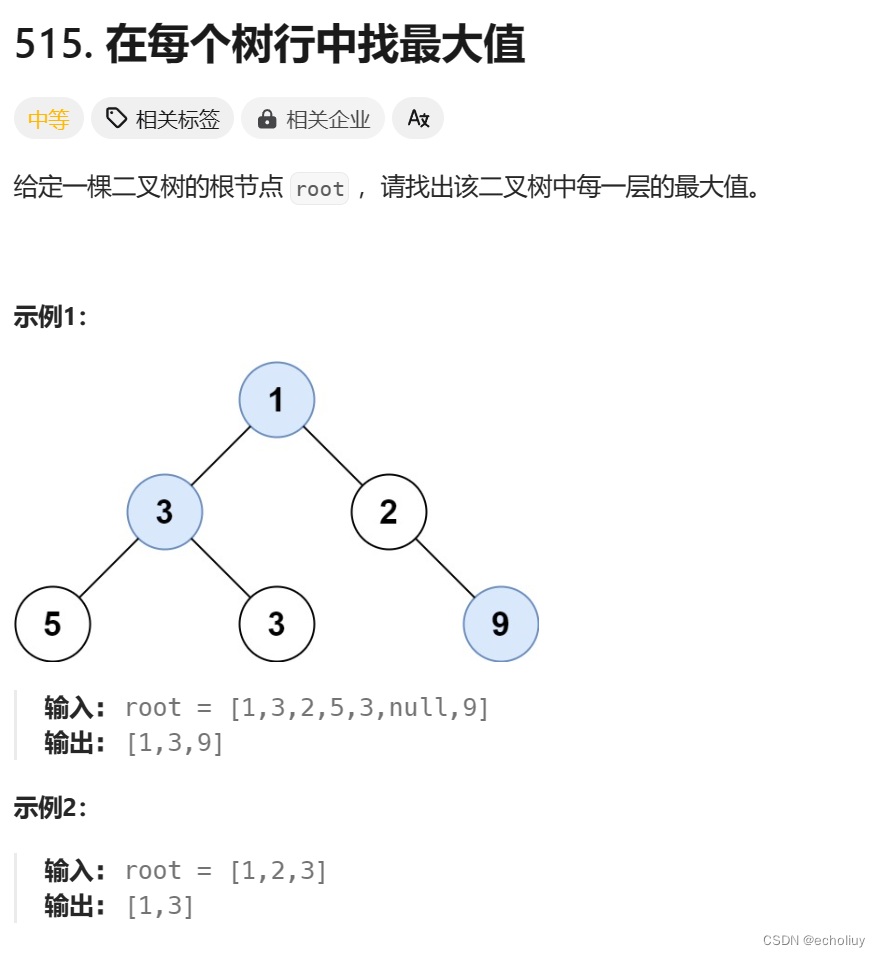
在这里插入图片描述

思路：每一层储存最大值Integer.MIN_VALUE

/*** Definition for a binary tree node.* public class TreeNode {*     int val;*     TreeNode left;*     TreeNode right;*     TreeNode() {}*     TreeNode(int val) { this.val = val; }*     TreeNode(int val, TreeNode left, TreeNode right) {*         this.val = val;*         this.left = left;*         this.right = right;*     }* }*/
class Solution {public List<Integer> largestValues(TreeNode root) {List<Integer> res = new ArrayList<>();Queue<TreeNode> queue = new LinkedList<>();if(root!=null){queue.add(root);}while(!queue.isEmpty()){int len=queue.size();int num=Integer.MIN_VALUE;for(int i=0;i<len;i++){TreeNode node = queue.poll();num=Math.max(num,node.val);if(node.left!=null){queue.add(node.left);}if(node.right!=null){queue.add(node.right);}}res.add(num);}return res;}
}

116.填充每个节点的下一个右侧节点指针

在这里插入图片描述

思路

本题依然是层序遍历，只不过在单层遍历的时候记录一下本层的头部节点，然后在遍历的时候让前一个节点指向本节点就可以了

class Solution {public Node connect(Node root) {Queue<Node>queue = new LinkedList<>();if(root!=null){queue.add(root);}while(!queue.isEmpty()){int len=queue.size();Node pre=null;Node node =null;for(int i=0;i<len;i++){if(i==0){//记录头部节点pre=queue.poll();node=pre;}else{//让头部节点指向本节点node=queue.poll();pre.next=node;pre=pre.next;}if(node.left!=null){queue.add(node.left);}if(node.right!=null){queue.add(node.right);}}pre.next=null;}return root;}
}

117.填充每个节点的下一个右侧节点指针II

在这里插入图片描述

思路：【和前一题相同】

104.二叉树的最大深度

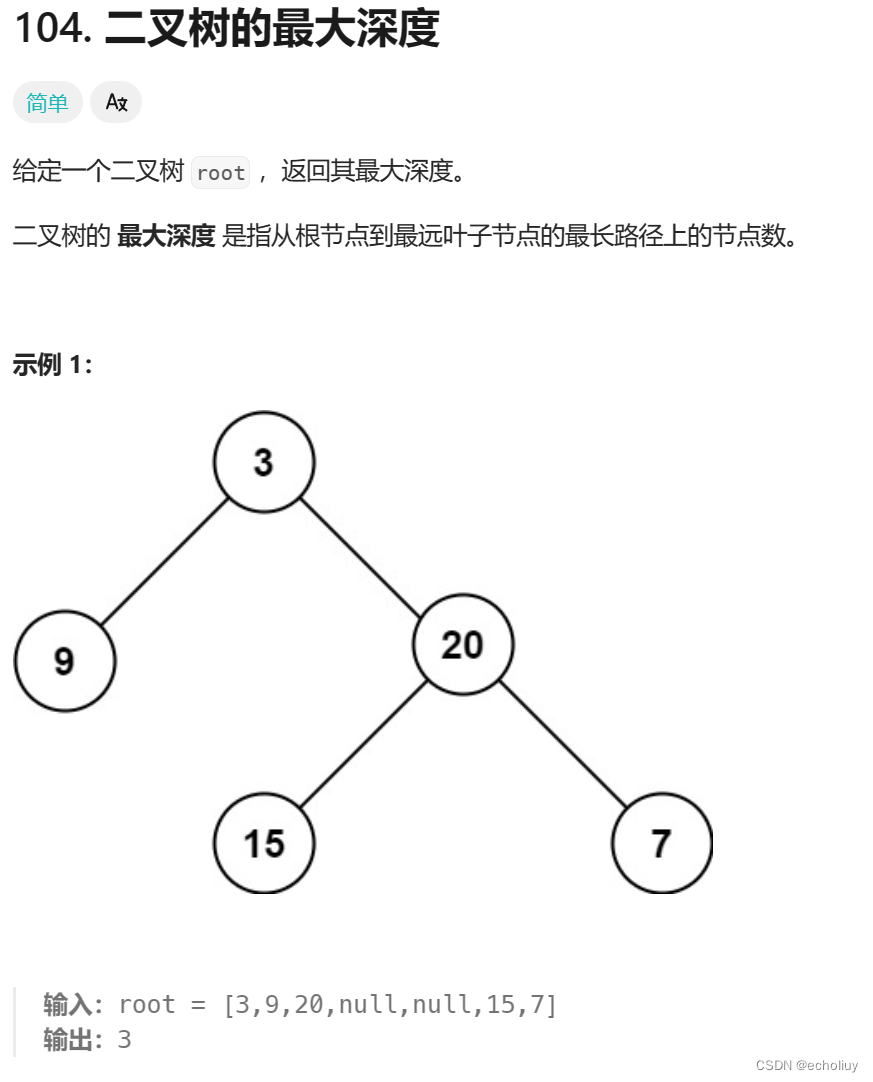
在这里插入图片描述

思路：每层deep+1

class Solution {public int maxDepth(TreeNode root) {Queue<TreeNode>queue = new LinkedList<>();int deep=0;if(root!=null){queue.add(root);}while(!queue.isEmpty()){deep++;int len=queue.size();for(int i=0;i<len;i++){TreeNode node = queue.poll();if(node.left!=null)queue.add(node.left);if(node.right!=null)queue.add(node.right);}}return deep;}
}

111.二叉树的最小深度

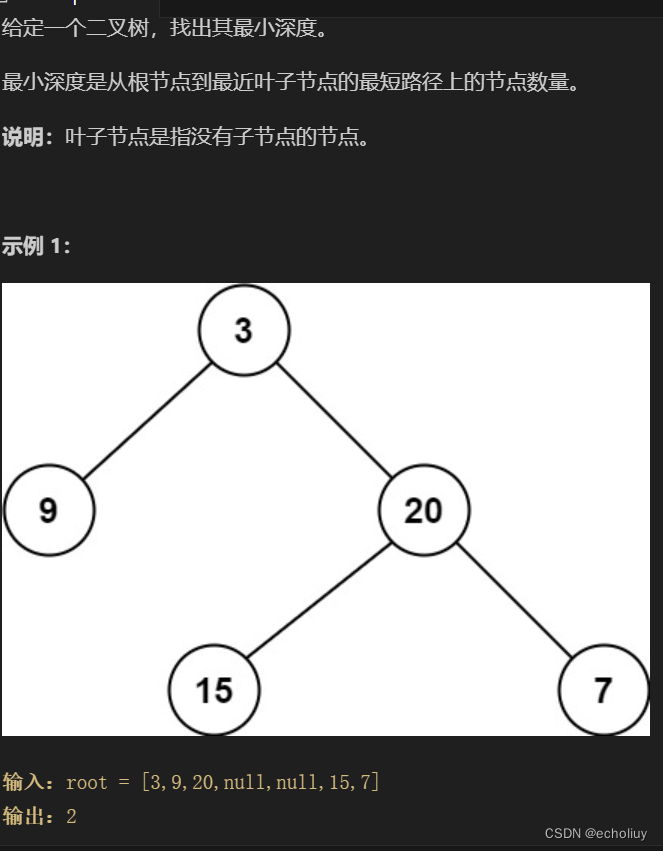
在这里插入图片描述

思路:

相对于 104.二叉树的最大深度，本题还也可以使用层序遍历的方式来解决，思路是一样的。

需要注意的是，只有当左右孩子都为空的时候，才说明遍历的最低点了。如果其中一个孩子为空则不是最低点

class Solution {public int minDepth(TreeNode root) {Queue<TreeNode> queue = new LinkedList<>();int deep=0;if(root!=null){queue.add(root);}while(!queue.isEmpty()){int len=queue.size();deep++;for(int i=0;i<len;i++){TreeNode node =queue.poll();if(node.left!=null)queue.add(node.left);if(node.right!=null)queue.add(node.right);if(node.left==null&&node.right==null){return deep;}}}return deep;}
}

226.翻转二叉树

在这里插入图片描述
注意只要把每一个节点的左右孩子翻转一下，就可以达到整体翻转的效果

这道题目使用前序遍历和后序遍历都可以，唯独中序遍历不方便，因为中序遍历会把某些节点的左右孩子翻转了两次！建议拿纸画一画，就理解了

那么层序遍历可以不可以呢？依然可以的！只要把每一个节点的左右孩子翻转一下的遍历方式都是可以的！

1.递归法DFS

把每一个节点的左右孩子都进行翻转
在这里插入图片描述

代码

后序遍历

class Solution {public TreeNode invertTree(TreeNode root) {if(root==null){return root;}invertTree(root.left);//左invertTree(root.right);//右// 交换				   //中TreeNode temp=null;temp=root.left;root.left=root.right;root.right=temp;return root;}
}

前序遍历

class Solution {public TreeNode invertTree(TreeNode root) {if(root==null){return root;}// 交换 			//中TreeNode temp=null;temp=root.left;root.left=root.right;root.right=temp;return root;invertTree(root.left);  //左invertTree(root.right); //右}
}

2.层序遍历BFS

在添加左右节点前，进行左右节点的交换。

代码：

class Solution {public TreeNode invertTree(TreeNode root) {// if(root==null){//     return root;// }Queue<TreeNode> queue=new LinkedList<>();if(root!=null){queue.add(root);}while(!queue.isEmpty()){int len = queue.size();for(int i=0;i<len;i++){TreeNode node=queue.poll();TreeNode tmp=null;// 交换tmp=node.right;node.right=node.left;node.left = tmp;// 放入左右节点if(node.left!=null){queue.add(node.left);}if(node.right!=null){queue.add(node.right);}}}return root;}
}

101. 对称二叉树

在这里插入图片描述

思路：-使用后序遍历左右中

首先想清楚，判断对称二叉树要比较的是哪两个节点，要比较的可不是左右节点！

对于二叉树是否对称，要比较的是根节点的左子树与右子树是不是相互翻转的，理解这一点就知道了其实我们要比较的是两个树（这两个树是根节点的左右子树），所以在递归遍历的过程中，也是要同时遍历两棵树。

递归法

确定递归函数的参数和返回值

因为我们要比较的是根节点的两个子树是否是相互翻转的，进而判断这个树是不是对称树，所以要比较的是两个树，参数自然也是左子树节点和右子树节点。

返回值自然是bool类型。

代码如下：

bool compare(TreeNode* left, TreeNode* right)

确定终止条件

要比较两个节点数值相不相同，首先要把两个节点为空的情况弄清楚！否则后面比较数值的时候就会操作空指针了。

节点为空的情况有：（注意我们比较的其实不是左孩子和右孩子，所以如下我称之为左节点右节点）

左节点为空，右节点不为空，不对称，return false
左不为空，右为空，不对称 return false
左右都为空，对称，返回true

此时已经排除掉了节点为空的情况，那么剩下的就是左右节点不为空：

左右都不为空，比较节点数值，不相同就return false

if (left == NULL && right != NULL) return false;
else if (left != NULL && right == NULL) return false;
else if (left == NULL && right == NULL) return true;
else if (left->val != right->val) return false; // 注意这里我没有使用else

注意上面最后一种情况，我没有使用else，而是else if，因为我们把以上情况都排除之后，剩下的就是左右节点都不为空，且数值相同的情况。

确定单层递归的逻辑

此时才进入单层递归的逻辑，单层递归的逻辑就是处理左右节点都不为空，且数值相同的情况。

比较二叉树外侧是否对称：传入的是左节点的左孩子，右节点的右孩子。
比较内侧是否对称，传入左节点的右孩子，右节点的左孩子。
如果左右都对称就返回true ，有一侧不对称就返回false 。

bool outside = compare(left->left, right->right);   // 左子树：左、 右子树：右
bool inside = compare(left->right, right->left);    // 左子树：右、 右子树：左
bool isSame = outside && inside;                    // 左子树：中、 右子树：中（逻辑处理）
return isSame;

递归代码

class Solution {public boolean isSymmetric(TreeNode root) {return Compare(root.left,root.right);}public boolean Compare(TreeNode left,TreeNode right){if(left==null&&right==null){return true;}// if(left==null&&right!=null){//     return false;// }    // if(left!=null&&right==null){//     return false;// }// if(left.val!=right.val){//     return false;// }if (left==null||right==null||left.val != right.val) {return false;}// 比较外侧boolean outside = Compare(left.left,right.right);// 比较内侧boolean inside = Compare(left.right,right.left);return outside&&inside;}
}