C++STL之map、set的使用和模拟实现

绪论​：
“我这个人走得很慢，但是我从不后退。——亚伯拉罕·林肯”，本章是接上一章搜索二叉树中红黑树的后续文章，若没有看过强烈建议观看，否则后面模拟实现部分很看懂其代码原理。本章主要讲了map、set是如何使用的，以及map、set如何底层实现相较于前面的章节本章更加考验你的逻辑能力来进行封装，还能帮助对大体框架的有更加好的了解。下一章将进入哈希表的学习，同样也是STL中非常重要且难以学习的章节敬请期待（早关注不迷路！）。话不多说安全带系好，发车啦（建议电脑观看）。

1.set

1.1set的概念

set其底层就是红黑树，所以其很多特性也和红黑树一样也就表明了他就是一个二叉搜索树。set看起来只是存着value，但是set是关联式容器容器内部的元素都是pair<key,value>构成的键值对，所以set的value元素其实就是pair<value,value>它两个元素是相同的且只存了一个值value，但我们在使用的时候并不用管底层只需要插入正常的value值即可，并且在set中所有元素值value都是唯一的不能重复出现，在set中不能修改元素的值，但可以正常增删元素，同二叉搜索树一样他可以通过迭代器遍历出有序对。
set常用来：去重（去掉一个序列的相同值）、排序、查找。

1.2set常使用的方法

  1. set的模板参数：
    在这里插入图片描述

Compare默认缺省为less也就表示其默认是其底层是 x < y，这样如果用迭代器遍历出来就是一个升序。 x < y 返回真进行交换，反之x>y的话返回假（和vector等若干容器中一样）。

  1. set的构造：
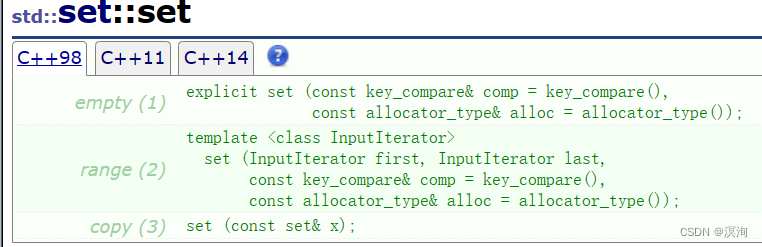    在这里插入图片描述上图123如何使用对应下图123的具体方法：
    在这里插入图片描述源码：
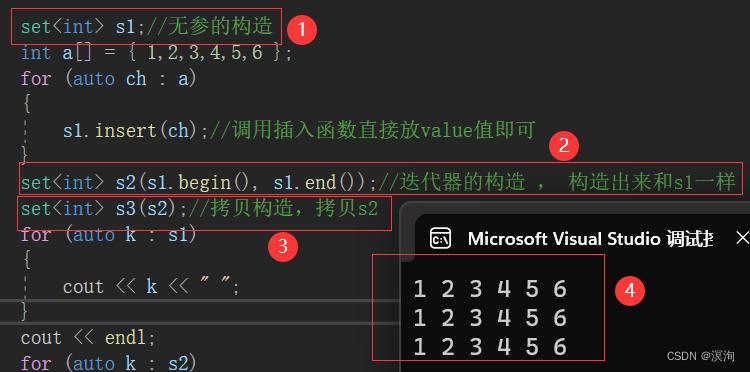
void test1()
{set<int> s1;//无参的构造int a[] = { 1,2,3,4,5,6 };for (auto ch : a){s1.insert(ch);//调用插入函数直接放value值即可}set<int> s2(s1.begin(), s1.end());//迭代器的构造 ， 构造出来和s1一样set<int> s3(s2);//拷贝构造，拷贝s2for (auto k : s1){cout << k << " ";}cout << endl;for (auto k : s2){cout << k << " ";}cout << endl;for (auto k : s3){cout << k << " ";}cout << endl;
}
  1. set的插入：
    在这里插入图片描述
    下图123对应着上图123的使用方法：
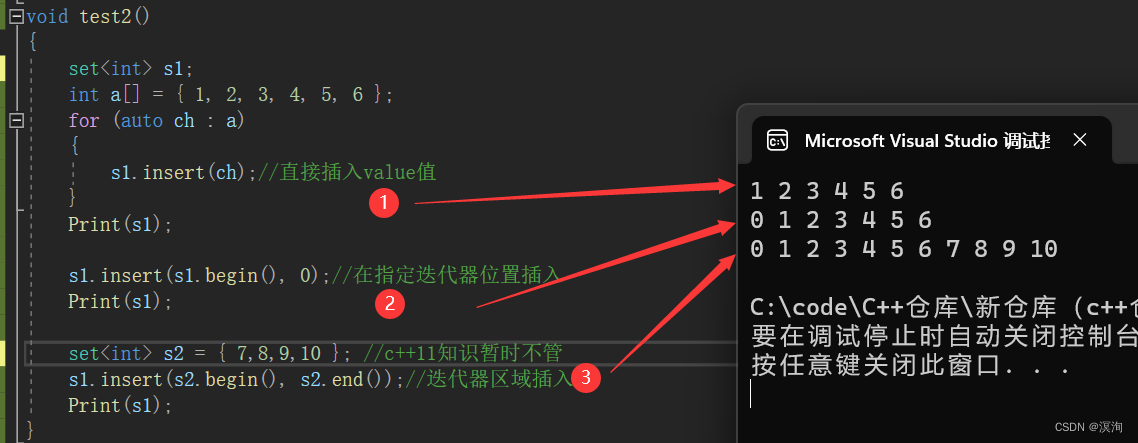
    在这里插入图片描述
    1. 在方法1中的返回值一个pair<iterator,bool>类型，其中iterator指向插入的节点，bool表示true表示不存在该元素插入成功，false表示已经存在该元素则插入失败。
    2. 在方法2中返回的是新插入节点的迭代器。
      源码：
void Print(const set<int>& s1)
{for (auto k : s1){cout << k << " ";}cout << endl;
}
void test2()
{set<int> s1;int a[] = { 1, 2, 3, 4, 5, 6 };for (auto ch : a){s1.insert(ch);//直接插入value值}Print(s1);s1.insert(s1.begin(), 0);//在指定迭代器位置插入Print(s1);set<int> s2 = { 7,8,9,10 }; //c++11知识暂时不管s1.insert(s2.begin(), s2.end());//迭代器区域插入Print(s1);
}
  1. set的删除：在这里插入图片描述
    下图123对应着上图123的使用方法：
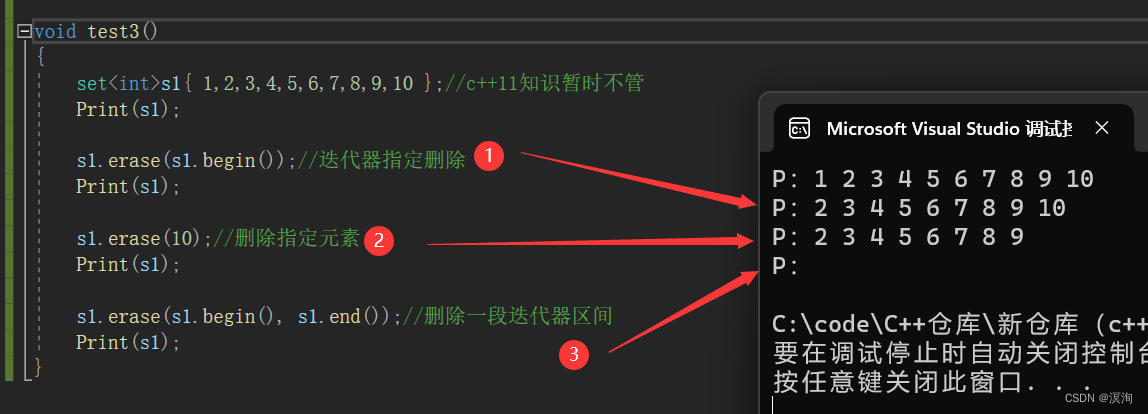
    在这里插入图片描述
    1. 在2方法中返回的是删除元素的个数（size_type 就是 size_t 无符号整形）
      源码：
void Print(const set<int>& s1)
{cout << "P：";for (auto k : s1){cout << k << " ";}cout << endl;
}
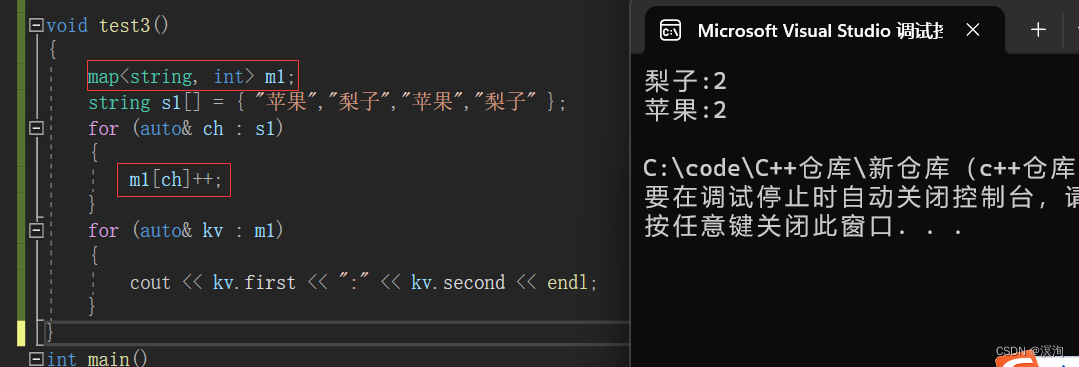
void test3()
{set<int>s1{ 1,2,3,4,5,6,7,8,9,10 };//c++11知识暂时不管Print(s1);s1.erase(s1.begin());//迭代器指定删除Print(s1);s1.erase(10);//删除指定元素Print(s1);s1.erase(s1.begin(), s1.end());//删除一段迭代器区间Print(s1);
}
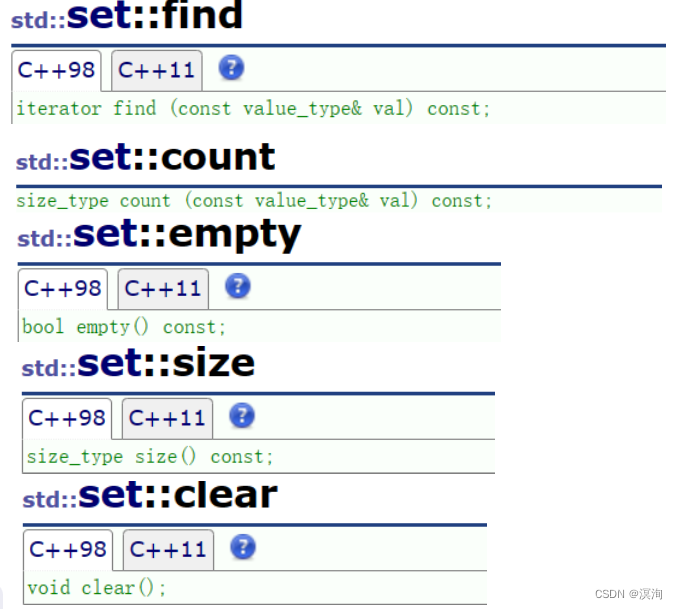
  1. set的查找find、查看个数size、是否为空empty、是否存在count、清空元素clear：
    在这里插入图片描述
    下面通过代码解释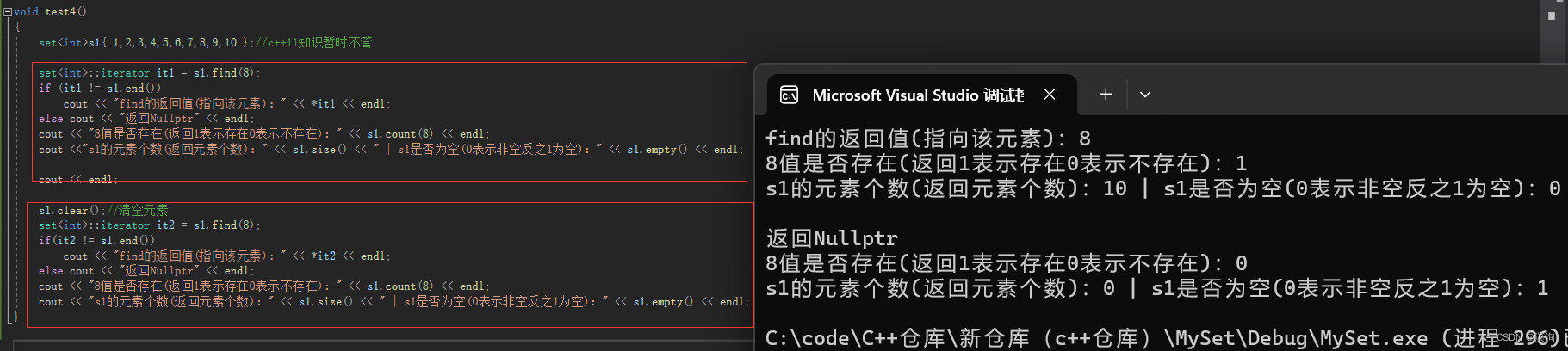：在这里插入图片描述
    1. find，返回迭代器，如找到则返回找到的元素的迭代器，反之返回nullptr的迭代器
    2. size，返回该容器的元素个数
    3. empty，返回0表示非空，返回1表示空
    4. count，返回1表示存在，返回0表示是不存在

源码：

void test4()
{set<int>s1{ 1,2,3,4,5,6,7,8,9,10 };//c++11知识暂时不管set<int>::iterator it1 = s1.find(8);if (it1 != s1.end())cout << "find的返回值(指向该元素)：" << *it1 << endl;else cout << "返回Nullptr" << endl;cout << "8值是否存在(返回1表示存在0表示不存在)：" << s1.count(8) << endl;cout <<"s1的元素个数(返回元素个数)：" << s1.size() << " | s1是否为空(0表示非空反之1为空)：" << s1.empty() << endl;cout << endl;s1.clear();//清空元素set<int>::iterator it2 = s1.find(8);if(it2 != s1.end())cout << "find的返回值(指向该元素)：" << *it2 << endl;else cout << "返回Nullptr" << endl;cout << "8值是否存在(返回1表示存在0表示不存在)：" << s1.count(8) << endl;cout << "s1的元素个数(返回元素个数)：" << s1.size() << " | s1是否为空(0表示非空反之1为空)：" << s1.empty() << endl;
}
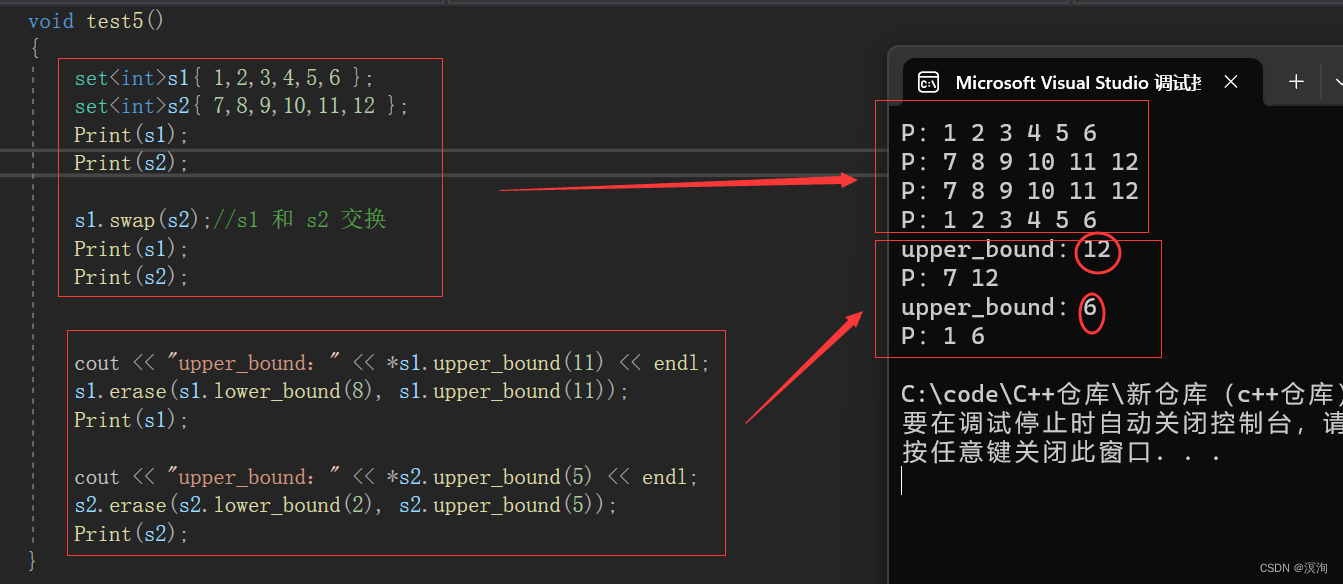
  1. set的交换swap、指定区间的lower_bound、upper_bound：
    在这里插入图片描述

lower_bound和upper_bound是通过指定数值来确定迭代器区间，常用来指定删除数据的区间，如：1 2 3 4 5 6 ， lower_bound(2)，upper_bound(5)，这样取出来的区间lower_bound指向的就是2（取>=value的值），而upper_bound指向的是6（取>value的值），但因为迭代器区间是左闭右开的所以即使删除也不会删到6！
在这里插入图片描述

源码：

void Print(const set<int>& s1)
{cout << "P：";for (auto k : s1){cout << k << " ";}c
void test5()
{set<int>s1{ 1,2,3,4,5,6 };set<int>s2{ 7,8,9,10,11,12 };Print(s1);Print(s2);s1.swap(s2);//s1 和 s2 交换Print(s1);Print(s2);cout << "upper_bound：" << *s1.upper_bound(11) << endl;s1.erase(s1.lower_bound(8), s1.upper_bound(11));Print(s1);cout << "upper_bound：" << *s2.upper_bound(5) << endl;s2.erase(s2.lower_bound(2), s2.upper_bound(5));Print(s2);
}

1.3 multiset的概念和使用

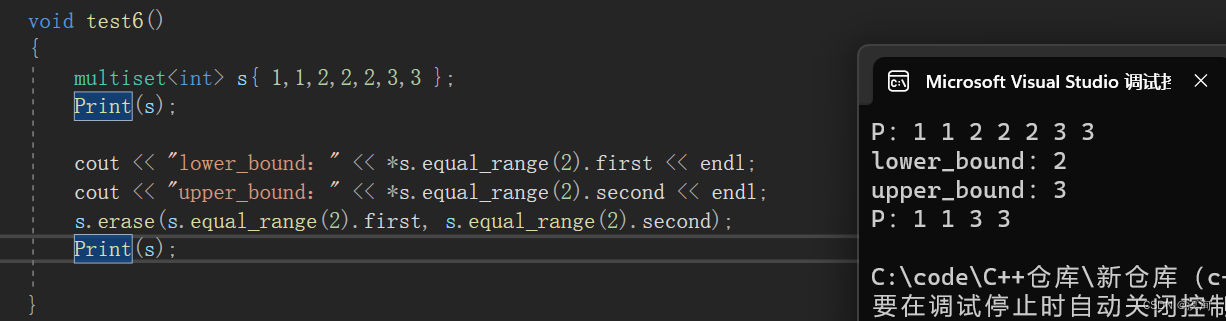
其原理和set几乎一致，只是multiset能存多个相同的值了
注意点：是find查找时是返回第一个遇到的value，count将返回该值存在的个数
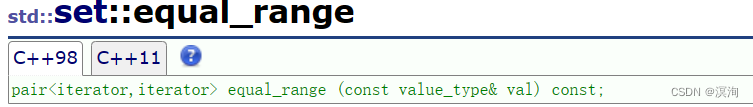
其中还要交代一个函数(set中也有不过不够实用)
在这里插入图片描述
他返回的是pair<iterator,iterator>，这两个迭代器分别表示的就是value值的lower_bound和upper_bound，这样就能一次性删除所有相同的元素！在这里插入图片描述

void Print(const multiset<int>& s1)
{cout << "P：";for (auto k : s1){cout << k << " ";}cout << endl;
}
void test6()
{multiset<int> s{ 1,1,2,2,2,3,3 };Print(s);cout << "lower_bound：" << *s.equal_range(2).first << endl;cout << "upper_bound：" << *s.equal_range(2).second << endl;s.erase(s.equal_range(2).first, s.equal_range(2).second);Print(s);
}

2.map

2.1map的概念

map底层也是红黑树，同理也就表明了他就是一个二叉搜索树。map不同于set他的K,V两个值都是有用的，map也是关联式容器，
1. 容器内部的元素都是pair<K,V>构成的一个个键值对
2. 如果是比较大小的话通常是用key值来进行
3. 在map中所有元素的值都是唯一的（指的是key值不能重复）
4. 在map中不能修改元素的值（即是不能修改K值的，只能修改value值）

2.2map常使用的方法

  1. map的模板参数：
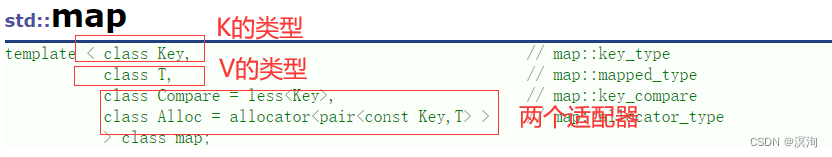
    在这里插入图片描述

同样Compare默认缺省为less

  1. map的构造：
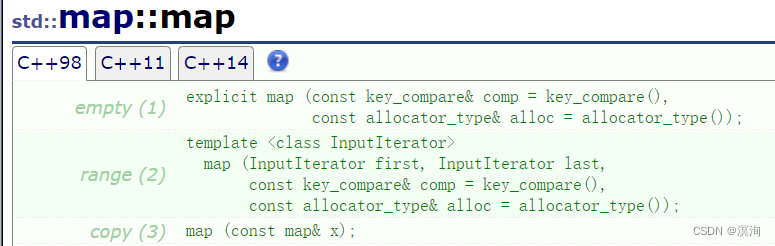
    在这里插入图片描述
    对照着set几乎一样，直接通过代码展示了
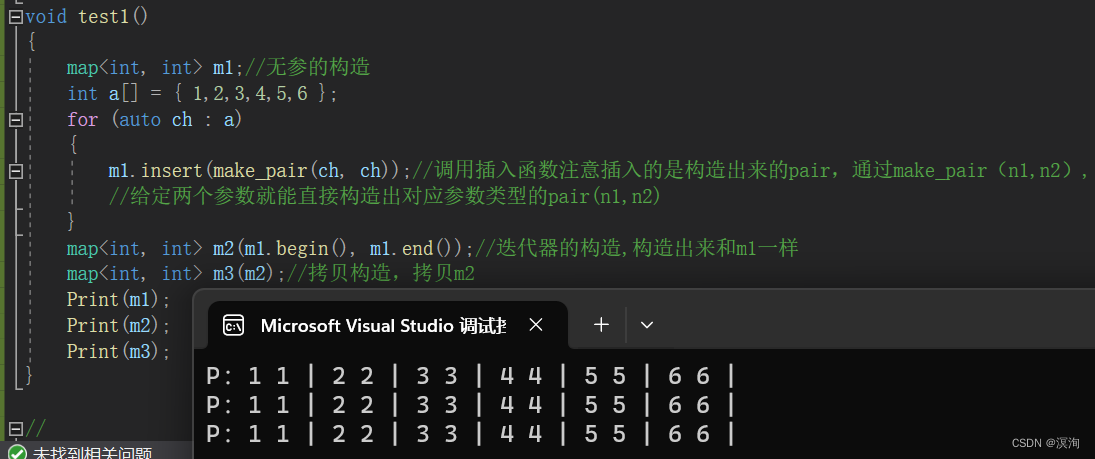
    在这里插入图片描述
    注意点：
    1. 范围for打印时给kv的是迭代器，迭代器的类型是pair<K,V>所以kv.first指向Key，kv.second指向value
    2. 插入的数据必须是键值对，用make_pair()函数直接构造

源码：

void Print(const map<int,int>& m1)
{cout << "P：";for (auto kv : m1){cout << kv.first << " " << kv.second << " | ";}cout << endl;
}
void test1()
{map<int, int> m1;//无参的构造int a[] = { 1,2,3,4,5,6 };for (auto ch : a){m1.insert(make_pair(ch, ch));//调用插入函数注意插入的是构造出来的pair，通过make_pair（n1,n2）,//给定两个参数就能直接构造出对应参数类型的pair(n1,n2)}map<int, int> m2(m1.begin(), m1.end());//迭代器的构造,构造出来和m1一样map<int, int> m3(m2);//拷贝构造，拷贝m2Print(m1);Print(m2);Print(m3);
}
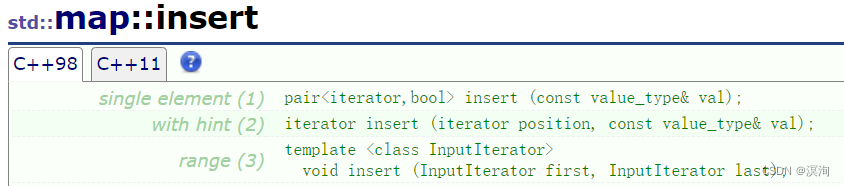
  1. set的插入：在这里插入图片描述在这里插入图片描述
    其中插入函数参数的value_type的实际类型是pair<const K,V>
    注意点：
    1. 用make_pair(key,value)构造出K,V类型的pair<K,V>当参数传递进去，或者写成用pair的构造pair<K,V>(key,value)（具体如下）在这里插入图片描述
      在这里插入图片描述
    2. 返回pair<iterator,bool>。
    3. 若返回的iterator，需要注意的是其类型是pair<K,V>型
map<int, int> m1;
m1.insert(make_pair(1,1));//构造出pair(1,1) 或m1.insert(pair<int,int>(1,1));
  1. map的删除：
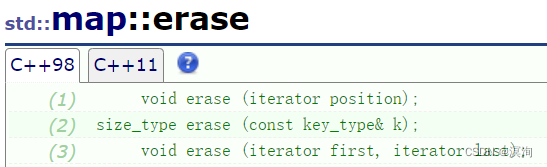
    在这里插入图片描述
    同理使用迭代器部分是一样的，此处删除时就不用在使用pair了，直接通过确定key即可删除。
    在这里插入图片描述
void test2()
{map<int, int> m1;int a[] = { 1,2,3};for (auto ch : a){m1.insert(make_pair(ch, ch));}Print(m1);m1.erase(1);Print(m1);
}
  1. map的查找find、查看个数size、是否为空empty、是否存在count、清空元素clear、交换swap：

此处find、count的参数同样用key即可使用、其余函数和set完全一样。
在这里插入图片描述

  1. map的重载operator[]（极其重要）

通过代码和底层来解释：在这里插入图片描述
其中我们operator[]的底层是：
(*((this->insert(make_pair(k,mapped_type()))).first)).second
其中能看到他是调用了插入函数，所以[]能实现插入功能
而我们map的插入返回的类型是：pair<iterator,bool>
所以就能简化为：(*pair<iterator,bool>.first).second//此处.的优先级高于*所以是先访问，得到iterator后，再(*iterator).second得到其迭代器的value值，也就是插入时的第二个参数
所以分析上面代码：m1[ch]++;第一次进去的时候插入成功并且返回来他的second++，这样就能不断的插入，即使后面是插入失败也能返回second进行++，所以就能形成计数器。（其中注意点我们虽然没有初始化int，但其实其内部会默认为0开始，也就是上面的mapped_type()他会自动构造出该类型的初始值，int为0，string为""）

2.3 multimap的概念和使用

与multiset一样，都是可以出现重复的值，只是其中没有了map的operator[ ]，就不过诉了。

3.map、set的底层实现

3.1红黑树的修改

为了给set、map做底层，我们需要完善一下红黑树在里面主要是

  1. 模板的改变：将原本第二个参数V改成T，T代表的是K，V组成成的键值对pair<K,V>
  2. 添加迭代器以及begin、end函数，让map、set也能用迭代器
  3. 修改插入的返回值：将原本的iterator改成pair<iterator,bool>，（这是STL源码内的设计，也是为了map的[]做准备）

修改后的源码：

#pragma once
#include<iostream>
using namespace std;
enum Color
{BLACK,RED
};template<class T>
struct RBTreeNode {RBTreeNode<T>* _left = nullptr;RBTreeNode<T>* _right = nullptr;RBTreeNode<T>* _parent = nullptr;T _data;Color _col = RED;//默认生成的节点颜色是红色RBTreeNode(const T& data):_data(data){}
};//迭代器
template<class T, class Ptr, class Ref>
struct _TreeIterator
{typedef _TreeIterator<T, Ptr, Ref> Self;typedef RBTreeNode<T> Node;Node* _node;//迭代器的成员变量_TreeIterator(Node* node):_node(node){}Self& operator++(){Node* cur = _node;if (cur->_right)//若右边不为空，则找到其左边的右边节点{cur = cur->_right;while (cur->_left){cur = cur->_left;}_node = cur;}else{Node* parent = cur->_parent;while (parent && parent->_left != cur){cur = parent;parent = parent->_parent;}_node = parent;}return *this;}Ref operator*(){return _node->_data;}Ptr operator->(){return &_node->_data;}bool operator==(const Self & it){return _node == it._node;}bool operator!=(const Self & it){return _node != it._node;}};template<class K, class T, class Compare>
class RBTree
{typedef RBTreeNode<T> Node;Compare kot;
public:typedef _TreeIterator<T,T*,T&> iterator;typedef _TreeIterator<T,const T*,const T&> const_iterator;//typedef typename RBTree<K, pair<K, V>, Mapofkey>::iterator iterator;// 在红黑树中插入值为data的节点，插入成功返回true，否则返回false// 注意：为了简单起见，本次实现红黑树不存储重复性元素iterator begin(){Node* cur = _root;while (cur && cur->_left){cur = cur->_left;}return iterator(cur);}iterator end(){return iterator(nullptr);//end指向最后数据的后面故为空}const_iterator begin() const{Node* cur = _root;while (cur && cur->_left){cur = cur->_left;}return const_iterator(cur);}const_iterator end() const{return const_iterator(nullptr);//end指向最后数据的后面故为空}//此处用Node* 的原因set处的iterator为了防止被修改所以set内的iterator本质还是const_iterator，//所以这里用了 Node* 来代替iterator 的返回pair<Node*, bool> Insert(const T& data){//此处和AVL平衡二叉树的性质一样找到所要插入节点的位置 大的在右 、 小的在左Node* parent = nullptr;Node* cur = _root;if (cur == nullptr){_root = new Node(data);_root->_col = BLACK;return make_pair(_root, true);}//找到插入的位置！while (cur)//当为null时表示此处就是要插入的位置！{if (kot(cur->_data) > kot(data)){parent = cur;cur = cur->_left;}else if (kot(cur->_data) < kot(data)){parent = cur;cur = cur->_right;}else{return make_pair(nullptr, false);}}//找到位置后，插入cur = new Node(data);//建立新节点Node* ret = cur;//建立链接if (kot(parent->_data) > kot(data)){parent->_left = cur;}else{parent->_right = cur;}cur->_parent = parent;//插入时要判断插入后是否会导致不平衡！对于红黑树来说主要问题有//1. 不能出现连续的红节点//2. 最长路径不超过最短路径的两倍//判断是否需要变色/旋转// //1.当父亲节点为黑色时，当新增了一个红色节点时就结束插入了// //2.当父为红时：//	情况一(仅变色即可)：当parent为红 grandfather为黑 uncle存在且为黑 插入一个新节点while (parent && parent->_col == RED){Node* g = parent->_parent;//grandfatherif (g->_left == parent){Node* u = g->_right;//uncleif (u && u->_col == RED)//u存在且为红{//变色即可u->_col = parent->_col = BLACK;g->_col = RED;//向上调整cur = g;parent = g->_parent;//当g 的 父亲为黑时或者为null时停止调整}else //u不存在或者为黑{if (cur == parent->_left)//此处u不存在和当插入节点在左边时的情况一样直接右旋加变色即可{//旋转加变色RotateR(g);parent->_col = BLACK;g->_col = RED;}else{//旋转加变色RotateL(parent);RotateR(g);cur->_col = BLACK;g->_col = RED;}}}else{Node* u = g->_left;//uncleif (u && u->_col == RED)//u存在且为红{//变色即可u->_col = parent->_col = BLACK;g->_col = RED;//向上调整cur = g;parent = g->_parent;//当g 的 父亲为黑时或者为null时停止调整}else //u不存在或者为黑{if (cur == parent->_right)//此处u不存在和当插入节点在左边时的情况一样直接右旋加变色即可{RotateL(g);parent->_col = BLACK;g->_col = RED;}else{RotateR(parent);RotateL(g);cur->_col = BLACK;g->_col = RED;}}}}_root->_col = BLACK;return make_pair(ret, true);}void Inorder(){_Inorder(_root);cout << endl;}// 获取红黑树最左侧节点Node* LeftMost(){Node* cur = _root;while (cur){if (cur->_left == nullptr){return cur;}cur = cur->_left;}return nullptr;}// 获取红黑树最右侧节点Node* RightMost(){Node* cur = _root;while (cur){if (cur->_right == nullptr){return cur;}cur = cur->_right;}return nullptr;}//  检测红黑树是否为有效的红黑树，注意：其内部主要依靠_IsValidRBTRee函数检测//	1.每条路径中的黑色节点个数是否一样//	2.最长路径不超过最短路径的两倍//	3.不能出现连续的红色节点//	4.根节点为黑色bool IsValidRBTRee(){if (_root == nullptr) return true;if (_root->_col == RED) return false;Node* cur = _root;int blackCount = 0;while (cur){if (cur->_col == BLACK){blackCount++;}cur = cur->_left;}return _IsValidRBTRee(_root, blackCount, 0);}int Height(){if (_root == nullptr) return 0;return _Height(_root);}int Size(){if (_root == nullptr) return 0;return _Size(_root);}//检测红黑树中是否存在值为data的节点，存在返回该节点的地址，否则返回nullptrNode* Find(const K& val){Node* cur = _root;while (cur){if (kot(cur->_data) == val){return cur;}else if (kot(cur->_data) > val){cur = cur->_left;}else {cur = cur->_right;}}return nullptr;}
private:int _Size(Node* root){if (root == nullptr)return 0;return _Size(root->_left) +_Size(root->_right) + 1;}int _Height(Node* root){if (root == nullptr)return 0;int lefthight = _Height(root->_left);int righthight = _Height(root->_right);return lefthight > righthight ? lefthight + 1 : righthight + 1;}void _Inorder(Node* root){if (root == nullptr)return;_Inorder(root->_left);cout << root->_data.first << " ";_Inorder(root->_right);}bool _IsValidRBTRee(Node* root, size_t blackCount, size_t pathBlack){if (root == nullptr){if (blackCount != pathBlack)//当为null时表示该路径已经结束，那么判断改路径的黑色节点（pathblack） 和其他路径的黑色节点（blacCount）是否相同{return false;}return true;}if (root->_col == RED){if (root->_left && root->_right && (root->_left->_col == RED || root->_right->_col == RED)){cout << "有连续的红色节点" << endl;return false;}}if (root->_col == BLACK){pathBlack++;}return _IsValidRBTRee(root->_left, blackCount, pathBlack) &&_IsValidRBTRee(root->_right, blackCount, pathBlack);}//	// 为了操作树简单起见：获取根节点//Node*& GetRoot();void RotateR(Node* parent){Node* SubL = parent->_left;//此处就为 curNode* SubLR = SubL->_right;//parent的左换成cur的右parent->_left = SubLR;//把cur的右孩子换成parentSubL->_right = parent;//注意还要修改其父指针Node* Ppnode = parent->_parent;parent->_parent = SubL;if (SubLR)//cur的右边可能为空SubLR->_parent = parent;if (_root == parent)//如果parent为根节点，则需要把subR改变成根节点并且其父亲为nullptr{_root = SubL;SubL->_parent = nullptr;}else{//同时还要考虑父亲 是祖先的左或右if (Ppnode->_left == parent){Ppnode->_left = SubL;}else{Ppnode->_right = SubL;}SubL->_parent = Ppnode;}}// 左单旋// 同理void RotateL(Node* parent){Node* SubR = parent->_right;//此处就为 curNode* SubRL = SubR->_left;//parent的右换成cur的左parent->_right = SubRL;//把cur的左孩子换成parentSubR->_left = parent;Node* Ppnode = parent->_parent;//注意 还要修改其父指针parent->_parent = SubR;if (SubRL)//右边可能为空SubRL->_parent = parent;if (_root == parent)//如果parent为根节点，则需要把subR改变成根节点并且其父亲为nullptr{_root = SubR;SubR->_parent = nullptr;}else{//同时还要考虑父亲 是祖先的左或右if (Ppnode->_left == parent){Ppnode->_left = SubR;}else{Ppnode->_right = SubR;}SubR->_parent = Ppnode;}}private:Node* _root = nullptr;
};

为了让set、map能实现迭代器所以还要写好迭代器常用的重载operator*、operator++、operator!=、…

下面是实现过程也就是再将以及写好的红黑树进行再一次的封装，实现set、map所需要的功能。

3.2set的实现

#pragma once
#include"RBTree.h"template<class K>
class MySet
{
public:struct Setofkey{//自己所写的适配器用来从pair中调出key值const K& operator()(const K& key){return key;}};//通过红黑树的迭代器来重定义生成自己的
//typename的意思是声明他是类型名typedef typename RBTree<K, K, Setofkey>::const_iterator iterator;typedef typename RBTree<K, K, Setofkey>::const_iterator const_iterator;pair<iterator, bool> Insert(const K& key){return _rb.Insert(key);// pair<Node*, bool> 给 pair<iterator, bool>// pair的构造函数： template<class U, class V> pair (const pair<U,V>& pr);// 这样就能通过一个pair去构造另外一个pair// 所以返回来的pair<Node*,bool> 就会对应给到要返回的pair<iterator,bool>的 iterator 和 bool 来进行构造// 这样就能iterator避免内外的不一样，外部的是iterator其实是const_iterator}pair<iterator, bool> Insert(const K& key)const{return _rb.Insert(key);//pair<iterator, bool>  }//普通对象、const对象都能调用iterator begin() const{return _rb.begin();}iterator end() const{return _rb.end();}private:RBTree<K,K,Setofkey> _rb;
};

3.3map的实现

#pragma once
#include"RBTree.h"template<class K,class V>
class MyMap
{
public:
//自己所写的适配器用来从pair中调出key值struct Mapofkey{const K& operator()(const pair<K, V>& kv){return kv.first;}};typedef typename RBTree<K, pair<const K, V>,Mapofkey>::iterator iterator;typedef typename RBTree<K, pair<const K, V>, Mapofkey>::const_iterator const_iterator;pair<iterator, bool> Insert(const pair<K,V>& kv){return _rb.Insert(kv);}iterator begin(){return _rb.begin();}iterator end(){return _rb.end();}const_iterator begin() const{return _rb.begin();}const_iterator end() const{return _rb.end();}V& operator[](const K& key){pair<iterator, bool> ret = Insert(make_pair(key,V()));return ret.first->second;}private:RBTree<K, pair<const K,V>,Mapofkey> _rb;
};

本章完。预知后事如何，暂听下回分解。

如果有任何问题欢迎讨论哈！

如果觉得这篇文章对你有所帮助的话点点赞吧！

持续更新大量C++细致内容，早关注不迷路。

​

本文来自互联网用户投稿，该文观点仅代表作者本人，不代表本站立场。本站仅提供信息存储空间服务，不拥有所有权，不承担相关法律责任。如若转载，请注明出处：http://www.rhkb.cn/news/247578.html

如若内容造成侵权/违法违规/事实不符，请联系长河编程网进行投诉反馈email:809451989@qq.com，一经查实，立即删除！

相关文章

Spring - 基本用法参考

Spring - 基本用法参考

Spring 官方文档 Spring容器启动流程&#xff08;源码解读&#xff09; BeanFactoryPostProcessor vs BeanPostProcessor vs BeanDefinitionRegistryPostProcessor&#xff1a; From java doc&#xff1a; BeanFactoryPostProcessor may interact with and modify bean defin…
阅读更多...
（九）springboot实战——springboot3下的webflux项目参数验证及其全局参数验证异常处理

（九）springboot实战——springboot3下的webflux项目参数验证及其全局参数验证异常处理

前言 在上一节内容中&#xff0c;我们介绍了如何在webflux项目中自定义实现一个全局的异常处理器ErrorWebExceptionHandler&#xff0c;正常情况下其可以处理我们系统的运行时异常&#xff0c;但是无法处理参数验证的异常WebExchangeBindException&#xff0c;所以这里提供另外…
阅读更多...
Nodejs前端学习Day3_准备工作

Nodejs前端学习Day3_准备工作

妈的&#xff0c;这几天真tm冷&#xff0c;前天上午还下了一整天的雪&#xff0c;大雪 文章目录 前言一、Node.js简介1.1何为1.2有什么 二、Node.js可以做什么三、学习路线四、下载nodejs4.1小坑记录4.2LTS和Current版本的不同 五、什么是终端六、在nodejs中执行js代码七、powe…
阅读更多...
Linux使用匿名管道实现进程池得以高效通信

Linux使用匿名管道实现进程池得以高效通信

&#x1f3ac;慕斯主页&#xff1a;修仙—别有洞天 ♈️今日夜电波&#xff1a;Nonsense—Sabrina Carpenter 0:50━━━━━━️&#x1f49f;──────── 2:43 &#x1f504; ◀️ ⏸ ▶️ …
阅读更多...
ubuntu 22.04 安装mysql-8.0.34

ubuntu 22.04 安装mysql-8.0.34

ubuntu 22.04 安装mysql-8.0.34 1、基础安装配置 更新软件包&#xff1a; sudo apt update查看可用软件包&#xff1a; sudo apt search mysql-server安装最新版本&#xff1a; sudo apt install -y mysql-server或者&#xff0c;安装指定版本&#xff1a; sudo apt inst…
阅读更多...
如何实现无公网ip远程SSH连接家中本地的树莓派

如何实现无公网ip远程SSH连接家中本地的树莓派

文章目录 如何通过 SSH 连接到树莓派步骤1. 在 Raspberry Pi 上启用 SSH步骤2. 查找树莓派的 IP 地址步骤3. SSH 到你的树莓派步骤 4. 在任何地点访问家中的树莓派4.1 安装 Cpolar4.2 cpolar进行token认证4.3 配置cpolar服务开机自启动4.4 查看映射到公网的隧道地址4.5 ssh公网…
阅读更多...
qemu + vscode图形化调试linux kernel

qemu + vscode图形化调试linux kernel

一、背景 使用命令行连接gdb 在调试时&#xff0c;虽然可以通过tui enable 显示源码&#xff0c;但还是存在设置断点麻烦&#xff08;需要对着源码设置&#xff09;&#xff0c;terminal显示代码不方便&#xff0c;不利于我们学习&#xff1b;另外在gdb 下p命令显示结构体内容…
阅读更多...
【command】使用nr简化npm run命令

【command】使用nr简化npm run命令

参考文章 添加 alias nrnpm run通过alias启动命令可以帮助我们节省运行项目输入命令的时间 $ cd ~ $ vim .bash_profile $ source ~/.bashrc
阅读更多...
ChatGPT与文心一言：智能回复与语言准确性的较量

ChatGPT与文心一言：智能回复与语言准确性的较量

在当今数字化时代&#xff0c;随着人们对智能化技术的需求不断增长&#xff0c;智能回复工具也成为了日常生活中不可或缺的一部分。ChatGPT和文心一言作为两个备受瞩目的智能回复工具&#xff0c;在智能回复、语言准确性以及知识库丰富度等方面各有卓越之处。 本文将对这两者进…
阅读更多...
14.5 Flash查询和添加数据库数据

14.5 Flash查询和添加数据库数据

14.5 Flash查询和添加数据库数据 在Flash与数据库通讯的实际应用中&#xff0c;如何实现用户的登录与注册是经常遇到的一个问题。登录实际上就是ASP根据Flash提供的数据查询数据库的过程&#xff0c;而注册则是ASP将Flash提供的数据写入数据库的过程。 1.启动Access2003&…
阅读更多...
LeetCode 热题 100 | 子串

LeetCode 热题 100 | 子串

目录 1 560. 和为 K 的子数组 2 239. 滑动窗口最大值 3 76. 最小覆盖子串 菜鸟做题第二周&#xff0c;语言是 C 1 560. 和为 K 的子数组 题眼&#xff1a;“子数组是数组中元素的连续非空序列。” 解决本问题的关键就在于如何翻译问题。子数组 s 的和可以看作数组 i 的…
阅读更多...
C++ 类与对象（上）

C++ 类与对象（上）

目录 本节目标 1.面向过程和面向对象初步认识 2.类的引入 3.类的定义 4.类的访问限定符及封装 4.1 访问限定符 4.2 封装 5. 类的作用域 6. 类的实例化 7.类对象模型 7.1 如何计算类对象的大小 7.2 类对象的存储方式猜测 7.3 结构体内存对齐规则 8.this指针 8.1 thi…
阅读更多...
Swift 周报 第四十六期

Swift 周报 第四十六期

文章目录 前言卖不动了&#xff1f;iPhone 15 系列跌破 5000 元大关StoreKit 和审核指南更新将你的 App 提交到 Apple Vision Pro 的 App Store 提案通过的提案 Swift论坛推荐博文话题讨论关于我们 前言 本期是 Swift 编辑组整理周报的第四十六期&#xff0c;每个模块已初步成…
阅读更多...
用C语言实现贪吃蛇游戏！！！

用C语言实现贪吃蛇游戏！！！

前言 大家好呀&#xff0c;我是Humble&#xff0c;不知不觉在CSND分享自己学过的C语言知识已经有三个多月了&#xff0c;从开始的C语言常见语法概念说到C语言的数据结构今天用C语言实现贪吃蛇已经有30余篇博客的内容&#xff0c;也希望这些内容可以帮助到各位正在阅读的小伙伴…
阅读更多...
Spark3内核源码与优化

Spark3内核源码与优化

文章目录 一、Spark内核原理1、Spark 内核概述1.1 简介1.2 Spark 核心组件1.3 Spark 通用运行流程概述 2、Spark 部署模式2.1 YARN Cluster 模式(重点)2.2 YARN Client 模式2.3 Standalone Cluster 模式2.4 Standalone Client 模式 3、Spark 通讯架构3.1 Spark 通信架构概述3.2…
阅读更多...
Springboot响应数据详解

Springboot响应数据详解

功能接口 Controller下每一个暴露在外的方法都是一个功能接口 功能接口的请求路径是RequestMapping定义的路径&#xff0c;浏览器需要请求该功能则需要发出该路径下的请求。 RestController RestControllerControllerResponseBody(响应数据的注解) ResponseBody 类型&#…
阅读更多...
视频号下载提取器：如何轻松获取视频号的视频

视频号下载提取器：如何轻松获取视频号的视频

在数字化的世界中&#xff0c;我们每天重复刷着形形色色的短视频&#xff0c;你们知道他们每天接收到的媒体内容就是经过不断的处理和编辑呈现在我们观看的产物。 其中&#xff0c;视频内容由于其生动形象的表现形式&#xff0c;已经成为人们获取信息、娱乐和学习的重要途径。然…
阅读更多...
[GXYCTF2019]BabyUpload1

[GXYCTF2019]BabyUpload1

尝试各种文件&#xff0c;黑名单过滤后缀ph&#xff0c;content-type限制image/jpeg 内容过滤<?&#xff0c;木马改用<script languagephp>eval($_POST[cmdjs]);</script> 上传.htaccess将上传的文件当作php解析 蚁剑连接得到flag
阅读更多...
城市开发区视频系统建设方案：打造视频基座、加强图像数据治理

城市开发区视频系统建设方案：打造视频基座、加强图像数据治理

一、背景需求 随着城市建设的步伐日益加快&#xff0c;开发区已经成为了我国工业化、城镇化和对外开放的重要载体。自贸区、开发区和产业园的管理工作自然也变得至关重要。在城市经开区的展览展示馆、进出口商品展示交易中心等地&#xff0c;数千路监控摄像头遍布各角落&#…
阅读更多...
嵌入式第十二天！（指针数组、指针和二维数组的关系、二级指针）

嵌入式第十二天！（指针数组、指针和二维数组的关系、二级指针）

1. 指针数组&#xff1a; int *a[5]; char *str[5]; 指针数组主要用来操作字符串数组&#xff0c;通常将指针数组的每个元素存放字符串的首地址实现对多个字符串的操作。 二维数组主要用来存储字符串数组&#xff0c;通过每行存储一个字符串&#xff0c;多行存储多个字符串所组…
阅读更多...
最新文章
推荐文章

Copyright @ 2022~2023