【opencv】教程代码 —features2D（4）利用两张摄像机拍摄的图片计算单应性矩阵...

homography_from_camera_displacement.cpp

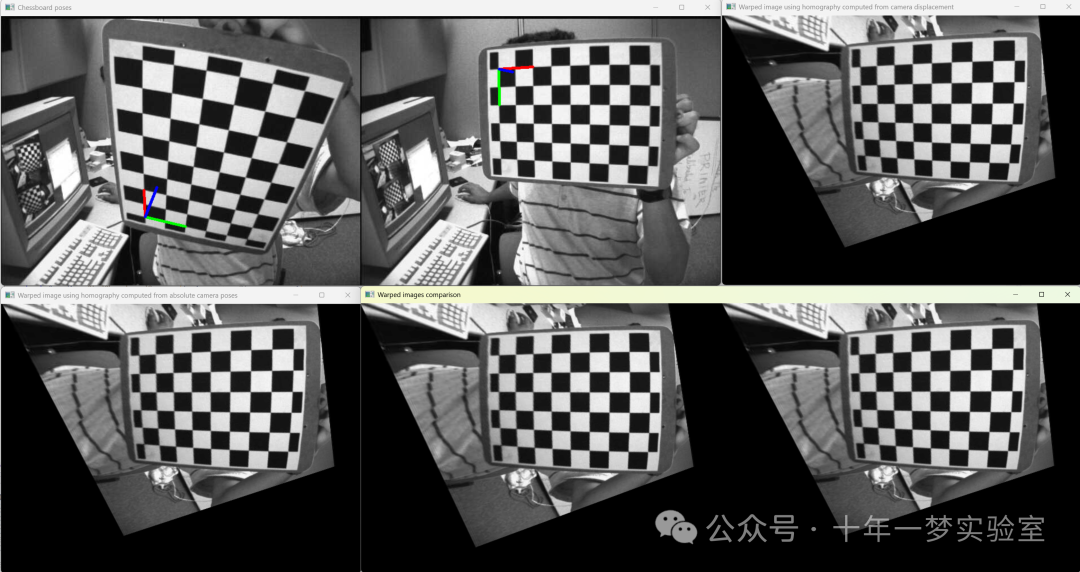
Chessboard poses 棋盘姿态

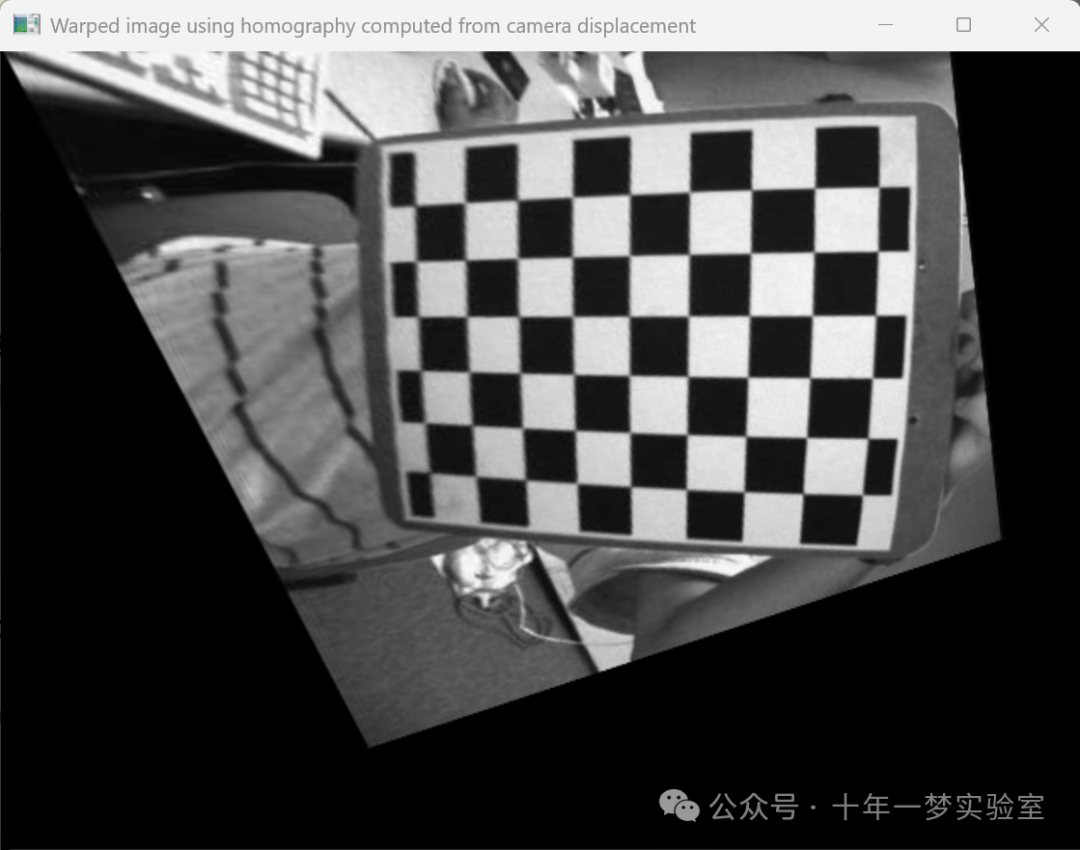
使用根据相机位移计算的单应性扭曲图像

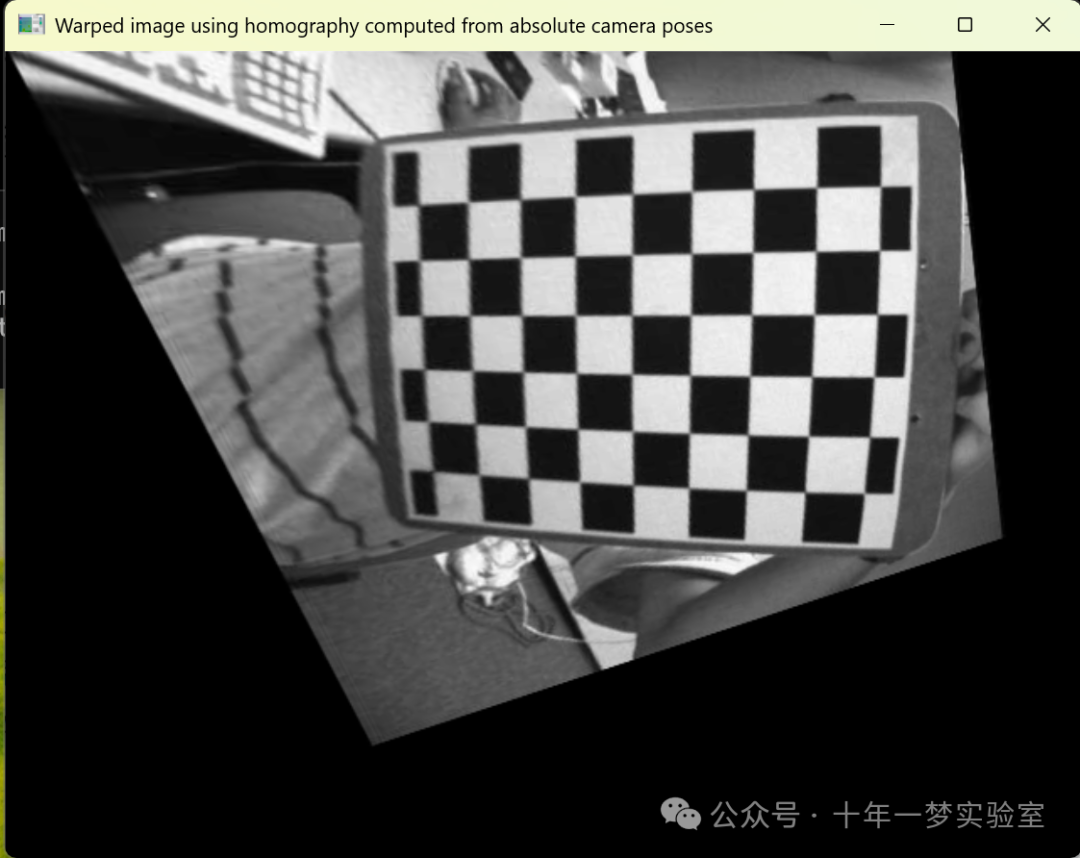
使用根据绝对相机姿势计算的单应性扭曲图像

Warped images comparison 扭曲图像比较

左侧-nfindHomography 右侧-使用根据相机位移计算的单应性扭曲图像

终端输出：

Euclidean Homography:（根据相机位移计算单应性）
[0.2215344870864446, -0.9949332344575121, 0.1140657984013492;0.6776201343947539, 0.1839368762588706, -0.1530250021245755;0.3300066201371719, -0.5683454599466965, 1]
Euclidean Homography 2:（同上，但使用绝对相机姿势而不是相机位移 仅供检查）
[0.2215344870864446, -0.9949332344575118, 0.1140657984013491;0.6776201343947537, 0.1839368762588706, -0.1530250021245755;0.3300066201371718, -0.5683454599466965, 1]findHomography H:
[0.3290339333220099, -1.244138808862929, 536.4769088231476;0.6969763913334047, -0.0893590907257152, -80.3406850408241;0.0004051172959296097, -0.001079740100565012, 1]
homography from camera displacement:（相机位移的单应性）
[0.4160569974777896, -1.306889022263172, 553.7055455031656;0.7917584238390836, -0.06341244817498765, -108.2770026444472;0.0005926357279773245, -0.00102065172285972, 1]
homography from absolute camera poses:（绝对相机姿势的单应性）
[0.4160569974777895, -1.306889022263171, 553.7055455031656;0.7917584238390833, -0.06341244817498759, -108.2770026444472;0.0005926357279773244, -0.00102065172285972, 1]

// 包含必要的库
#include <iostream> // 用于基本输入输出
#include <opencv2/core.hpp> // 包含OpenCV库的核心部分
#include <opencv2/imgproc.hpp> // 包含OpenCV库的图像处理部分
#include <opencv2/highgui.hpp> // 包含OpenCV库的高级GUI部分
#include <opencv2/calib3d.hpp> // 包含OpenCV库的相机标定和3D重建部分using namespace std; // 使用标准命名空间
using namespace cv; // 使用OpenCV命名空间namespace // 定义一个无名命名空间
{
enum Pattern { CHESSBOARD, CIRCLES_GRID, ASYMMETRIC_CIRCLES_GRID }; // 定义一个枚举类型Pattern，包含棋盘、圆形网格和非对称圆形网格三种模式void calcChessboardCorners(Size boardSize, float squareSize, vector<Point3f>& corners, Pattern patternType = CHESSBOARD)
// 定义一个函数，用于计算棋盘角点
{corners.resize(0); // 重置角点向量switch (patternType) // 根据模式类型进行不同的操作{case CHESSBOARD:case CIRCLES_GRID:for( int i = 0; i < boardSize.height; i++ ) // 遍历棋盘的每一行for( int j = 0; j < boardSize.width; j++ ) // 遍历棋盘的每一列corners.push_back(Point3f(float(j*squareSize), // 将计算得到的角点坐标添加到角点向量中float(i*squareSize), 0));break;case ASYMMETRIC_CIRCLES_GRID:for( int i = 0; i < boardSize.height; i++ ) // 遍历棋盘的每一行for( int j = 0; j < boardSize.width; j++ ) // 遍历棋盘的每一列corners.push_back(Point3f(float((2*j + i % 2)*squareSize), // 将计算得到的角点坐标添加到角点向量中float(i*squareSize), 0));break;default:CV_Error(Error::StsBadArg, "Unknown pattern type\n"); // 如果模式类型未知，则抛出错误}
}//! [compute-homography]
Mat computeHomography(const Mat &R_1to2, const Mat &tvec_1to2, const double d_inv, const Mat &normal)
// 定义一个函数，用于计算单应性矩阵
{Mat homography = R_1to2 + d_inv * tvec_1to2*normal.t(); // 计算单应性矩阵return homography; // 返回单应性矩阵
}
//! [compute-homography]Mat computeHomography(const Mat &R1, const Mat &tvec1, const Mat &R2, const Mat &tvec2,const double d_inv, const Mat &normal)
// 定义一个函数，用于计算单应性矩阵
{Mat homography = R2 * R1.t() + d_inv * (-R2 * R1.t() * tvec1 + tvec2) * normal.t(); // 计算单应性矩阵return homography; // 返回单应性矩阵
}//! [compute-c2Mc1]
void computeC2MC1(const Mat &R1, const Mat &tvec1, const Mat &R2, const Mat &tvec2,Mat &R_1to2, Mat &tvec_1to2)
// 定义一个函数，用于计算相机位移
{//c2Mc1 = c2Mo * oMc1 = c2Mo * c1Mo.inv()R_1to2 = R2 * R1.t(); // 计算旋转矩阵tvec_1to2 = R2 * (-R1.t()*tvec1) + tvec2; // 计算平移向量
}
//! [compute-c2Mc1]void homographyFromCameraDisplacement(const string &img1Path, const string &img2Path, const Size &patternSize,const float squareSize, const string &intrinsicsPath)
// 定义一个函数，用于根据相机位移计算单应性矩阵
{Mat img1 = imread( samples::findFile( img1Path ) ); // 读取第一张图片Mat img2 = imread( samples::findFile( img2Path ) ); // 读取第二张图片//! [compute-poses]vector<Point2f> corners1, corners2; // 定义两个角点向量bool found1 = findChessboardCorners(img1, patternSize, corners1); // 在第一张图片中寻找棋盘角点bool found2 = findChessboardCorners(img2, patternSize, corners2); // 在第二张图片中寻找棋盘角点if (!found1 || !found2) // 如果在任何一张图片中都找不到棋盘角点{cout << "Error, cannot find the chessboard corners in both images." << endl; // 输出错误信息return; // 返回}vector<Point3f> objectPoints; // 定义一个对象点向量calcChessboardCorners(patternSize, squareSize, objectPoints); // 计算棋盘角点FileStorage fs( samples::findFile( intrinsicsPath ), FileStorage::READ); // 打开内参文件Mat cameraMatrix, distCoeffs; // 定义相机矩阵和畸变系数fs["camera_matrix"] >> cameraMatrix; // 读取相机矩阵fs["distortion_coefficients"] >> distCoeffs; // 读取畸变系数Mat rvec1, tvec1; // 定义旋转向量和平移向量solvePnP(objectPoints, corners1, cameraMatrix, distCoeffs, rvec1, tvec1); // 求解PnP问题Mat rvec2, tvec2; // 定义旋转向量和平移向量solvePnP(objectPoints, corners2, cameraMatrix, distCoeffs, rvec2, tvec2); // 求解PnP问题//! [compute-poses]Mat img1_copy_pose = img1.clone(), img2_copy_pose = img2.clone(); // 复制两张图片Mat img_draw_poses; // 定义一个用于绘制姿态的图像drawFrameAxes(img1_copy_pose, cameraMatrix, distCoeffs, rvec1, tvec1, 2*squareSize); // 在第一张图片上绘制坐标轴drawFrameAxes(img2_copy_pose, cameraMatrix, distCoeffs, rvec2, tvec2, 2*squareSize); // 在第二张图片上绘制坐标轴hconcat(img1_copy_pose, img2_copy_pose, img_draw_poses); // 将两张图片水平拼接imshow("Chessboard poses", img_draw_poses); // 显示棋盘姿态//! [compute-camera-displacement]Mat R1, R2; // 定义两个旋转矩阵Rodrigues(rvec1, R1); // 将旋转向量转换为旋转矩阵Rodrigues(rvec2, R2); // 将旋转向量转换为旋转矩阵Mat R_1to2, t_1to2; // 定义旋转矩阵和平移向量computeC2MC1(R1, tvec1, R2, tvec2, R_1to2, t_1to2); // 计算相机位移Mat rvec_1to2; // 定义旋转向量Rodrigues(R_1to2, rvec_1to2); // 将旋转矩阵转换为旋转向量//! [compute-camera-displacement]//! [compute-plane-normal-at-camera-pose-1]//平面法线在相机1坐标系下的坐标表示Mat normal = (Mat_<double>(3,1) << 0, 0, 1); // 定义法线向量Mat normal1 = R1*normal; // 计算第一帧的法线向量//! [compute-plane-normal-at-camera-pose-1]//! [compute-plane-distance-to-the-camera-frame-1]//世界坐标系原点在相机1坐标系下的坐标表示origin1 Mat origin(3, 1, CV_64F, Scalar(0)); // 定义原点Mat origin1 = R1*origin + tvec1; // 计算第一帧的原点double d_inv1 = 1.0 / normal1.dot(origin1); // 计算平面到第一帧相机坐标系原点的距离的倒数//! [compute-plane-distance-to-the-camera-frame-1]//!根据相机位移（位姿变化）计算单应性 [compute-homography-from-camera-displacement]Mat homography_euclidean = computeHomography(R_1to2, t_1to2, d_inv1, normal1); // 计算欧几里得单应性矩阵Mat homography = cameraMatrix * homography_euclidean * cameraMatrix.inv(); // 计算单应性矩阵homography /= homography.at<double>(2,2); // 归一化单应性矩阵homography_euclidean /= homography_euclidean.at<double>(2,2); // 归一化欧几里得单应性矩阵//! [compute-homography-from-camera-displacement]//Same but using absolute camera poses instead of camera displacement, just for checkMat homography_euclidean2 = computeHomography(R1, tvec1, R2, tvec2, d_inv1, normal1); // 计算欧几里得单应性矩阵Mat homography2 = cameraMatrix * homography_euclidean2 * cameraMatrix.inv(); // 计算单应性矩阵homography_euclidean2 /= homography_euclidean2.at<double>(2,2); // 归一化欧几里得单应性矩阵homography2 /= homography2.at<double>(2,2); // 归一化单应性矩阵cout << "\nEuclidean Homography:\n" << homography_euclidean << endl; // 输出欧几里得单应性矩阵cout << "Euclidean Homography 2:\n" << homography_euclidean2 << endl << endl; // 输出欧几里得单应性矩阵//! 估计单应性矩阵H  corners2 = H * corners1   [estimate-homography]Mat H = findHomography(corners1, corners2); // 估计单应性矩阵cout << "\nfindHomography H:\n" << H << endl; // 输出估计的单应性矩阵//! [estimate-homography]cout << "homography from camera displacement:\n" << homography << endl; // 输出由相机位移计算得到的单应性矩阵cout << "homography from absolute camera poses:\n" << homography2 << endl << endl; // 输出由绝对相机姿态计算得到的单应性矩阵//! [warp-chessboard]Mat img1_warp; // 定义一个变形后的图像warpPerspective(img1, img1_warp, H, img1.size()); // 对第一张图片进行透视变换//! [warp-chessboard]Mat img1_warp_custom; // 定义一个自定义的变形后的图像warpPerspective(img1, img1_warp_custom, homography, img1.size()); // 对第一张图片进行透视变换imshow("Warped image using homography computed from camera displacement", img1_warp_custom); // 显示由相机位移计算得到的单应性矩阵变形后的图像Mat img_draw_compare; // 定义一个用于比较的图像hconcat(img1_warp, img1_warp_custom, img_draw_compare); // 将两张变形后的图像水平拼接imshow("Warped images comparison", img_draw_compare); // 显示比较后的图像Mat img1_warp_custom2; // 定义一个自定义的变形后的图像warpPerspective(img1, img1_warp_custom2, homography2, img1.size()); // 对第一张图片进行透视变换imshow("Warped image using homography computed from absolute camera poses", img1_warp_custom2); // 显示由绝对相机姿态计算得到的单应性矩阵变形后的图像waitKey(); // 等待用户按键
}const char* params= "{ help h         |       | print usage }""{ image1         | left02.jpg | path to the source chessboard image }""{ image2         | left01.jpg | path to the desired chessboard image }""{ intrinsics     | left_intrinsics.yml | path to camera intrinsics }""{ width bw       | 9     | chessboard width }""{ height bh      | 6     | chessboard height }""{ square_size    | 0.025 | chessboard square size }";// 定义一个参数列表，包含帮助信息、图像路径、内参路径、棋盘宽度、棋盘高度和棋盘方格大小}int main(int argc, char *argv[]) // 主函数
{CommandLineParser parser(argc, argv, params); // 定义一个命令行解析器if (parser.has("help")) // 如果用户请求帮助{parser.about("Code for homography tutorial.\n""Example 3: homography from the camera displacement.\n"); // 输出关于信息parser.printMessage(); // 打印消息return 0; // 返回0}Size patternSize(parser.get<int>("width"), parser.get<int>("height")); // 获取棋盘的尺寸float squareSize = (float) parser.get<double>("square_size"); // 获取棋盘方格的大小homographyFromCameraDisplacement(parser.get<String>("image1"), // 调用函数，计算单应性矩阵parser.get<String>("image2"),patternSize, squareSize,parser.get<String>("intrinsics"));return 0; // 返回0
}

上面的C++代码是使用OpenCV库来实现基于棋盘格的摄像头标定、计算相机姿态、以及基于相机位移计算单应性矩阵。主要步骤如下：