文章目录
- [A - The bottom of the ninth](https://atcoder.jp/contests/abc351/tasks/abc351_a)
- [B - Spot the Difference ](https://atcoder.jp/contests/abc351/tasks/abc351_b)
- [D - Grid and Magnet](https://atcoder.jp/contests/abc351/tasks/abc351_d)
- E
Note:省略模板代码,模版代码在末尾
A - The bottom of the ninth
题目:
给两个序列A和B,求B总和比A总和大时需要加上的最小值
思路:
答案=A总和-B总和+1
static void solve() throws IOException {int[] a = pIntArray(0);int[] b =pIntArray(0);int acnt = 0, bcnt = 0;for (int i = 0; i < a.length; i++) {acnt += a[i];}for (int i = 0; i < b.length; i ++) {bcnt += b[i];}out.println(acnt - bcnt + 1);
}
B - Spot the Difference
题目:
给两个仅包含小写字母的NxN矩阵,找出唯一不同字母的下标对
思路:
模拟
static void solve() throws IOException {int n = pInt(in.readLine());String[] a = new String[n], b = new String[n];for (int i = 0; i < n; i++) {a[i] = in.readLine();}for (int i = 0; i < n; i++) {b[i] = in.readLine();}for (int i = 0; i < n; i++) {for (int j = 0; j < n; j++) {if (a[i].charAt(j) != b[i].charAt(j)) {out.println(i + 1 + " " + (j + 1) );return;}}}
}
C - Merge the balls
题目:
给一个空序列和N个球,第i个球的大小为 2 A i 2^{A_i} 2Ai;执行N次操作,在第i次操作时将第i个球加入到序列的最右边,然后重复以下步骤:当序列有两个及以上的球且最右边的两个球大小相同时,移去这两个球,并加入一个球,其大小为移除的球大小的总和,直到不能执行该步骤为止;
求执行完N次操作后序列还有多少个球
思路:
模拟
static void solve() throws IOException {int n = pInt(in.readLine());long[] balls = pLongArray(1);long[] t = new long[n + 1];int hh = 0;for (int i = 1; i <= n; i ++) {t[++ hh] = balls[i];while (hh > 1 && t[hh] == t[hh - 1]) {t[hh - 1] += 1;hh -= 1;}}out.println(hh);
}
D - Grid and Magnet
题目:
一个HxW的矩阵,某些格子里面会有磁铁,用 # 表示,其余格子为空,用. 表示;
如果在一个有磁铁的格子旁边,则无法继续移动;
问在这个矩阵里面的某个点出发能够移动的最大单元格数量;
思路:
实际上是求最大的连通块的格子数量,但连通块中边缘是一些磁铁,那么可以标记一下磁铁旁边的空单元格,当走到该种单元格时无法继续移动;
剩下的只需要用 BFS 来求连通块的大小即可;
static final int EMPTY = 0;
static final int MAGNET = 1;
static final int STICK = 2;
static final int[] d = new int[] {0, -1, 0, 1, 0};
static int bfs(int h, int w, int sx, int sy, int[][] vis) {Queue<int[]> q = new LinkedList<>();q.offer(new int[] {sx, sy});final int CURRENT_VISITED = 1010 * (sx + 1) + sy;vis[sx][sy] = CURRENT_VISITED;int ans = 0;while (!q.isEmpty()) {int[] u = q.poll();int x = u[0], y = u[1];ans ++;for (int i = 0; i < 4; i ++) {int dx = x + d[i], dy = y + d[i + 1];if (dx < 0 || dx >= h || dy < 0 || dy >= w || vis[dx][dy] == MAGNET || vis[dx][dy] == CURRENT_VISITED) continue;if (vis[dx][dy] == EMPTY) {q.offer(new int[] {dx, dy});vis[dx][dy] = CURRENT_VISITED;} else if (vis[dx][dy] != MAGNET && vis[dx][dy] != CURRENT_VISITED) {ans ++;vis[dx][dy] = CURRENT_VISITED;}}}return ans;
}
static void solve() throws IOException {String[] ins = pStringArray();int h = pInt(ins[0]), w = pInt(ins[1]);char[][] map = new char[h][w];int[][] vis = new int[h][w];for (int i = 0; i < h; i ++) {char[] t = in.readLine().toCharArray();System.arraycopy(t, 0, map[i], 0, w);for (int j = 0; j < w; j ++) {if (map[i][j] == '#') {vis[i][j] = MAGNET;for (int k = 0; k < 4; k++) {int dx = i + d[k], dy = j + d[k + 1];if (dx < 0 || dx >= h || dy < 0 || dy >= w) continue;if (vis[dx][dy] == EMPTY) {vis[dx][dy] = STICK;}}}}}int ans = 1;for (int i = 0; i < h; i ++) {for (int j = 0; j < w; j ++) {if (vis[i][j] == EMPTY) {ans = Math.max(ans, bfs(h, w, i, j, vis));}}}out.println(ans);
}
E
题目:
在二维坐标平面上有 N 个点,兔子只能走对角线,计算AB两个点之间的距离 d i s t ( P A , P B ) dist(P_A, P_B) dist(PA,PB) 为:兔子从A点开始走对角线,到达B点的最小次数;求 ∑ i = 1 N − 1 ∑ j = i + 1 N d i s t ( P i , P j ) \sum^{N-1}_{i=1}\sum^{N}_{j=i + 1} dist(P_i, P_j) ∑i=1N−1∑j=i+1Ndist(Pi,Pj)
思路:
找规律,如下图,兔子从A点开始走,那么红色路线上的点都是可以到达的,注意到,可达点的坐标 ( x , y ) (x,y) (x,y) 相加之后与起点A的坐标相加后模2同余,那么可以对此进行分类;
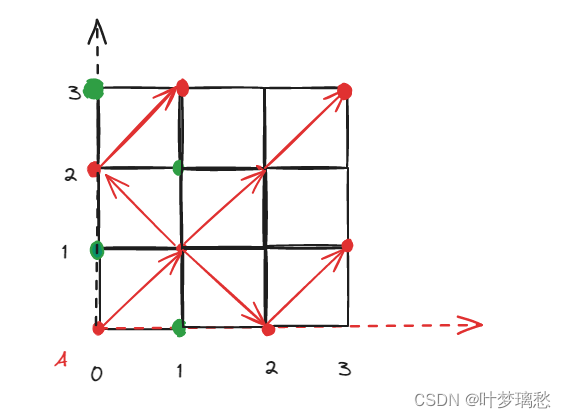
如何进行计算距离呢?
将二维坐标平面旋转45度(坐标可以变换为: x ′ = x + y , y ′ = x − y x'=x+y, y'=x-y x′=x+y,y′=x−y),兔子从走对角线就变成了上下左右走,两个点的距离就变成了水平方向和竖直方向上的距离了;
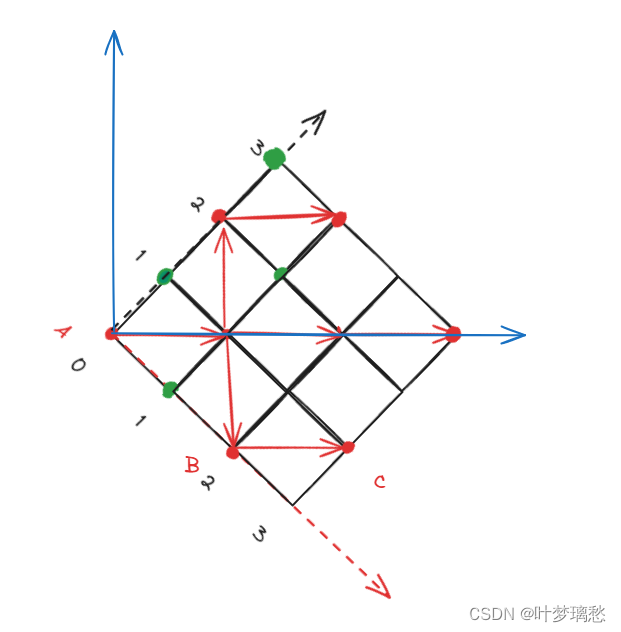
题目数据量是2e5,不可能两重循环来计算两个点的距离,这样会超时;
我们可以将x轴和y轴分开来计算:比如上面的A、B、C点
- 先计算x轴的,A到B的距离为1,B到C的距离为1,因为C点在A点的右边,那么A到C的距离为1+1=2
- 计算y轴的,A到B的距离为1
总的值就是3
需要注意的是,需要对转换后的x轴和y轴坐标排序,保证可以根据前面计算的距离来推出当前的距离(比如上面的A到C点)
static void solve() throws IOException {int n = pInt(in.readLine());List<Integer>[] px = new ArrayList[2];List<Integer>[] py = new ArrayList[2];for (int i = 0; i < 2; i ++) {px[i] = new ArrayList<>();py[i] = new ArrayList<>();}for (int i =0 ; i < n; i ++) {String[] ins = pStringArray();int x = pInt(ins[0]), y = pInt(ins[1]);int t = Math.abs(x + y) % 2;// 根据取模的结果分别拆分x轴和y轴px[t].add( x - y);py[t].add(x + y);}long ans = 0;for (int i = 0; i < 2; i ++) {// 排序,保证坐标小的在前面px[i].sort(Comparator.comparingInt(a -> a));py[i].sort(Comparator.comparingInt(a -> a));int size = px[i].size();long sum1 = 0, sum2 = 0;for (int j = 0; j < size; j ++) {// 当前点到前面其他点位置的距离ans += (long) j * (px[i].get(j) + py[i].get(j)) - sum1 - sum2;sum1 += px[i].get(j);sum2 += py[i].get(j);}}// 要求的值是 0->N-1, i+1->N-1 所以除以2out.println(ans / 2);
}
模版代码:
import java.io.*;
import java.nio.file.Files;
import java.nio.file.Paths;public class Example {static void solve() throws IOException {}public static void main(String[] args) throws IOException {int t = 1;
// t = Integer.parseInt(in.readLine());while (t -- > 0) {solve();}in.close();out.flush();out.close();}private static InputStream is = System.in;static {try {is = Files.newInputStream(Paths.get("F:\\Notes\\Algorithm\\Problems\\java\\java\\src\\main\\java\\input.txt"));} catch (Exception e) {is = System.in;}}private static final BufferedReader in = new BufferedReader(new InputStreamReader(is));private static final PrintWriter out = new PrintWriter(System.out);private static int pInt(String s) {return Integer.parseInt(s);}private static long pLong(String s) {return Long.parseLong(s);}private static String[] pStringArray() throws IOException {return in.readLine().split(" ");}private static int[] pIntArray(int start) throws IOException {String[] s = pStringArray();int[] arr = new int[start + s.length];for (int i = start, j = 0; i < arr.length; i++, j ++) {arr[i] = Integer.parseInt(s[j]);}return arr;}private static long[] pLongArray(int start) throws IOException {String[] s = pStringArray();long[] arr = new long[start + s.length];for (int i = start, j = 0; i < arr.length; i++, j ++) {arr[i] = Long.parseLong(s[j]);}return arr;}
}



![[ESP32]:TFLite Micro推理CIFAR10模型](https://img-blog.csdnimg.cn/direct/ae16a3c0615d4e91b58fd70e30d64cd0.png)


![[蓝桥杯2024]-PWN:fd解析(命令符转义,标准输出重定向)](https://img-blog.csdnimg.cn/direct/fe37ee3eed4841558d05d5ad0f674fc9.png)