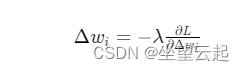这次比赛没打，记错时间了，看了一下，如果去做的话大概也只能做出那两道简单的题，还是太菜啦

EzRSA

题目描述：

from Crypto.Util.number import *
import random
from gmpy2 import *
from libnum import *
from flag import flagdef padding(f):random_chars = bytes([random.randint(0, 255) for _ in range(32)])f = f + random_charsreturn fdef guess_p(p):e = 65537P = pn1 = getPrime(512)*getPrime(512)with open('enc.txt', 'w+') as f:while jacobi(2,n1) == 1:n1 = getPrime(512)*getPrime(512)while P:pad = random.randint(0, 2**2023)**2 message = pad << 1 + P % 2cipher = pow(message, e, n1)f.write(str(cipher)+'n')P //= 2print("n1 = "+ str(n1) )    def guess_q(q):def encrypt(q, n):e = random.randint(1000,2000)noise = random.randint(0, n - 1)c = pow(q+noise,e,n)return e, noise,c n2 = getPrime(512)*getPrime(512)e1, noise1, c1 = encrypt(q, n2)e2, noise2, c2 = encrypt(q, n2)print("n2 = "+ str(n2) ) print('(e1, noise1, c1) =', (e1,noise1,c1))print('(e2, noise2, c2) =', (e2,noise2,c2))
p = getPrime(512)
q = getPrime(512)n = p*q
guess_p(p)
guess_q(q)
e = 0x10001
flag = padding(flag)
m = bytes_to_long(flag)
c = pow(m,e,n)print("c = " + str(c))
'''
n1 = 65634094430927080732256164808833233563732628654160389042977689628512527168256899310662239009610512772020503283842588142453533499954947692968978190310627721338357432052800695091789711809256924541784954080619073213358228083200846540676931341013554634493581962527475555869292091755676130810562421465063412235309
n2 = 103670293685965841863872863719573676572683187403862749665555450164387906552249974071743238931253290278574192713467491802940810851806104430306195931179902098180199167945649526235613636163362672777298968943319216325949503045377100235181706964846408396946496139224344270391027205106691880999410424150216806861393
(e1, noise1, c1) = (1743, 44560588075773853612820227436439937514195680734214431948441190347878274184937952381785302837541202705212687700521129385632776241537669208088777729355349833215443048466316517110778502508209433792603420158786772339233397583637570006255153020675167597396958251208681121668808253767520416175569161674463861719776, 65643009354198075182587766550521107063140340983433852821580802983736094225036497335607400197479623208915379722646955329855681601551282788854644359967909570360251550766970054185510197999091645907461580987639650262519866292285164258262387411847857812391136042309550813795587776534035784065962779853621152905983)
(e2, noise2, c2) = (1325, 35282006599813744140721262875292395887558561517759721467291789696459426702600397172655624765281531167221787036009507833425145071265739486735993631460189629709591456017092661028839951392247601628468621576100035700437892164435424035004463142959219067199451575338270613300215815894328788753564798153516122567683, 50327632090778183759544755226710110702046850880299488259739672542025916422119065179822210884622225945376465802069464782311211031263046593145733701591371950349735709553105217501410716570601397725812709771348772095131473415552527749452347866778401205442409443726952960806789526845194216490544108773715759733714)
c = 124349762993424531697403299350944207725577290992189948388824124986066269514204313888980321088629462472088631052329128042837153718129149149661961926557818023704330462282009415874674794190206220980118413541269327644472633791532767765585035518183177197863522573410860341245613331398610013697803459403446614221369
'''

题目分析：
gen_q:
看到jacobi(2,n1)便知道是二次剩余的知识
$$\begin{gathered} c=(2\ast a^2{)}^e，当P\%2=0 \\ c=(2\ast a^2+1{)}^e，当P\%2=1 \\ {c}^{(n1-1)//2}\equiv (2\ast a^2{)}^{e\ast (n1-1)//2}\equiv -1\ast 1\mathrm{mod}n1 \\ \Rightarrow jacobi(en{c}_i,n1)=-1,p=0+p \end{gathered}$$
gen_q:
相关消息攻击直接解啦

exp:

import binascii
import libnum
from gmpy2 import *
from Crypto.Util.number import *
n1 = 65634094430927080732256164808833233563732628654160389042977689628512527168256899310662239009610512772020503283842588142453533499954947692968978190310627721338357432052800695091789711809256924541784954080619073213358228083200846540676931341013554634493581962527475555869292091755676130810562421465063412235309
(e1, noise1, c1) = (1743, 44560588075773853612820227436439937514195680734214431948441190347878274184937952381785302837541202705212687700521129385632776241537669208088777729355349833215443048466316517110778502508209433792603420158786772339233397583637570006255153020675167597396958251208681121668808253767520416175569161674463861719776, 65643009354198075182587766550521107063140340983433852821580802983736094225036497335607400197479623208915379722646955329855681601551282788854644359967909570360251550766970054185510197999091645907461580987639650262519866292285164258262387411847857812391136042309550813795587776534035784065962779853621152905983)
(e2, noise2, c2) = (1325, 35282006599813744140721262875292395887558561517759721467291789696459426702600397172655624765281531167221787036009507833425145071265739486735993631460189629709591456017092661028839951392247601628468621576100035700437892164435424035004463142959219067199451575338270613300215815894328788753564798153516122567683, 50327632090778183759544755226710110702046850880299488259739672542025916422119065179822210884622225945376465802069464782311211031263046593145733701591371950349735709553105217501410716570601397725812709771348772095131473415552527749452347866778401205442409443726952960806789526845194216490544108773715759733714)
c = 124349762993424531697403299350944207725577290992189948388824124986066269514204313888980321088629462472088631052329128042837153718129149149661961926557818023704330462282009415874674794190206220980118413541269327644472633791532767765585035518183177197863522573410860341245613331398610013697803459403446614221369
n2 = 103670293685965841863872863719573676572683187403862749665555450164387906552249974071743238931253290278574192713467491802940810851806104430306195931179902098180199167945649526235613636163362672777298968943319216325949503045377100235181706964846408396946496139224344270391027205106691880999410424150216806861393ciphers = []
with open('enc.txt') as f:for line in f.read().split('n'):if line.strip():ciphers.append(int(line.strip()))p = ''
for i in ciphers:if jacobi(i,n1) == -1:p = '0' + pelse:p = '1' + pp = int(p,2)def franklinReiter(n,e1,e2,c1,c2,noise1,noise2):PR.<x> = PolynomialRing(Zmod(n))g1 = (x + noise1)^e1 - c1g2 = (x + noise2)^e2 - c2def gcd(g1, g2):while g2:g1, g2 = g2, g1 % g2return g1.monic() # return -gcd(g1, g2)[0]q=franklinReiter(n2,e1,e2,c1,c2,noise1,noise2)
q = 13189337905641321257372188436353844418280745284875462357019668708167547026960641869513283218672677712590326347601424108528959315675307896082223561007980457
p = 9473204278465588641589315677772678997836862033858760337441231265335880892205102590571357305720744128962068300763212493598006400853597404586755248901932203
e = 0x10001
phi = (p - 1) * (q - 1)
d = inverse(e,phi)
print(long_to_bytes(int(pow(c,d,p * q))))
# DASCTF{W05-y03r_m2st1r-j2c0b1_2nd_p01yn0mi2l!}

CB backpack

题目描述：

from random import shuffledef gen_e():e = []for i in range(8):ee = [0]*3+[1]*3shuffle(ee)e += eereturn ee = gen_e()
nbit = len(e) # 48
flag = 'DASCTF{'+sha256(''.join([str(i) for i in e]).encode()).hexdigest()+'}'a = [randint(1,2^nbit) for i in range(nbit)]re = 0
for i in range(nbit):re += e[i]*a[i]print(a)
print(re)

题目分析：
一开始看到，感觉很熟悉啊，这不妥妥的背包加密吗，结果。。。是我想简单了
又涉及到了知识盲区，跟着大佬的wp做了一遍，学习到了
总的来说就是数据给的不够大，直接用背包格解出不来，需要爆破几位，通过jsdn测试密度是否达标（d < 0.9408）
测试了一下，得爆破10位以上才能出结果，尽管爆破8位也满足d < 0.9408，但确实是得不到
这样的话我爆破12位吧

from math import *
n = 37
a = [65651991706497, 247831871690373, 120247087605020, 236854536567393, 38795708921144, 256334857906663, 120089773523233, 165349388120302, 123968326805899, 79638234559694, 259559389823590, 256776519514651, 107733244474073, 216508566448440, 39327578905012, 118682486932022, 263357223061004, 132872609024098, 44605761726563, 24908360451602, 237906955893793, 204469770496199, 7055254513808, 221802659519968, 169686619990988, 23128789035141, 208847144870760, 272339624469135, 269511404473473, 112830627321371, 73203551744776, 42843503010671, 118193938825623, 49625220390324, 230439888723036, 241486656550572, 107149406378865, 233503862264755, 269502011971514, 181805192674559, 152612003195556, 184127512098087, 165959151027513, 188723045133473, 241615906682300, 216101484550038, 81190147709444, 124498742419309]
a = a[11:]
d = n / log2(max(a))
N = ceil(1 / 2 * sqrt(n))
assert d < 0.9408, f"Density should be less than 0.9408 but was {d}."
print(d) # 0.7715984980953817

为了节省时间这里直接逆序，很快，几分钟就出来了

from tqdm import tqdm
a=[65651991706497, 247831871690373, 120247087605020, 236854536567393, 38795708921144, 256334857906663, 120089773523233, 165349388120302, 123968326805899, 79638234559694, 259559389823590, 256776519514651, 107733244474073, 216508566448440, 39327578905012, 118682486932022, 263357223061004, 132872609024098, 44605761726563, 24908360451602, 237906955893793, 204469770496199, 7055254513808, 221802659519968, 169686619990988, 23128789035141, 208847144870760, 272339624469135, 269511404473473, 112830627321371, 73203551744776, 42843503010671, 118193938825623, 49625220390324, 230439888723036, 241486656550572, 107149406378865, 233503862264755, 269502011971514, 181805192674559, 152612003195556, 184127512098087, 165959151027513, 188723045133473, 241615906682300, 216101484550038, 81190147709444, 124498742419309]
re=4051501228761632
A = a[12:]
bits=36
def ju(j):for i in j:if abs(i)!=1:return 0return 1
for i in tqdm(range(2^12,1,-1)):temp=[int(j) for j in bin(i)[2:].zfill(12)]t1,t2=temp[:6],temp[6:12]if sum(t1)!=3 or sum(t2)!=3:continuerr = sum([i*j for i,j in zip(temp,a[:12])])new_re = re - rrM=Matrix(ZZ,bits+1)for i in range(bits):M[i,i]=2M[i,-1]=A[i]for i in range(bits):M[-1,i]=1M[-1,-1]=new_reres=M.LLL()if ju(res[0][:-1]):print('find')print(temp)print(res[0])break

CB ezDHKE
这题很常见也很简单就不说了
CB curve
CB cipher
这两题等有时间再继续复现吧

学习到了爆破+背包格