Java二叉树 (2)

🐵本篇文章将对二叉树的一些基础操作进行梳理和讲解

一、操作简述

int size(Node root);  // 获取树中节点的个数int getLeafNodeCount(Node root);  // 获取叶子节点的个数int getKLevelNodeCount(Node root,int k);  // 获取第K层节点的个数int getHeight(Node root);  // 获取二叉树的高度TreeNode find(Node root, int val);   // 检测值为value的元素是否存在void levelOrder(Node root);  //层序遍历boolean isCompleteTree(Node root)   // 判断一棵树是不是完全二叉树

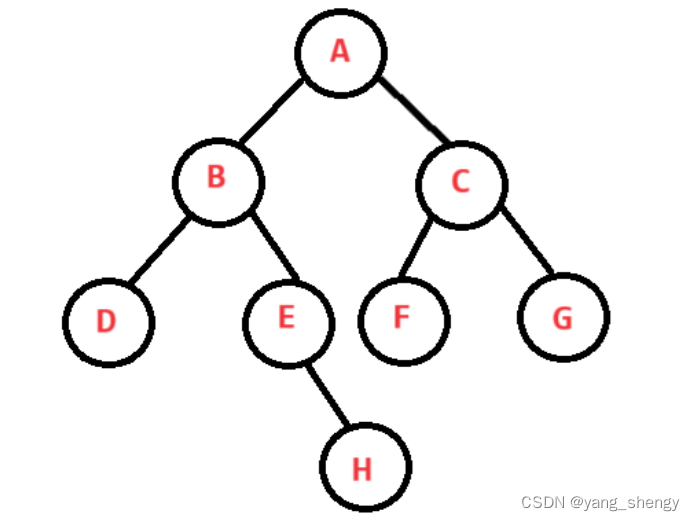
接下来会对下面这棵树进行上述操作:

public class BinaryTree {static class TreeNode {public char val;public TreeNode left;public TreeNode right;public TreeNode(char val) {this.val = val;}}
}

二、代码实现

1.获取树中结点的个数

思路：定义一个nodeSize, 按照二叉树前序遍历的方式遍历这颗二叉树, 每遍历一个结点, nodeSize就+1

    public int nodeSize; //nodeSize不能写到方法内部,否则每次递归nodeSize都会被初始化为0,最终导致结果错误public int size(TreeNode root) {if (root == null) {return 0;}nodeSize++;size(root.left);size(root.right);return nodeSize;}

2. 获取树中叶子结点的个数

思路：叶子结点也就是没有左右孩子的结点，该方法的实现和上一个方法思路大体一致，定义一个leafNode，在遍历这颗二叉树的过程中，如果该节点没有左右孩子则leafNode + 1

    public static int leafNode;public int getLeafNodeCount(TreeNode root) { //计算叶子结点个数if (root == null) {return 0;}if (root.left == null && root.right == null) {leafNode++;}getLeafNodeCount(root.left);getLeafNodeCount(root.right);return leafNode;}

3. 计算k层结点的个数

思路：假如要计算第3层结点的个数，k = 3，整个树的第3层也就是这个树的左子树（B）的第2层+右子树（C）的第2层，也就是B的左子树的第一层 + B的右子树的第一层和C的左子树的第一层 + C的右子树的第一层，通过前序遍历的方式，每遍历到一层k就减1，当k == 1时就返回1

    public int getKLevelNodeCount(TreeNode root,int k) {//计算第k层结点的个数if (root == null) {return 0;}if (k == 1) {return 1;}k--;return getKLevelNodeCount(root.left, k) +getKLevelNodeCount(root.right, k);}

4. 获取树的高度

思路：整个树的高度也就是左子树的高度和右子树的高度的最大值+1，再通过递归的方式求左子树和右子树的高度

    public int getHeight(TreeNode root) {if (root == null) {return 0;}int leftHeight = getHeight(root.left);int rightHeight = getHeight(root.right);return Math.max(leftHeight, rightHeight) + 1;}

5. 检测值为val的元素的结点是否存在

思路：遍历这棵二叉树，找到值为val的结点后逐层返回，直接看代码：

    public TreeNode find(TreeNode root, char val) {if (root == null) {return null;}if (root.val == val) {return root;}TreeNode leftNode = find(root.left, val);//必须用一个变量来接收,否则上述返回的root没有意义,最终返回的还是nullif (leftNode != null) { //leftNode不为空说明找到了，将其返回return leftNode;}TreeNode rightNode = find(root.right, val);if (rightNode != null) {return rightNode;}return null; //没有找到val结点就返回null}

6. 层序遍历二叉树

思路：定义一个队列，先将这个树的根结点入队，之后通过循环如果队列不为空，则让队头结点出队，判断该结点的左和右是否为空，不为空的入队，如此循环知道队列为空，整个二叉树遍历完毕

    public void levelOrder(TreeNode root) {if (root == null) {return;}Queue<TreeNode> queue = new LinkedList<>();queue.offer(root);while(!queue.isEmpty()) {TreeNode cur = queue.poll();System.out.print(cur.val +" ");if(cur.left != null) {queue.offer(cur.left);}if (cur.right != null) {queue.offer(cur.right);}}}

7. 判断一棵树是不是完全二叉树

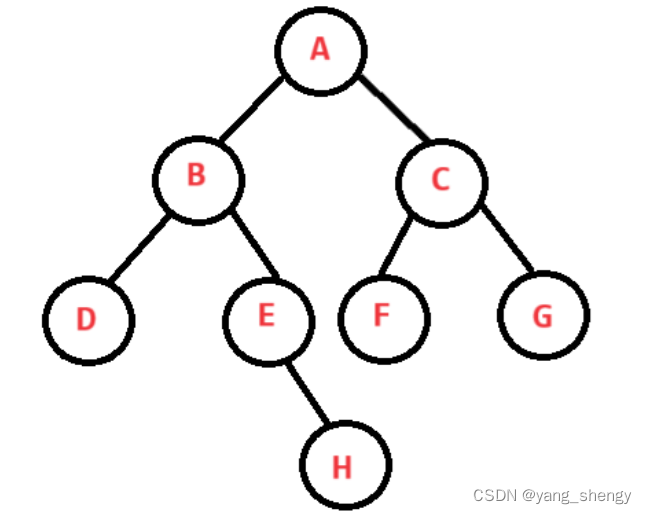
以这棵树为例：

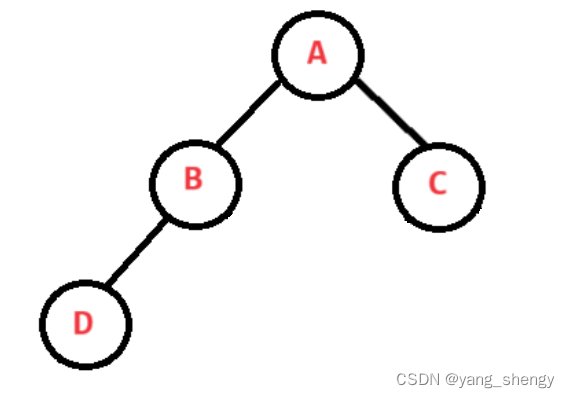
一开始和层序遍历的思路一样，定义一个队列，将树的根结点存入队列中，接下来设置一个循环，当队列不为空的情况下将队头元素出队，如果出队结点不为空则直接将其左右孩子入队（不用判断其左右孩子是否为空）如果出队结点为空则结束该循环

完成上述操作后再设置一个循环，循环条件仍然是队列不为空，每次循环都将队头元素出队然后进行判断，如果该结点不为空，则该树不是完全二叉树

根据上述操作对上面这棵树进行实操

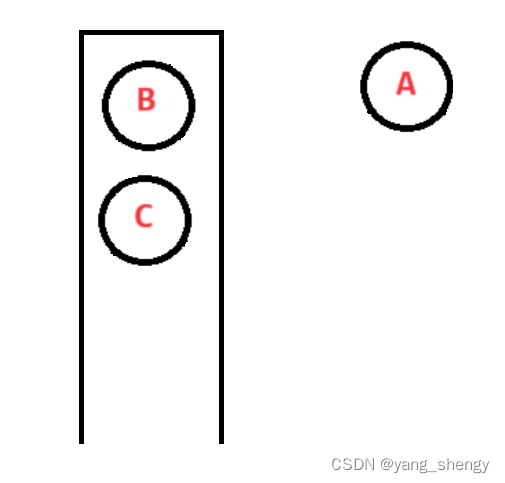
将根结点入队，之后进入循环，将队头元素出队，A结点不为空所以将其左右孩子入队，之后再将队头元素出队，B结点不为空所以再将其左右孩子入队

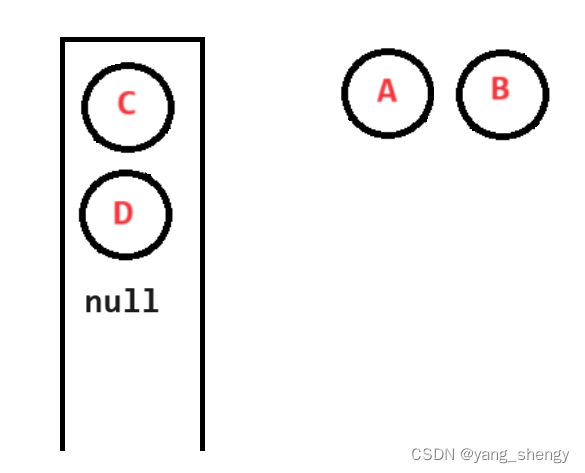
再将C出队，C结点不为空，再将其左右孩子入队，再将D结点出队，D结点不为空，再将其左右孩子入队，之后再将队头元素出队，此时出队的元素为空，此循环结束

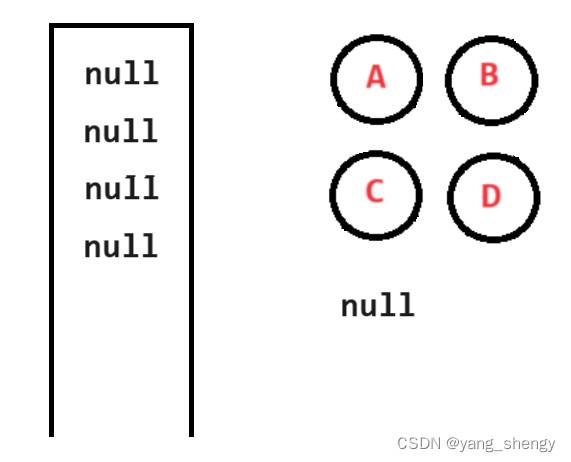
进入第二个循环，只要队列不为空，就出队队头元素然后对其进行判断，只要出队元素不为空，则其不是完全二叉树，上述队列全部为null，所以该树是完全二叉树

如果是下面这棵树，在第一次循环后，会是如下情况：

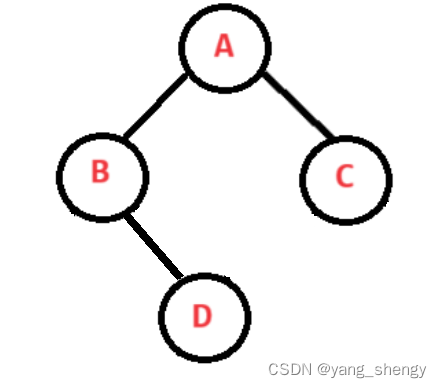
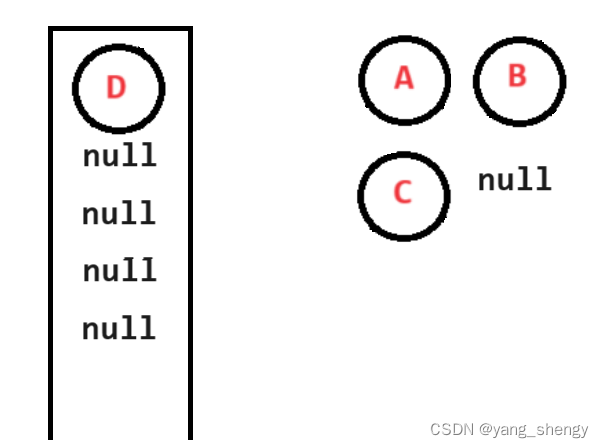
在第二个循环由于D结点不为null，所以该树不是完全二叉树

代码如下：

    public boolean isCompleteTree(TreeNode root) {if (root == null) {return false;}Queue<TreeNode> queue = new LinkedList<>();queue.offer(root);while(!queue.isEmpty()) {TreeNode cur = queue.poll();if (cur == null) {break;}queue.offer(cur.left);queue.offer(cur.right);}while(!queue.isEmpty()) {TreeNode cur = queue.poll();if (cur != null) {return false;}}return true;}