【C++】用红黑树模拟实现set、map

前言及准备：

set、map的底层结构是红黑树，它们的函数通过调用红黑树的接口来实现，红黑树一些接口需要通过树形迭代器来实现。set是k模型，map是kv模型，红黑树要不要写两份呢？答案是不需要，只用一份即可，通过仿函数来控制。

定义树的节点：

template<class T>
struct RBTreeNode
{RBTreeNode<T>* _left;RBTreeNode<T>* _right;RBTreeNode<T>* _parent;T _data;Colour _col;RBTreeNode(const T& data):_left(nullptr),_right(nullptr),_parent(nullptr),_data(data),_col(RED){}
};

红黑树有3个指针域，数据域用T来表示，如果是set，那么传过来的是k模型；如果是map，是kv模型。新增的节点的颜色默认是红色（根节点除外）。

一、红黑树接口

1.1 begin

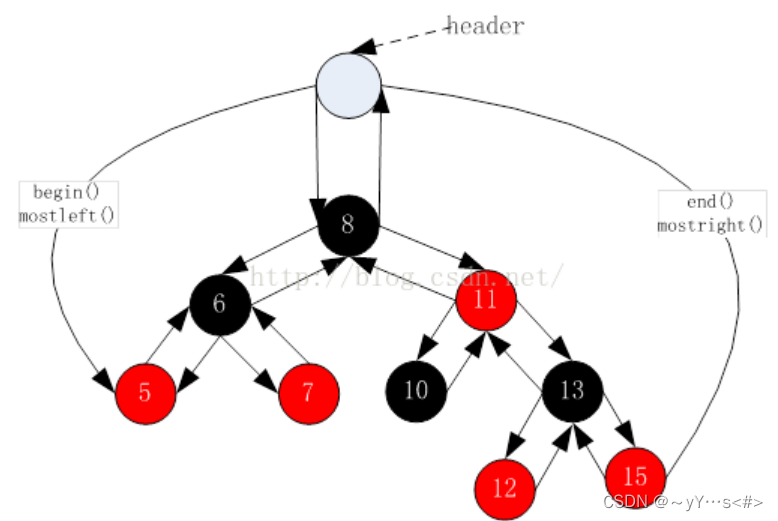
返回的是红黑树的第一个节点，注意，这里的第一个的顺序是按中序遍历来的，所以，第一个节点的位置是树的最左节点。

//返回的迭代器指向的数据可修改
iterator begin()
{Node* subLeft = _root;while (subLeft->_left){subLeft = subLeft->_left;}return iterator(subLeft);
}
//返回的迭代器指向的数据不可修改
const_iterator begin() const
{Node* subLeft = _root;while (subLeft->_left){subLeft = subLeft->_left;}return const_iterator(subLeft);
}

1.2 end

返回的是最后一个节点（最右侧节点）的下一个位置。由于这里实现的红黑树没有头节点，所以只能给nullptr来勉强实现这个迭代器。但是这样其实是不行的，因为对end()位置的迭代器进行 - - 操作，必须要能找最后一个元素，给nullptr就找不到了。如果有头节点，那么end()的位置应该是在头节点的位置。
在这里插入图片描述

iterator end()
{return iterator(nullptr);
}
const_iterator end() const
{return const_iterator(nullptr);
}

1.3 查找

查找的过程与之前写的二叉搜索树没有多大区别，要注意的是返回类型，找到了，返回的是该节点的迭代器，找不到就返回end()。

iterator Find(const K& key)
{KeyOfT kot;Node* cur = _root;while (cur){if (kot(cur->_data) < key){cur = cur->_right;}else if (kot(cur->_data) > key){cur = cur->_left;}else{return iterator(cur);}}return end();
}

咋知道是set还是map的数据进行比较，看传过来的类模板参数中的仿函数是set的还是map的。当然，这里只需写好就行，不用关心传过来的是什么，set和map的仿函数内部已经确定好了。

说明一下：

template<class K, class T, class KeyOfT>

这是该红黑树的类模板，K是Find()函数中要对比的数据类型，T是节点的数据域，可能是k模型，也有可能是kv模型。怎么确定呢？通过第三个类模板参数——仿函数来确定。仿函数传的是set的，就是k模型；仿函数传的是map的，就是kv模型。仿函数内部具体实现下面再说。

1.4 插入

为了接近STL库中的insert函数，返回类型是pair，即插入成功，返回该节点的迭代器和true；插入失败，说明该节点已经存在，返回该节点的迭代器和false。

pair<iterator, bool> Insert(const T& data)
{//为空if (_root == nullptr){_root = new Node(data);_root->_col = BLACK;//根节点都是黑色的，特殊处理return make_pair(iterator(_root), true);}//非空KeyOfT kot;Node* cur = _root;Node* parent = nullptr;while (cur){if (kot(cur->_data) < kot(data)){parent = cur;cur = cur->_right;}else if (kot(cur->_data) > kot(data)){parent = cur;cur = cur->_left;}else{return make_pair(iterator(cur), false);}}//插入新节点cur = new Node(data);//红色的if (kot(parent->_data) < kot(data)){parent->_right = cur;}else{parent->_left = cur;}cur->_parent = parent;Node* newnode = cur;//调整颜色while (parent && parent->_col == RED){Node* grandfather = parent->_parent;//爷爷节点//父节点在爷爷节点的左边，那么叔叔节点在右边if (parent == grandfather->_left){Node* uncle = grandfather->_right;//情况一：叔叔存在且为红if (uncle && uncle->_col == RED){grandfather->_col = RED;uncle->_col = parent->_col = BLACK;cur = grandfather;//爷爷不是根，向上更新parent = cur->_parent;}//情况二：叔叔不存在/存在且为黑else{//单旋if (cur == parent->_left){RotateR(grandfather);//右单旋parent->_col = BLACK;//变色grandfather->_col = RED;}//左右双旋 // cur == parent->_rightelse{RotateL(parent);//先左单旋RotateR(grandfather);//再右单旋grandfather->_col = RED;//变色cur->_col = BLACK;}}}else//父节点在右边，叔叔在左边{Node* uncle = grandfather->_left;//情况一：叔叔存在且为红if (uncle && uncle->_col == RED){grandfather->_col = RED;uncle->_col = parent->_col = BLACK;cur = grandfather;//爷爷不是根，向上更新parent = cur->_parent;}//情况二：叔叔不存在/存在且为黑else{//单旋if (cur == parent->_right){RotateL(grandfather);//左单旋parent->_col = BLACK;//变色grandfather->_col = RED;}//右左双旋 // cur == parent->_leftelse{RotateR(parent);//先右单旋RotateL(grandfather);//再左单旋grandfather->_col = RED;//变色cur->_col = BLACK;}break;//经过情况二后跳出}}}_root->_col = BLACK;//统一处理，根必须是黑的return make_pair(iterator(newnode), true);
}

1.5 左单旋和右单旋

这两个就是之前的，这里不作重复叙述了

//左单旋
void RotateL(Node* parent)
{Node* subR = parent->_right;Node* subRL = subR->_left;parent->_right = subRL;//不为空if (subRL){subRL->_parent = parent;}subR->_left = parent;Node* ppnode = parent->_parent;parent->_parent = subR;//处理parent如果为根if (parent == _root){_root = subR;subR->_parent = nullptr;}//不为根，处理与ppnode的连接else{if (ppnode->_left == parent){ppnode->_left = subR;}else{ppnode->_right = subR;}subR->_parent = ppnode;}
}//右单旋
void RotateR(Node* parent)
{Node* subL = parent->_left;Node* subLR = subL->_right;parent->_left = subLR;//不为空if (subLR){subLR->_parent = parent;}subL->_right = parent;Node* ppnode = parent->_parent;parent->_parent = subL;if (parent == _root){_root = subL;subL->_parent = nullptr;}else{if (ppnode->_left == parent){ppnode->_left = subL;}else{ppnode->_right = subL;}subL->_parent = ppnode;}
}

二、树形迭代器（正向）

2.1 前置++

首先要清楚的是++到下一个节点位置是按中序遍历走的，主要有两种情况：

该节点有右子树
该节点没有右子树

1️⃣有右子树
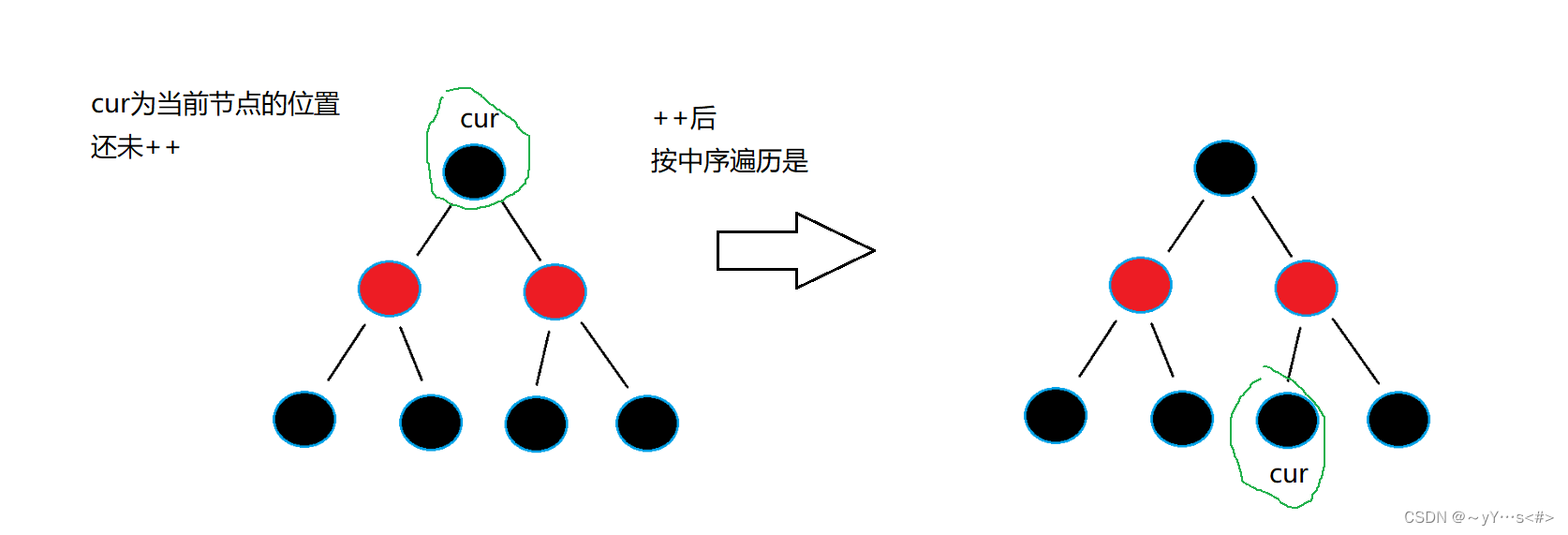
在这里插入图片描述
总结：有右子树++后的下一个节点是右子树的最左节点

2️⃣没有右子树
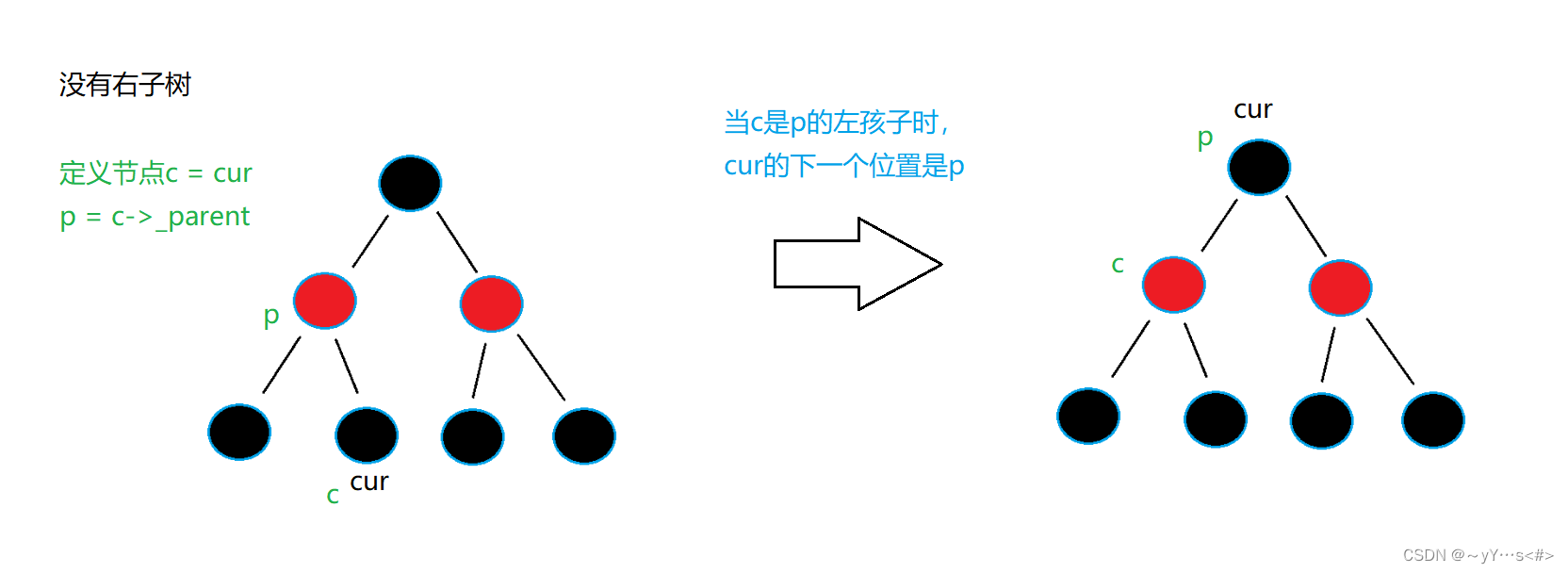
在这里插入图片描述
总结：没有右子树++后下一个节点是祖先节点中左孩子是当前节点（原来节点的位置）或者左孩子是当前节点的父亲的那个祖先

有点弯，再来图捋一捋：
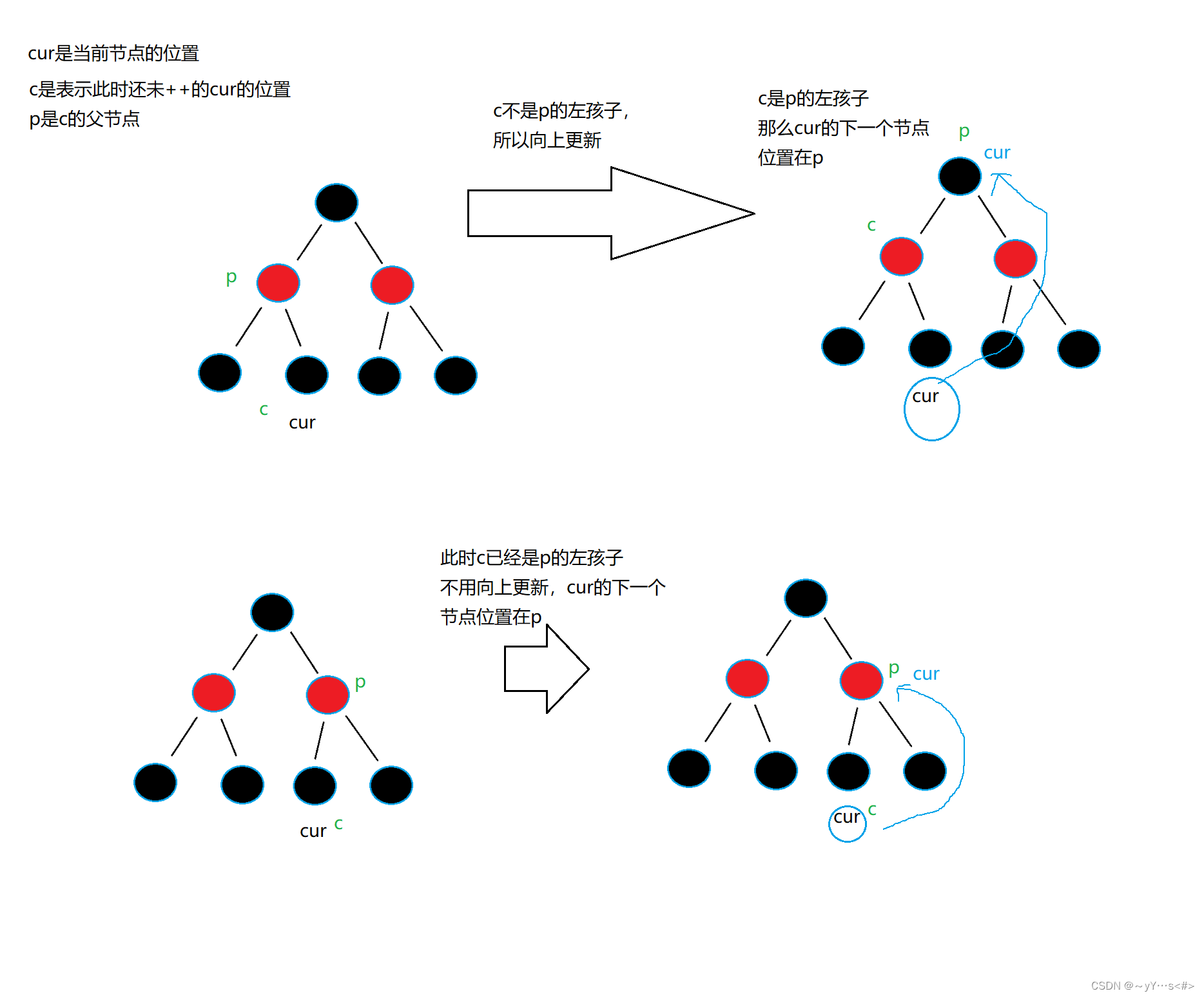
在这里插入图片描述

前置- -的逻辑与前置++刚好相反

template<class T, class Ref, class Ptr>
struct RBTreeIterator
{typedef RBTreeNode<T> Node;typedef RBTreeIterator<T, Ref, Ptr> Self;Node* _node;RBTreeIterator(Node* node):_node(node){}Ref operator*(){return _node->_data;}Ptr operator->(){return &_node->_data;}//前置++Self& operator++(){//右子树存在if (_node->_right){//下一个节点在右子树的最左边Node* subLeft = _node->_right;while (subLeft->_left){subLeft = subLeft->_left;}_node = subLeft;}//右子树不存在else{Node* cur = _node;Node* parent = cur->_parent;//cur是parent的左子树，parent就是下一个while (parent && parent->_right == cur){cur = parent;parent = parent->_parent;}_node = parent;}return *this;}//前置--Self& operator--()//与前置++的逻辑相反{//左子树存在if (_node->_left){//下一个节点是左子树的最右一个Node* subRight = _node->_left;while (subRight->_right){subRight = subRight->_right;}_node = subRight;}//左子树不存在else{Node* cur = _node;Node* parent = cur->_parent;//cur是parent的右子树时parent就是下一个while (parent && parent->_left == cur){cur = parent;parent = parent->_parent;}_node = parent;}return *this;}bool operator!=(const Self& s){  return _node != s._node;}bool operator==(const Self& s){return _node == s._node;}
};

三、模拟实现set

set是k模型，仿函数返回的只有key值。其他接口调用红黑树的

template<class K>
class set
{//仿函数struct SetKeyOfT{const K& operator()(const K& key){return key;}};
public:typedef typename RBTree<K, const K, SetKeyOfT>::iterator iterator;typedef typename RBTree<K, const K, SetKeyOfT>::const_iterator const_iterator;//迭代器iterator begin(){return _t.begin();}const_iterator begin() const{return _t.begin();}iterator end(){return _t.end();}const_iterator end() const{return _t.end();}//插入pair<iterator, bool> Insert(const K& key){return _t.Insert(key);}//查找iterator Find(const K& key){_t.Find(key);}
private:RBTree<K, const K, SetKeyOfT> _t;
};

四、模拟实现map

map是kv模型，仿函数返回的取kv中的key值。其他接口调用红黑树的，除此之外，多了一个operator[]

template<class K, class V>
class map
{struct MapKeyOfT{const K& operator()(const pair<K, V>& kv){return kv.first;}};public:typedef typename RBTree<K, pair<const K, V>, MapKeyOfT>::iterator iterator;typedef typename RBTree<K, pair<const K, V>, MapKeyOfT>::const_iterator const_iterator;//迭代器iterator begin(){return _t.begin();}const_iterator begin() const{return _t.begin();}iterator end(){return _t.end();}const_iterator end() const{return _t.end();}//插入pair<iterator, bool> Insert(const pair<K, V>& kv){return _t.Insert(kv);}//查找iterator Find(const K& key){_t.Find(key);}//operator[]V& operator[](const K& key){pair<iterator, bool> ret = Insert(make_pair(key, V()));return ret.first->second;}
private:RBTree<K, pair<const K, V>, MapKeyOfT> _t;
};