我们知道2维的旋转变换公式为
$$Q=\left(\begin{array}{cc}\cos \left(\theta \right)& \sin \left(\theta \right)\\ -\sin \left(\theta \right)& \cos \left(\theta \right)\end{array}\right)$$
如果$y=Q^{T}x$，那么逆时针旋转x就得到了y。旋转矩阵可以看我的其他博客3维旋转的3种表示方法之间的关系，还有从几何与代数的角度推算坐标旋转变换矩阵(以2维为例)

而镜像变换，是点x以某个向量为轴，得到其镜像y。
$$Q=\left(\begin{array}{cc}\cos \left(\theta \right)& \sin \left(\theta \right)\\ \sin \left(\theta \right)& -\cos \left(\theta \right)\end{array}\right)$$
该镜像变换以向量$(cos(\theta /2),sin(\theta /2))$为轴

镜像变换是对称矩阵，因此$y=Q^{T}x=Qx$

该镜像变换矩阵是怎么来的呢，下面进行推导。假设需要镜像的点为A$(x_0,y_0)$，镜像后得到的点为B$(x_1,y_1)$
那么就有下面两个约数（1）$\overrightarrow{AB}$垂直与向量，（2）A到向量的距离与B到向量的距离相等，并且方向相反。
根据条件(1)
$$\left(x_0-x_1\right)\cos \frac{\theta }{2}+\left(y_0-y_1\right)\sin \frac{\theta }{2}=0$$

向量$(cos(\theta /2),sin(\theta /2))$可以看做一条直线，该直线为
$$-\sin \frac{\theta }{2}x+\cos \frac{\theta }{2}y=0$$
A到该直线距离为
$$f1=-\sin \frac{\theta }{2}{x}_0+\cos \frac{\theta }{2}{y}_0$$
B到该直线距离为
$$f2=-\sin \frac{\theta }{2}{x}_1+\cos \frac{\theta }{2}{y}_1$$
条件(2)为
$$f1=-f2$$
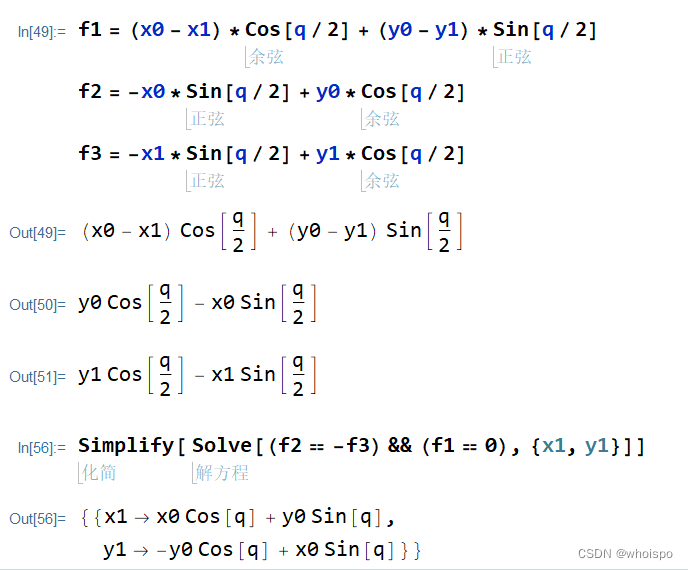
根据解这两个方程，就可以得到上面的镜像变换矩阵Q，我用mathematica验证，确实如此