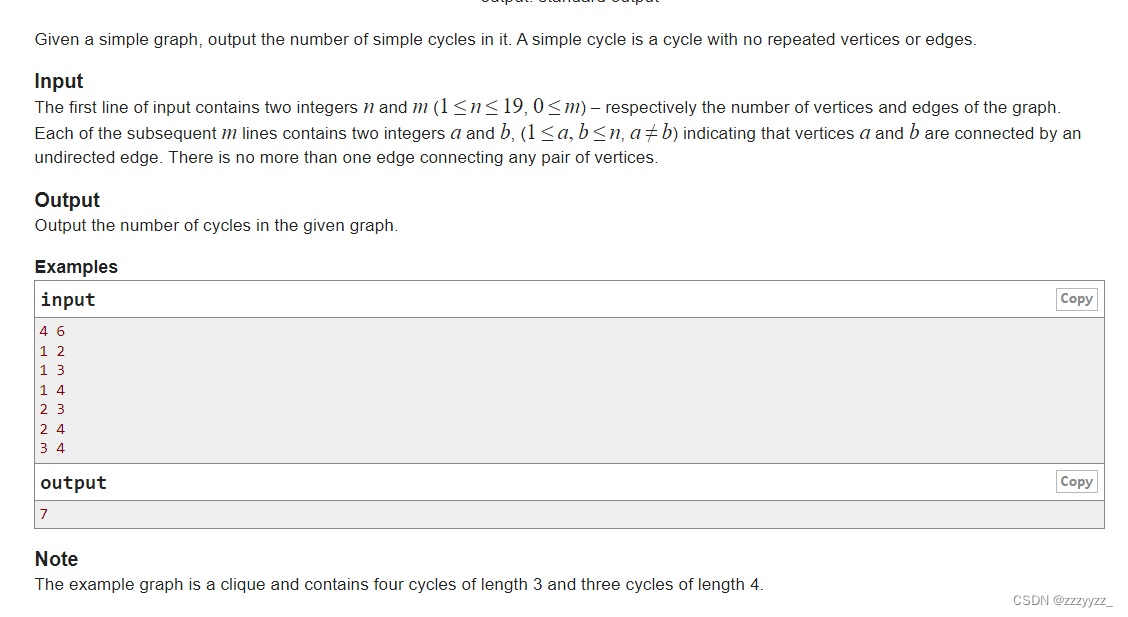
Problem - D - Codeforces

思路：这个题就是求环的数量，通过数据范围的大小，我们可以想到用状压dp来做，因为只有19个点，我们可以将环的路径进行状态压缩，用一个二进制数表示环，当某一位为1时表示这个点在环上，那么我们可以用f[i][j]表示当前的路径状态为i，当前所在的点为j的情况，为了让环不重复统计，那么我们可以将路径中最小的点作为起点，可以保证不重复，那么我们只需要将状态从小到大枚举，这样一定会枚举到所有的情况，因为i一定是变大的不会变小，然后再枚举现在再哪个位置了，再枚举接下来要走到哪个点，那么如果当前的位置或者要到的位置比起点的编号小了，那么就跳过，并且如果从当前位置不能够走到下一个位置也跳过，并且我们再更新i的过程中要保证路径i没有环，因为如果存在环会重复统计。       

// Problem: D. A Simple Task
// Contest: Codeforces - Codeforces Beta Round 11
// URL: https://codeforces.com/contest/11/problem/D
// Memory Limit: 256 MB
// Time Limit: 2000 ms#include<bits/stdc++.h>
#include<sstream>
#include<cassert>
#define fi first
#define se second
#define i128 __int128
using namespace std;
typedef long long ll;
typedef double db;
typedef pair<int,int> PII;
const double eps=1e-7;
const int N=5e5+7 ,M=5e5+7, INF=0x3f3f3f3f,mod=1e9+7,mod1=998244353;
const long long int llINF=0x3f3f3f3f3f3f3f3f;
inline ll read() {ll x=0,f=1;char c=getchar();while(c<'0'||c>'9') {if(c=='-') f=-1;c=getchar();}
while(c>='0'&&c<='9') {x=(ll)x*10+c-'0';c=getchar();} return x*f;}
inline void write(ll x) {if(x < 0) {putchar('-'); x = -x;}if(x >= 10) write(x / 10);putchar(x % 10 + '0');}
inline void write(ll x,char ch) {write(x);putchar(ch);}
void stin() {freopen("in_put.txt","r",stdin);freopen("my_out_put.txt","w",stdout);}
bool cmp0(int a,int b) {return a>b;}
template<typename T> T gcd(T a,T b) {return b==0?a:gcd(b,a%b);}
template<typename T> T lcm(T a,T b) {return a*b/gcd(a,b);}
void hack() {printf("\n----------------------------------\n");}int T,hackT;
int n,m,k;
bool st[30][30];
ll f[1<<20][20];int lowbit(int x) {return x&-x;
}void solve() {n=read(),m=read();for(int i=1;i<=m;i++) {int a=read(),b=read();st[a-1][b-1]=st[b-1][a-1]=true;}	for(int i=0;i<n;i++) f[1<<i][i]=1;ll res=0;for(int i=0;i<(1<<n);i++) {int start=lowbit(i);for(int j=0;j<n;j++) {if((1<<j)<start) continue;for(int k=0;k<n;k++) {if((1<<k)<start) continue;if(!st[j][k]) continue;if(start==(1<<k)) {res+=f[i][j];}else if(!((i>>k)&1)){f[i|(1<<k)][k]+=f[i][j];}}}}res=(res-m)/2;printf("%lld\n",res);
}int main() {// init();// stin();// ios::sync_with_stdio(false); // scanf("%d",&T);T=1; while(T--) hackT++,solve();return 0;       
}