题目描述

给定一种规律 pattern 和一个字符串 s ，判断 s 是否遵循相同的规律。

这里的 遵循 指完全匹配，例如， pattern 里的每个字母和字符串 s 中的每个非空单词之间存在着双向连接的对应规律。
提示:

1 <= pattern.length <= 300
pattern 只包含小写英文字母
1 <= s.length <= 3000
s 只包含小写英文字母和 ' '
s 不包含 任何前导或尾随对空格
s 中每个单词都被 单个空格 分隔

题目分析

首先判断pattern串的长度与s串的单词数是否相等，如果不相等那么这两个字符串的模式必然不一样，如果相等了在继续进行下一项的判断，因为总共有26个小写字母，所以我们可以利用字母的值为串的下标将单词存储起来，如果字母一样就检查当前单词是否出现过，如果没有出现过记录下来此单词，如果已经出现过了那么就将当前单词与已出现的单词作比较，如果相同那么目前的模式仍然一样。依次比对下去即可。

代码

bool wordPattern(char* pattern, char* s) {char *x[26];for(int i=0; i<26; i++){x[i] = NULL;}int pattern_length = 0;while(pattern[pattern_length++]!='\0');pattern_length--;int k = 0;int count = 0;for(;s[k]!='\0'; k++){if(s[k]==' '){count++;}}if(k!=0){count++;}if(count!=pattern_length){return false;}int i=0; int j=0;for(i=0; pattern[i]!='\0'; i++){int length_word = 0;if(x[pattern[i]-'a']==NULL){while(s[j+length_word]!=' '&&s[j+length_word]!='\0'){length_word++;}x[pattern[i]-'a'] = (char *)malloc(sizeof(char)*(length_word+1));for(int k=0; k<length_word; k++){x[pattern[i]-'a'][k] = s[j+k];}x[pattern[i]-'a'][length_word] = '\0';j = j + length_word+1;   }else{char *y = NULL;while(s[j+length_word]!=' '&&s[j+length_word]!='\0'){length_word++;}y = (char *)malloc(sizeof(char)*(length_word+1));for(int k=0; k<length_word; k++){y[k] = s[j+k];}y[length_word] = '\0';j = j + length_word+1;if(strcmp(x[pattern[i]-'a'], y)){return false;}}}for(int i=0; i<26; i++){for(int j=i+1; j<26; j++){if(x[i]!=NULL&&x[j]!=NULL){if(!strcmp(x[i], x[j])){return false;}}}}return true;
}

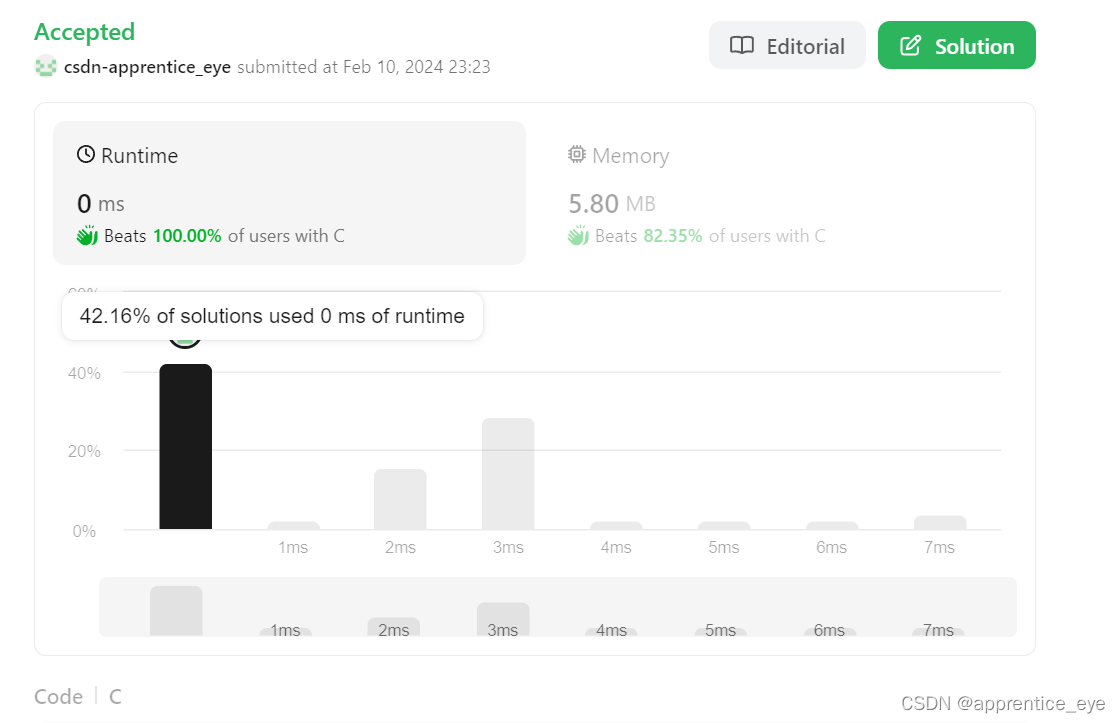
提交结果截图