题目链接
UVa11595 - Crossing Streets EXTREME
题意
平面上有 n(n≤35)条直线,各代表一条街道。街道相互交叉,形成一些路段(对应于几何上的线段)。你的任务是设计一条从A到B的路线,使得穿过路段的拥挤值之和尽量小。为了安全,你的路线不能直接穿过街道的交叉点,也不能沿着街道走,而只能从路段的中间过街。
平面上还有c(c≤1000)座大楼。正常情况下,每条路段的拥挤值为1,但如果和一座大楼相邻(即可以从这座大楼出发,不经过其他路段或者交叉点到达这条路段),拥挤值需要加上该大楼的拥挤度。

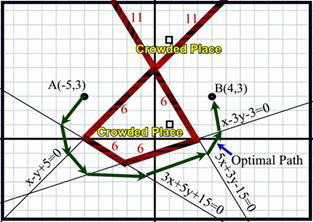
分析
综合了平面区域分割和加权最短路的繁琐题目。
本题解决平面区域分割采用“卷包裹”算法比切割多边形算法更合适。根据本题的数据特点,加权最短路用Dijkstra比SPFA更合适一点。
说一些细节:
1、区域的边界[-M,M],M取值多少合适?不要被题目诱导直接取1000,这其实是错的,取1000000也不严谨,应该取2e9。
2、区域的边界值很大,伴随而来的套平面几何算法模板的一些与0比较相关的函数eps需要调整,分析下来应该取1e-3或1e-4。
3、对每条线段(所有直线加上4条边界裁剪后变成线段)计算它与其他线段的交点,需要去重索引起来,后面分割出多边形也只用点的索引保存,这能为后续处理带来便利。
4、卷包裹分割出各个多边形后,怎么高效判断多边形相邻(有公共边)?每个多边形的边,要么只属于它(在外边界),要么还属于另外一个多边形,可以用map<pair<int, int>, int> mp来记录关联。比如某个多边形x有边pair(u, v),则包含此边的另外一个多边形y = mp[pair(v, u)]。
5、根据多边形相邻关系及多边形包含的大楼建加权图,对每个查询用Dijkstra求解。
给一份测试数据。
更多繁琐细节,参见AC代码。
AC代码
#include <iostream>
#include <cmath>
#include <cstring>
#include <map>
#include <algorithm>
#include <queue>
using namespace std;#define eps 1e-3
struct Point {double x, y;Point(double x = 0., double y = 0.): x(x), y(y) {}
};
typedef Point Vector;Vector operator+ (const Vector& A, const Vector& B) {return Vector(A.x + B.x, A.y + B.y);
}Vector operator- (const Vector& A, const Vector& B) {return Vector(A.x - B.x, A.y - B.y);
}Vector operator* (const Vector& A, double p) {return Vector(A.x * p, A.y * p);
}int dcmp(double x) {return abs(x) < eps ? 0 : (x < 0. ? -1 : 1);
}bool operator== (const Point& a, const Point& b) {return dcmp(a.x - b.x) == 0 && dcmp(a.y - b.y) == 0;
}double Cross(const Vector& A, const Vector& B) {return A.x * B.y - A.y * B.x;
}double Dot(const Vector& A, const Vector& B) {return A.x * B.x + A.y * B.y;
}double LineInterp(const Point& P, const Vector& v, const Point& Q, const Vector& w) {Vector u = P - Q;return Cross(w, u) / Cross(v, w);
}#define M 2.1e9
#define N 42
struct node {int h, i;bool operator< (const node& rhs) const {return h > rhs.h;}
};
int g[N*N>>1][N<<1], t[N*N>>1], u[N*N>>1][N], e[N*N>>1], w[N*N>>1], h[N*N>>1], m, n, c, q, kase = 0;
Point p[N*N>>1], s[N]; Vector v[N], d[N*N>>1][N<<1]; double l[N], f[N]; bool vis[N*N>>1][N<<1];int addPoint(const Point& x) {for (int i=0; i<n; ++i) if (p[i] == x) return i;p[n] = x;return n++;
}void dfs(int x, int i, int s) {vis[x][i] = true;if (x == s) u[m][0] = s, e[m] = 1;int y = g[x][i];if (y != s) {u[m][e[m]++] = y;double c = 1., f; int z = -1;for (int j=0; j<t[y]; ++j)if (!vis[y][j] && Cross(d[x][i], d[y][j]) > 0. && (f = Dot(d[x][i], d[y][j])) < c) c = f, z = j;if (z >= 0) dfs(y, z, s);for (int j=0; j<t[y]; ++j) if (!vis[y][j]) dfs(y, j, y);} else u[m][e[m]] = s, w[m++] = 0;
}int find(const Point& t) {for (int i=0, wn; i<m; ++i) {for (int j=wn=0; j<e[i]; ++j) {int k = dcmp(Cross(p[u[i][j+1]]-p[u[i][j]], t-p[u[i][j]]));int d1 = dcmp(p[u[i][j]].y - t.y);int d2 = dcmp(p[u[i][j+1]].y - t.y);if (k > 0 && d1 <= 0 && d2 > 0) wn++;if (k < 0 && d2 <= 0 && d1 > 0) wn--;}if (wn) return i;}return m;
}void addW() {Point t; int c; cin >> t.x >> t.y >> c; w[find(t)] += c;
}int query() {Point s, u; cin >> s.x >> s.y >> u.x >> u.y;int x = find(s), y = find(u);if (x == y) return 0;memset(h, 10, sizeof(h)); h[x] = 0;priority_queue<node> q; q.push({0, x});while (!q.empty()) {node z = q.top(); q.pop(); int i = z.i;if (i == y) return h[y];if (z.h > h[i]) continue;for (int j=0; j<t[i]; ++j) {int k = g[i][j], v = h[i] + w[i] + w[k] + 1;if (h[k] > v) h[k] = v, q.push({h[k], k});}}return h[y];
}void solve() {m = 4;while (n--) {int a, b, c; cin >> a >> b >> c;if (a == 0) {if (abs(c) >= M*abs(b)) continue;s[m] = {-M, c/(double)-b}; v[m] = {1., 0.}; l[m++] = 2.*M;} else if (b == 0) {if (abs(c) >= M*abs(a)) continue;s[m] = {c/(double)-a, -M}; v[m] = {0., 1.}; l[m++] = 2.*M;} else {double d = sqrt(a*a + b*b); s[m] = {-M, (a*M-c)/b}; v[m] = {abs(b)/d, b>0 ? -a/d : a/d};f[0] = 0.; f[1] = Dot(Point(M, -(a*M+c)/b) - s[m], v[m]); f[2] = Dot(Point((b*M-c)/a, -M) - s[m], v[m]);f[3] = Dot(Point(-(b*M+c)/a, M) - s[m], v[m]); sort(f, f+4);p[1] = s[m] + v[m]*f[1]; p[2] = s[m] + v[m]*f[2];if (!dcmp(f[1]-f[2]) || dcmp(max(max(abs(p[1].x), abs(p[1].y)), max(abs(p[2].x), abs(p[2].y))) - M) > 0)continue;s[m] = p[1]; l[m++] = f[2] - f[1];}}memset(t, n = 0, sizeof(t));for (int i=0; i<m; ++i) {f[0] = 0.; f[1] = l[i]; Vector r(-v[i].x, -v[i].y); int c = 2;for (int j=0; j<m; ++j) if (dcmp(Cross(v[i], v[j]))) {double t = LineInterp(s[i], v[i], s[j], v[j]);if (t > 0. && t < l[i]) f[c++] = t;}sort(f, f+c); c = unique(f, f+c) - f;for (int j=1, a = addPoint(s[i]+v[i]*f[0]), b; j<c; a=b, ++j) {if (dcmp(f[j]-f[j-1]) == 0) {b = a; continue;}g[a][t[a]] = b = addPoint(s[i]+v[i]*f[j]), g[b][t[b]] = a, d[a][t[a]++] = v[i], d[b][t[b]++] = r;}}memset(vis, 0, sizeof(vis)); dfs(0, 0, m = 0);map<pair<int, int>, int> b;for (int i=0; i<m; ++i) for (int j=0; j<e[i]; ++j) b[pair<int, int>(u[i][j], u[i][j+1])] = i;for (int i=0; i<m; ++i) {for (int j=t[i]=0; j<e[i]; ++j) {pair<int, int> p(u[i][j+1], u[i][j]);if (b.count(p)) g[i][t[i]++] = b[p];}sort(g[i], g[i]+t[i]); t[i] = unique(g[i], g[i]+t[i]) - g[i];}while (c--) addW();cout << "Case " << ++kase << ':' << endl;while (q--) cout << query() << endl;
}int main() {ios::sync_with_stdio(false); cin.tie(0); cout.tie(0);s[0] = s[3] = {-M, -M}; s[1] = {M, -M}; s[2] = {-M, M};v[0] = v[2] = {1., 0.}; v[1] = v[3] = {0., 1.}; l[0] = l[1] = l[2] = l[3] = 2.*M;while (cin >> n >> c >> q && n) solve();return 0;
}