Open CASCADE学习|GeomFill

Open CASCADE学习|GeomFill_Frenet

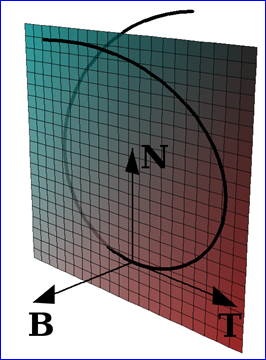
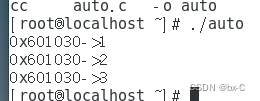
GeomFill_Frenet继承自GeomFill_TrihedronLaw类。GeomFill_Frenet类主要用于实现Frenet标架的计算。Frenet标架是一个沿曲线移动的局部坐标系，它由切向量、法向量和副法向量组成，常用于机器人学、计算机图形学等领域。

class GeomFill_Frenet : public GeomFill_TrihedronLaw
{
public:Standard_EXPORT GeomFill_Frenet();Standard_EXPORT virtual Handle(GeomFill_TrihedronLaw) Copy() const Standard_OVERRIDE;Standard_EXPORT void Init();Standard_EXPORT virtual Standard_Boolean SetCurve (const Handle(Adaptor3d_Curve)& C) Standard_OVERRIDE;Standard_EXPORT virtual Standard_Boolean D0 (const Standard_Real Param, gp_Vec& Tangent, gp_Vec& Normal, gp_Vec& BiNormal) Standard_OVERRIDE;Standard_EXPORT virtual Standard_Boolean D1 (const Standard_Real Param, gp_Vec& Tangent, gp_Vec& DTangent, gp_Vec& Normal, gp_Vec& DNormal, gp_Vec& BiNormal, gp_Vec& DBiNormal) Standard_OVERRIDE;Standard_EXPORT virtual Standard_Boolean D2 (const Standard_Real Param, gp_Vec& Tangent, gp_Vec& DTangent, gp_Vec& D2Tangent, gp_Vec& Normal, gp_Vec& DNormal, gp_Vec& D2Normal, gp_Vec& BiNormal, gp_Vec& DBiNormal, gp_Vec& D2BiNormal) Standard_OVERRIDE;Standard_EXPORT virtual Standard_Integer NbIntervals (const GeomAbs_Shape S) const Standard_OVERRIDE;Standard_EXPORT virtual void Intervals (TColStd_Array1OfReal& T, const GeomAbs_Shape S) const Standard_OVERRIDE;Standard_EXPORT virtual void GetAverageLaw (gp_Vec& ATangent, gp_Vec& ANormal, gp_Vec& ABiNormal) Standard_OVERRIDE;Standard_EXPORT virtual Standard_Boolean IsConstant() const Standard_OVERRIDE;Standard_EXPORT virtual Standard_Boolean IsOnlyBy3dCurve() const Standard_OVERRIDE;DEFINE_STANDARD_RTTIEXT(GeomFill_Frenet,GeomFill_TrihedronLaw)
protected:
private:Standard_EXPORT Standard_Boolean IsSingular (const Standard_Real U, Standard_Integer& Index) const;Standard_EXPORT Standard_Boolean DoSingular (const Standard_Real U, const Standard_Integer Index, gp_Vec& Tangent, gp_Vec& BiNormal, Standard_Integer& n, Standard_Integer& k, Standard_Integer& TFlag, Standard_Integer& BNFlag, Standard_Real& Delta);Standard_EXPORT Standard_Boolean SingularD0 (const Standard_Real Param, const Standard_Integer Index, gp_Vec& Tangent, gp_Vec& Normal, gp_Vec& BiNormal, Standard_Real& Delta);Standard_EXPORT Standard_Boolean SingularD1 (const Standard_Real Param, const Standard_Integer Index, gp_Vec& Tangent, gp_Vec& DTangent, gp_Vec& Normal, gp_Vec& DNormal, gp_Vec& BiNormal, gp_Vec& DBiNormal, Standard_Real& Delta);Standard_EXPORT Standard_Boolean SingularD2 (const Standard_Real Param, const Standard_Integer Index, gp_Vec& Tangent, gp_Vec& DTangent, gp_Vec& D2Tangent, gp_Vec& Normal, gp_Vec& DNormal, gp_Vec& D2Normal, gp_Vec& BiNormal, gp_Vec& DBiNormal, gp_Vec& D2BiNormal, Standard_Real& Delta);Standard_EXPORT Standard_Boolean RotateTrihedron (gp_Vec& Tangent, gp_Vec& Normal, gp_Vec& BiNormal, const gp_Vec& NewTangent) const;gp_Pnt P;Handle(TColStd_HArray1OfReal) mySngl;Handle(TColStd_HArray1OfReal) mySnglLen;Standard_Boolean isSngl;

GeomFill_Frenet()：构造函数，用于初始化GeomFill_Frenet对象。

Copy()：返回当前对象的副本。

Init()：初始化函数，用于初始化GeomFill_Frenet对象的状态。

SetCurve(const Handle(Adaptor3d_Curve)& C)：设置曲线，Adaptor3d_Curve是一个适配器，用于将不同类型的曲线统一到一个接口。

D0, D1, D2函数：这些函数是Frenet标架计算的核心，用于获取曲线在不同阶数下的导数信息。D0：计算给定参数Param处的Frenet标架的零阶导数，即切向量（Tangent）、法向量（Normal）和副法向量（BiNormal）。D1：计算一阶导数，除了切向量（Tangent）外，还包括切向量的导数（DTangent）、法向量的导数（DNormal）和副法向量的导数（DBiNormal）。D2：计算二阶导数，包括切向量的二阶导数（D2Tangent）、法向量的二阶导数（D2Normal）和副法向量的二阶导数（D2BiNormal）。

NbIntervals和Intervals函数：这两个函数用于处理曲线分段的情况，当曲线不是单调或连续时，可能需要将其拆分成多个区间来单独处理。

NbIntervals：返回给定形状S的区间数量。Intervals：将形状S的区间填充到数组T中。

GetAverageLaw函数：计算Frenet标架的平均值，包括平均切向量（ATangent）、平均法向量（ANormal）和平均副法向量（ABiNormal）。

IsConstant和IsOnlyBy3dCurve函数：这两个函数用于查询Frenet标架的某些特定属性。IsConstant：检查Frenet标架是否是常数，即是否不随参数变化。IsOnlyBy3dCurve：检查Frenet标架是否仅由3D曲线决定，与其他因素无关。

RTTI宏：DEFINE_STANDARD_RTTIEXT(GeomFill_Frenet,GeomFill_TrihedronLaw)：定义运行时类型信息，使得在运行时可以准确识别GeomFill_Frenet对象的类型。

受保护和私有成员函数：IsSingular：检查给定参数U处的Frenet标架是否奇异（即无法定义或计算不稳定）。DoSingular：处理奇异点的情况，计算奇异点处的Frenet标架及其相关属性。SingularD0：可能是处理奇异点处的零阶导数的函数，但代码片段不完整，无法提供完整解释。

以下代码的主要目的是生成一组随机点，拟合一个B样条曲线，对曲线进行采样，并计算每个采样点的Frenet标架。

#include <TColgp_Array1OfPnt.hxx>
#include <math_BullardGenerator.hxx>
#include <GCPnts_UniformAbscissa.hxx>
#include <GCPnts_UniformDeflection.hxx>
#include <GCPnts_TangentialDeflection.hxx>
#include <GCPnts_QuasiUniformDeflection.hxx>
#include <Geom_BSplineCurve.hxx>
#include <GeomAPI_PointsToBSpline.hxx>
#include <GeomFill_Fixed.hxx>
#include <GeomFill_Frenet.hxx>
#include <GeomFill_ConstantBiNormal.hxx>
#include <GeomFill_CorrectedFrenet.hxx>
#include <GeomFill_Darboux.hxx>
#include <GeomFill_DiscreteTrihedron.hxx>
#include <GeomFill_GuideTrihedronAC.hxx>
#include <GeomFill_GuideTrihedronPlan.hxx>
#include <BRepBuilderAPI_MakeEdge.hxx>
#include <BRepTools.hxx>
#include <GeomAdaptor_Curve.hxx>void test()
{TColgp_Array1OfPnt aPoints(1, 6);math_BullardGenerator aBullardGenerator;for (Standard_Integer i = aPoints.Lower(); i <= aPoints.Upper(); ++i){Standard_Real aX = aBullardGenerator.NextReal() * 50.0;Standard_Real aY = aBullardGenerator.NextReal() * 50.0;Standard_Real aZ = aBullardGenerator.NextReal() * 50.0;aPoints.SetValue(i, gp_Pnt(aX, aY, aZ));}GeomAPI_PointsToBSpline aBSplineFitter(aPoints);if (!aBSplineFitter.IsDone()){return;}Handle(Geom_BSplineCurve) aBSplineCurve = aBSplineFitter.Curve();Handle(GeomAdaptor_Curve) aCurveAdaptor = new GeomAdaptor_Curve(aBSplineCurve);BRepBuilderAPI_MakeEdge anEdgeMaker(aBSplineCurve); Handle(GeomFill_Frenet) aFrenet = new GeomFill_Frenet();aFrenet->SetCurve(aCurveAdaptor); GeomAdaptor_Curve GAC(aBSplineCurve);GCPnts_UniformAbscissa aPointSampler(GAC, 5.0);for (Standard_Integer i = 1; i <= aPointSampler.NbPoints(); ++i){Standard_Real aParam = aPointSampler.Parameter(i);gp_Pnt aP = aCurveAdaptor->Value(aParam);gp_Vec aT;gp_Vec aN;gp_Vec aB;aFrenet->D0(aParam, aT, aN, aB);std::cout << "vtrihedron vt" << i << " -origin " << aP.X() << " " << aP.Y() << " " << aP.Z() << " "<< " -zaxis " << aB.X() << " " << aB.Y() << " " << aB.Z() << " "<< " -xaxis " << aT.X() << " " << aT.Y() << " " << aT.Z() << std::endl;std::cout << "vtrihedron vt" << i << " -labels xaxis T 1" << std::endl;std::cout << "vtrihedron vt" << i << " -labels yaxis N 1" << std::endl;std::cout << "vtrihedron vt" << i << " -labels zaxis B 1" << std::endl;std::cout << "vsize vt" << i << " 2" << std::endl;}
}
int main(int argc, char* argv[])
{test();return 0;
}

部分输出：

vtrihedron vt1 -origin 14.3321 39.5759 36.4378 -zaxis 0.603904 -0.215816 -0.767283 -xaxis 0.245889 -0.865249 0.436902

vtrihedron vt1 -labels xaxis T 1

vtrihedron vt1 -labels yaxis N 1

vtrihedron vt1 -labels zaxis B 1

vsize vt1 2

vtrihedron vt2 -origin 15.5482 35.2417 38.614 -zaxis 0.606185 -0.214462 -0.765862 -xaxis 0.240416 -0.868495 0.433493

vtrihedron vt2 -labels xaxis T 1

vtrihedron vt2 -labels yaxis N 1

vtrihedron vt2 -labels zaxis B 1

vsize vt2 2

vtrihedron vt3 -origin 16.7352 30.8904 40.772 -zaxis 0.608637 -0.213027 -0.764317 -xaxis 0.234266 -0.872095 0.429616