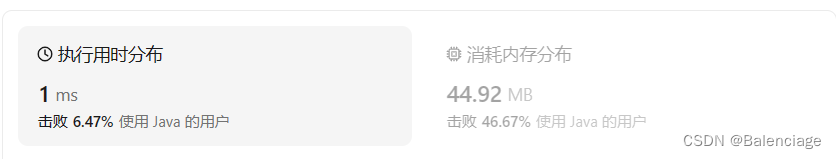
目录

题目：

题解：

方法一：

方法二：

---

题目：

给你一个按照非递减顺序排列的整数数组 nums，和一个目标值 target。请你找出给定目标值在数组中的开始位置和结束位置。

如果数组中不存在目标值 target，返回 [-1, -1]。

你必须设计并实现时间复杂度为 O(log n) 的算法解决此问题。

示例 1：

输入：nums = [5,7,7,8,8,10], target = 8
输出：[3,4]

示例 2：

输入：nums = [5,7,7,8,8,10], target = 6
输出：[-1,-1]

示例 3：

输入：nums = [], target = 0
输出：[-1,-1]

提示：

• 0 <= nums.length <= 105
• -109 <= nums[i] <= 109
• nums 是一个非递减数组
• -109 <= target <= 109

题解：

方法一：

直接遍历num数组，将数组的元素存进list中，然后利用List自带的方法indexOf返回第一次出现target的下标，lastIndexOf返回最后一次出现的target的下标。

class Solution {public int[] searchRange(int[] nums, int target) {int[] res = new int[2];List<Integer> list = new ArrayList<>();for (int num : nums) {list.add(num);}int last = list.lastIndexOf(target);int first = list.indexOf(target);res[0] = first;res[1] = last;return res;}
}

这种解法所需的时间比较长

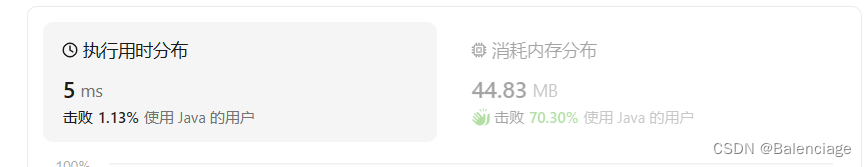
方法二（双指针）：

使用双指针，从数组两边开始遍历数组，直到两者都找到为止。

class Solution {public int[] searchRange(int[] nums, int target) {int[] res = {-1, -1};if (nums.length == 1 && nums[0] == target) {return new int[]{0, 0};}boolean f = false;boolean l = false;int L = 0;int R = nums.length - 1;while (!(f && l) && L <= nums.length - 1 && R >= 0) {if (nums[L] == target && !f) {res[0] = L;f = true;}if (nums[R] == target && !l) {res[1] = R;l = true;}L++;R--;}return res;}
}