Day28:回溯法 491.递增子序列 46.全排列 47.全排列 II 332.重新安排行程 51. N皇后 37. 解数独蓝桥杯与或异或

491. 非递减子序列

给你一个整数数组 nums ，找出并返回所有该数组中不同的递增子序列，递增子序列中 至少有两个元素 。你可以按 任意顺序 返回答案。

数组中可能含有重复元素，如出现两个整数相等，也可以视作递增序列的一种特殊情况。

示例 1：

输入：nums = [4,6,7,7]
输出：[[4,6],[4,6,7],[4,6,7,7],[4,7],[4,7,7],[6,7],[6,7,7],[7,7]]

示例 2：

输入：nums = [4,4,3,2,1]
输出：[[4,4]]

提示：

1 <= nums.length <= 15
-100 <= nums[i] <= 100

思路:

本题和求子集相似,要求取有序子集,但子集不能重复,所以就不能将原数组排序后取子集实现去重,本题也是同一父节点下的同层上使用过的元素就不能再使用了

递归三部曲:

1.确定返回值和参数的类型

定义两个全局变量

List<List<Integer>> result;记录所有结点
List<Integer> node;记录当前结点

返回值为void,在递归过程中更高全局变量

2.确定递归结束条件

本题其实类似求子集问题，也是要遍历树形结构找每一个节点,但本题收集结果有所不同，题目要求递增子序列大小至少为2

if(startIndex>nums.length)
return;
这行代码可以省略,因为startIndex在递归过程中会加1,不会无限递归下去(进不去for循环)

3.确定单层逻辑

同一父节点下的同层上使用过的元素就不能再使用了,我们使用set对本层元素去重,注意set只负责本层,所以进入下一层需要清空

将个数大于1的node加入到result

对本层元素去重

递归找到所有node

代码参考:

class Solution {List<List<Integer>> result = new  LinkedList<>();List<Integer> path = new LinkedList<>(); public List<List<Integer>> findSubsequences(int[] nums) {backTracking(nums,0);return result;}public void backTracking(int[] nums,int startIndex){if(path.size()>1)result.add(new LinkedList<>(path));if(startIndex == nums.length){return;}Set<Integer> hashSet= new HashSet<>();for(int i=startIndex;i<nums.length;i++){if(!path.isEmpty()&&nums[i]<path.get(path.size()-1)||hashSet.contains(nums[i])){continue;}     hashSet.add(nums[i]);path.add(nums[i]);backTracking(nums,i+1);path.removeLast();}}}

46. 全排列

给定一个不含重复数字的数组 nums ，返回其 所有可能的全排列 。你可以 按任意顺序 返回答案。

示例 1：

输入：nums = [1,2,3]
输出：[[1,2,3],[1,3,2],[2,1,3],[2,3,1],[3,1,2],[3,2,1]]

示例 2：

输入：nums = [0,1]
输出：[[0,1],[1,0]]

示例 3：

输入：nums = [1]
输出：[[1]]

提示：

1 <= nums.length <= 6
-10 <= nums[i] <= 10
nums 中的所有整数 互不相同

思路:

思路:用回溯法收集叶节点,用uesd数组记录路径上哪些数字已经使用,实现路径去重,这里就不是树层去重了

递归三部曲:

1.确定返回值和参数的类型

定义两个全局变量List<List<Integer>> result=new ArrayList<>();记录结果集
List<Integer>path=new LinkedList<>();记录递归路径,也就是全排列的过程

返回值为void,传入需要排列的数组nums,和数组used(记录递归过程中哪些数被用掉了,实现路径去重)

2.确定递归结束条件

if(nums.length==path.size()){
result.add(new ArrayList<>(path));
return;
}

nums.length==path.size()时到达叶节点,也就是找到一组全排列了

3.确定单层逻辑

将路径上没用过的数加入路径

代码:

class Solution {List<List<Integer>>  result = new LinkedList<>();List<Integer> path = new LinkedList<>();public List<List<Integer>> permute(int[] nums) {boolean[] used = new boolean[nums.length];backTracking(nums,used);return result;}public void backTracking(int[] nums,boolean[] used){if(path.size()==nums.length){result.add(new LinkedList(path));}for(int i=0;i<nums.length;i++){if(used[i]){continue;}path.add(nums[i]);used[i]=true;backTracking(nums,used);used[i]=false;path.removeLast();}}
}

47. 全排列 II

给定一个可包含重复数字的序列 nums ，按任意顺序 返回所有不重复的全排列。

示例 1：

输入：nums = [1,1,2]
输出：
[[1,1,2],[1,2,1],[2,1,1]]

示例 2：

输入：nums = [1,2,3]
输出：[[1,2,3],[1,3,2],[2,1,3],[2,3,1],[3,1,2],[3,2,1]]

提示：

1 <= nums.length <= 8
-10 <= nums[i] <= 10

思路:本题与上一题区别在于,序列nums包含重复数字, 本题该如何去重呢?

先将数组排序,让相同的数字排在一起,实现树层去重,利用used数组实现路径去重

i>0&&nums[i-1]==nums[i]&&!used[i-1]:说明在同一层使用了,注意这里的used[i-1]必须为false,只有这样两个相同的数才会出现在同一层

used[i]:说明该数在路径中已经使用了,不能再使用了

代码:

if(i>0&&nums[i-1]==nums[i]&&!used[i-1]||used[i]){ continue; }

代码参考:

class Solution {List<List<Integer>> result= new LinkedList<>();List<Integer> path= new LinkedList<>();public List<List<Integer>> permuteUnique(int[] nums) {boolean[] used = new boolean[nums.length];Arrays.sort(nums);backTracking(nums,used);return result;}public void backTracking(int[] nums,boolean[] used){if(nums.length==path.size()){result.add(new LinkedList<>(path));return;}for(int i=0;i<nums.length;i++){if(i>0&&nums[i-1]==nums[i]&&!used[i-1]||used[i]){continue;}used[i]=true;path.add(nums[i]);backTracking(nums,used);used[i]=false;path.removeLast();}}
}

332. 重新安排行程

给你一份航线列表 tickets ，其中 tickets[i] = [fromi, toi] 表示飞机出发和降落的机场地点。请你对该行程进行重新规划排序。

所有这些机票都属于一个从 JFK（肯尼迪国际机场）出发的先生，所以该行程必须从 JFK 开始。如果存在多种有效的行程，请你按字典排序返回最小的行程组合。

例如，行程 ["JFK", "LGA"] 与 ["JFK", "LGB"] 相比就更小，排序更靠前。

假定所有机票至少存在一种合理的行程。且所有的机票必须都用一次且只能用一次。

示例 1：

输入：tickets = [["MUC","LHR"],["JFK","MUC"],["SFO","SJC"],["LHR","SFO"]]
输出：["JFK","MUC","LHR","SFO","SJC"]

示例 2：

输入：tickets = [["JFK","SFO"],["JFK","ATL"],["SFO","ATL"],["ATL","JFK"],["ATL","SFO"]]
输出：["JFK","ATL","JFK","SFO","ATL","SFO"]
解释：另一种有效的行程是 ["JFK","SFO","ATL","JFK","ATL","SFO"] ，但是它字典排序更大更靠后。

提示：

1 <= tickets.length <= 300
tickets[i].length == 2
fromi.length == 3
toi.length == 3
fromi 和 toi 由大写英文字母组成
fromi != toi

思路:

本题用回溯法,遍历所有的票的使用顺序,由于所有机票至少存在一种合理的行程,我们先将票按照起始位置的开头字母小的排序,递归过程中找到其中一种合理行程就返回,该行程一定是字典排序更小的行程。

递归三部曲:

1.确定返回值和参数的类型

使用全局变量保存结果 ,参数传入所有的票(List<List<String>> tickets),和每张票的使用情况(used[])

由于只取第一个结果,所有返回值类型设置为boolean类型,当找到第一个结果时,就返回true

List<String> path=new LinkedList<>(); List<String> result; boolean travel(List<List<String>> tickets,boolean used[]) 2.确定递归结束条件

当记录路径的数组的长度==票的长度+1,说明合理用完了所有票,找到了合理旅游路径,结束递归

if(path.size()==tickets.size()+1){ result=new ArrayList<>(path); return true; } 3.单层递归逻辑

递归找到所有方案

for(int i=0;i<tickets.size();i++){ if(!used[i]&&path.get(path.size()-1).equals(tickets.get(i).get(0))){ path.add(tickets.get(i).get(1)); used[i]=true; //找到第一个方案,结束递归 if( travel(tickets,used))return true; path.remove(path.size()-1); used[i]=false; } } return false; 总体代码:

class Solution {List<String> path=new LinkedList<>();List<String> result;public List<String> findItinerary(List<List<String>> tickets) {boolean[] used=new boolean[tickets.size()];//给票排序Collections.sort(tickets,(a,b)->a.get(1).compareTo(b.get(1)));path.add("JFK");travel(tickets,used);return result;}boolean travel(List<List<String>> tickets,boolean used[]){if(path.size()==tickets.size()+1){result=new ArrayList<>(path);return true;}for(int i=0;i<tickets.size();i++){if(!used[i]&&path.get(path.size()-1).equals(tickets.get(i).get(0))){path.add(tickets.get(i).get(1));used[i]=true;//找到第一个方案,结束递归if( travel(tickets,used))return true;path.remove(path.size()-1);used[i]=false;}}return false;}
}

使用本方法因为排序的原因会出现超时

改进方法:

用Map<出发机场, Map<到达机场, 航班次数>> map来记录车票,Map<到达机场, 航班次数>为升序TreeMap

class Solution {private Deque<String> path = new LinkedList<>();//双端队列,用来存储飞行路径private Map<String,Map<String,Integer>> map = new HashMap<>();//hashmap存储一个其他到其他地方的票数public List<String> findItinerary(List<List<String>> tickets) {for(int i=0;i<tickets.size();i++){//统计每个出发地到目的地的票数if(map.containsKey(tickets.get(i).get(0))){Map<String,Integer> temp= map.get(tickets.get(i).get(0));//获取目的地们与其对应的票数temp.put(tickets.get(i).get(1),temp.getOrDefault(tickets.get(i).get(1),0)+1);map.put(tickets.get(i).get(0),temp);}else{Map temp = new TreeMap<>();temp.put(tickets.get(i).get(1),1);map.put(tickets.get(i).get(0),temp);}}path.add("JFK");backTracking(tickets.size());return new LinkedList( path);}public boolean backTracking(int tickets){if(path.size()==tickets+1){return true;}String start =  path.getLast();//取出同一出发地点的各个机票的目的地和对应的票数if(map.get(start)!=null)for(Map.Entry<String, Integer> target : map.get(start).entrySet()){int  times= target.getValue();if(times>0){path.add(target.getKey());target.setValue(times-1);if( backTracking(tickets)){return true;}path.removeLast();target.setValue(times);}}return false;}
}

51. N 皇后

按照国际象棋的规则，皇后可以攻击与之处在同一行或同一列或同一斜线上的棋子。

n 皇后问题 研究的是如何将 n 个皇后放置在 n×n 的棋盘上，并且使皇后彼此之间不能相互攻击。

给你一个整数 n ，返回所有不同的 n 皇后问题 的解决方案。

每一种解法包含一个不同的 n 皇后问题 的棋子放置方案，该方案中 'Q' 和 '.' 分别代表了皇后和空位。

示例 1：

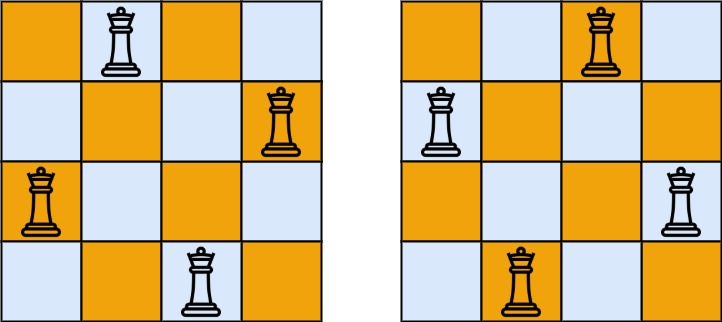
输入：n = 4
输出：[[".Q..","...Q","Q...","..Q."],["..Q.","Q...","...Q",".Q.."]]
解释：如上图所示，4 皇后问题存在两个不同的解法。

示例 2：

输入：n = 1
输出：[["Q"]]

提示：

1 <= n <= 9

皇后们的约束条件：

不能同行
不能同列
不能同斜线

思路:用回溯法,遍历出所有可以放置的可能性

递归三部曲:

1.确定返回值和参数类型

要把所有能摆放的位置都得出,用全局变量public List<List<String>> result=new ArrayList<>();记录所有合理的棋盘,返回值为void,传入棋盘(char[][] chessboard),要放置的皇后在哪一行(int row),棋盘的宽度(n);

public void backTracking(int n,int row,char[][] chessboard)

2.确定递归结束条件

当row==n时,说明所有行都放置了皇后,找到了一种合理放法,将该棋盘存入result中,递归结束

if(row==n){
List<String> temp= array2List(chessboard);
result.add(temp);
return;
}

3.确定单层递归逻辑

遍历该行的每一个位置并检查其合理性,如果合理,进入下一行棋盘的摆放

for(int i=0;i<n;i++){
//如果当前位置合法,就递归放下一行
if(isVaild(chessboard,row,i,n)){
chessboard[row][i]='Q';
backTracking(n,row+1,chessboard);
chessboard[row][i]='.';
}
}

class Solution {List<List<String>> result = new LinkedList<>();List<String> board = new LinkedList<>();public List<List<String>> solveNQueens(int n) {char[][] chessboard = new char[n][n];for(char[] c:chessboard){Arrays.fill(c,'.');}backTracking(n,0,chessboard);return result;}public void backTracking(int n,int row,char[][] chessboard){if(row==chessboard.length){List<String> temp= array2List(chessboard);result.add(temp);return;}for(int i=0;i<chessboard.length;i++){if(isVaild(n,chessboard,row,i)){chessboard[row][i]='Q';backTracking(n,row+1,chessboard);chessboard[row][i]='.';}}}//将数组转为listList<String> array2List(char[][] chessboard){List<String> result=new ArrayList<>();for(int i=0;i<chessboard.length;i++){StringBuilder temp=new StringBuilder();for(int j=0;j<chessboard[0].length;j++){temp.append(chessboard[i][j]);}result.add(temp.toString());}return result;}public boolean isVaild(int n,char[][] chessboard,int row,int col){//检查列for(int i=row;i>=0;i--){if(chessboard[i][col]=='Q'){return false;}}//45°角for(int i=row-1,j=col+1;i>=0&&j<n;i--,j++){if(chessboard[i][j]=='Q'){return false;}}//135°for(int i=row-1,j=col-1;i>=0&&j>=0;i--,j--){if(chessboard[i][j]=='Q'){return false;}}return true;}
}