一劳永逸 维基百科公式不显示怎么办？

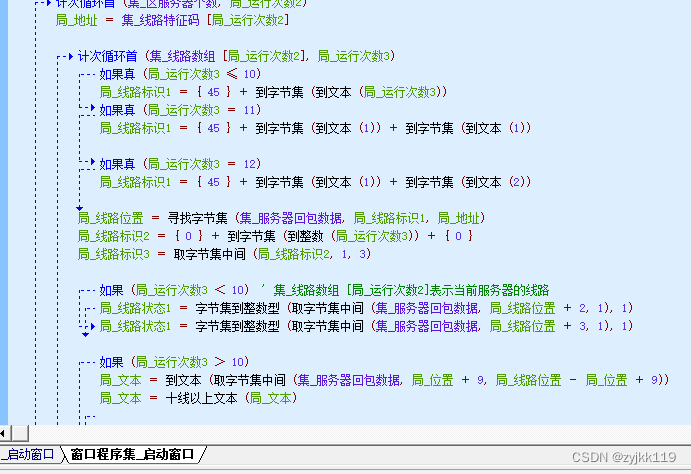
1. 注册1个wiki账号，
2. 参数设置,
3. 显示选项卡，最后的PNG图片点上；
   成功；

- 有人说改http，为https协议就可以，但是我的本来就是https协议，同样显示不了图片，https://en.wikipedia.org/wiki/Cholesky_decomposition -

2 用Latex源+浏览器插件

1 上述图片，不选png图片，选第二个 Latex源
2 chrom是Math Anywhere，一键转换；很powerful

推荐勾选beta版本，测试功能 翻译很给力，中文wiki和英文的资料不是一个量级，但看英文有时候又很痛苦。

3 懒得写latex公式的一条捷径，借鉴wiki！

不想注册账号？ 拷贝wiki原文，放入latex解析功能的文档中；比如CSDN （$前后空格全部替换掉)；如下：（或者前面装mathanywhere插件时候，点击关掉，拷贝，即可）ARicianfadingchannelcanbedescribedbytwoparameters:$K$and$\Omega$.[1]$K$istheratiobetweenthepowerinthedirectpathandthepowerintheother,scattered,paths.[2]$\Omega$isthetotalpowerfrombothpaths($\Omega =\nu ^{2}+2\sigma ^{2}$), and acts as a scaling factor to the distribution.

The received signal amplitude (not the received signal power)$R$is then Rice distributed with parameters${\nu }^2=\frac{K}{1+K}\Omega$and${\sigma }^2=\frac{\Omega }{2(1+K)}$.[3] The resulting PDF then is:

$f(x)=\frac{2(K+1)x}{\Omega }\exp \left(-K-\frac{(K+1)x^2}{\Omega }\right)I_0\left(2\sqrt{\frac{K(K+1)}{\Omega }}x\right),$
where$I_0(\cdot )$is the 0th order modified Bessel function of the first kind.

latex写论文的小伙伴有福了，果断抄抄，高大上 糙猛快，既能装逼又能深入理解数学大厦；