文章目录
- 57. 插入区间
- 823. 带因子的二叉树
- 解法——递推
- 1654. 到家的最少跳跃次数(BFS,🚹最远距离上界的证明)
- 1761. 一个图中连通三元组的最小度数
- 2240. 买钢笔和铅笔的方案数
- 解法1——完全背包
- 解法2——枚举买了几支钢笔(推荐解法)
- 2511. 最多可以摧毁的敌人城堡数目
- 解法——一次遍历
- 1921. 消灭怪物的最大数量(贪心)
57. 插入区间
https://leetcode.cn/problems/insert-interval/

提示:
0 <= intervals.length <= 10^4
intervals[i].length == 2
0 <= intervals[i][0] <= intervals[i][1] <= 10^5
intervals 根据 intervals[i][0] 按 升序 排列
newInterval.length == 2
0 <= newInterval[0] <= newInterval[1] <= 10^5
当前区间与要加入的新区间之间的关系只有两种可能:相交或者不相交。
如果相交,将两者结合即可。
如果不相交,判断新加入的区间是否在当前区间之前,如果是,就先加入答案。
遍历一遍之后,如果没有处理过新加入的区间,就说明新区间应该加在最后。
class Solution {public int[][] insert(int[][] intervals, int[] newInterval) {if (intervals.length == 0) return new int[][]{newInterval};List<int[]> ans = new ArrayList<>();int s = newInterval[0], e = newInterval[1];boolean f = false;for (int[] interval: intervals) {int a = interval[0], b = interval[1];// 和 newInterval 相交if (b >= s && a <= e) {f = true;a = Math.min(a, s);b = Math.max(b, e);}// 不相交 newInterval 在此区间之前if (a > e) {if (ans.size() == 0 || ans.get(ans.size() - 1)[1] < s) {ans.add(newInterval);f = true;}}if (ans.size() == 0 || ans.get(ans.size() - 1)[1] < a) ans.add(new int[]{a, b});else ans.get(ans.size() - 1)[1] = b;}// 如果没有处理过 newIntervalif (!f) ans.add(newInterval);return ans.toArray(new int[ans.size()][2]);}
}
823. 带因子的二叉树
https://leetcode.cn/problems/binary-trees-with-factors/

提示:
1 <= arr.length <= 1000
2 <= arr[i] <= 10^9
arr 中的所有值 互不相同
解法——递推
将元素排序之后,从小到大依次处理各个数值作为根节点时的二叉树数量。
class Solution {final long MOD = (long)1e9 + 7;public int numFactoredBinaryTrees(int[] arr) {Arrays.sort(arr);int n = arr.length;long ans = 0;Map<Integer, Long> m = new HashMap<>(); // 存储每个数字作为根节点时的二叉树数量for (int i = 0; i < n; ++i) {long cnt = 1;for (int j = 0; j < i; ++j) { // 枚举儿子节点if (arr[i] % arr[j] == 0 && m.containsKey(arr[i] / arr[j])) {cnt = (cnt + m.get(arr[j]) * m.get(arr[i] / arr[j])) % MOD;}}ans = (ans + cnt) % MOD;m.put(arr[i], cnt);}return (int)ans;}
}
1654. 到家的最少跳跃次数(BFS,🚹最远距离上界的证明)
https://leetcode.cn/problems/minimum-jumps-to-reach-home/description/

提示:
1 <= forbidden.length <= 1000
1 <= a, b, forbidden[i] <= 2000
0 <= x <= 2000
forbidden 中所有位置互不相同。
位置 x 不在 forbidden 中。
可以证明,一定可以在[0, max(f + a + b, x + b)] 的下标范围内找到最优解,其中 f 是最远进制点的坐标。
因为 f, a, b, x <= 2000,故搜索范围不会超过 6000。(证明略,我也没整明白)
用 bfs 计算最短路。
class Solution {public int minimumJumps(int[] forbidden, int a, int b, int x) {Set<Integer> s = new HashSet<>();for (int v: forbidden) s.add(v);Deque<int[]> q = new ArrayDeque<>();q.offer(new int[]{0, 1}); // 将起点放入队列final int N = 6000;boolean[][] vis = new boolean[N][2];vis[0][1] = true;for (int ans = 0; !q.isEmpty(); ++ans) {for (int t = q.size(); t > 0; --t) {int[] p = q.poll();int i = p[0], k = p[1];// 到达了目的地,直接返回答案if (i == x) return ans;List<int[]> nxt = new ArrayList<>();nxt.add(new int[]{i + a, 1});// 如果上一步 不是向后跳,那么这一步可以向后跳if ((k & 1) == 1) nxt.add(new int[]{i - b, 0});for (int[] e: nxt) {int j = e[0];k = e[1];if (j >= 0 && j < N && !s.contains(j) && !vis[j][k]) {q.offer(new int[]{j, k});vis[j][k] = true;}}}}return -1;}
}
1761. 一个图中连通三元组的最小度数
https://leetcode.cn/problems/minimum-degree-of-a-connected-trio-in-a-graph/

提示:
2 <= n <= 400
edges[i].length == 2
1 <= edges.length <= n * (n-1) / 2
1 <= ui, vi <= n
ui != vi
图中没有重复的边。
构造邻接表,枚举每一个三元组。
class Solution {public int minTrioDegree(int n, int[][] edges) {int[] cnt = new int[n + 1];int[][] isC = new int[n + 1][n + 1];for (int[] edge: edges) {int x = edge[0], y = edge[1];isC[x][y] = 1;isC[y][x] = 1;cnt[x]++;cnt[y]++;}int ans = Integer.MAX_VALUE;for (int i = 1; i <= n; ++i) {for (int j = i + 1; j <= n; ++j) {if (isC[i][j] == 0) continue;for (int k = j + 1; k <= n; ++k) {if (isC[j][k] == 0 || isC[i][k] == 0) continue;ans = Math.min(ans, cnt[i] + cnt[j] + cnt[k] - 6);}}}return ans != Integer.MAX_VALUE? ans: -1;}
}
2240. 买钢笔和铅笔的方案数
https://leetcode.cn/problems/number-of-ways-to-buy-pens-and-pencils/

提示:
1 <= total, cost1, cost2 <= 10^6
解法1——完全背包
套用完全背包模板,对dp数组求和即可。
class Solution {public long waysToBuyPensPencils(int total, int cost1, int cost2) {long[] dp = new long[total + 1];dp[0] = 1;for (int j = cost1; j <= total; ++j) dp[j] += dp[j - cost1];for (int j = cost2; j <= total; ++j) dp[j] += dp[j - cost2];long ans = 0;for (long d: dp) ans += d;return ans;}
}
解法2——枚举买了几支钢笔(推荐解法)
通过枚举购买钢笔的数量,计算此时可以购买铅笔的数量,+1即是购买此时数量钢笔时,购买铅笔的方案数。
class Solution {public long waysToBuyPensPencils(int total, int cost1, int cost2) {long ans = 0;for (int i = 0; i * cost1 <= total; ++i) {ans += (total - i * cost1) / cost2 + 1;}return ans;}
}
2511. 最多可以摧毁的敌人城堡数目
https://leetcode.cn/problems/maximum-enemy-forts-that-can-be-captured/?envType=daily-question&envId=2023-09-02

提示:
1 <= forts.length <= 1000
-1 <= forts[i] <= 1
解法——一次遍历
题目要求是计算 1 和 -1 之间的 0 的最大数量。
一次遍历,遍历的过程中记录上一个 1 或 -1 出现的位置即可。
class Solution {public int captureForts(int[] forts) {int lastId = 0, ans = 0;for (int i = 0; i < forts.length; ++i) {if (forts[i] == -1 || forts[i] == 1) {if (forts[i] + forts[lastId] == 0) ans = Math.max(ans, i - lastId - 1);lastId = i;}}return ans;}
}
1921. 消灭怪物的最大数量(贪心)
https://leetcode.cn/problems/eliminate-maximum-number-of-monsters/
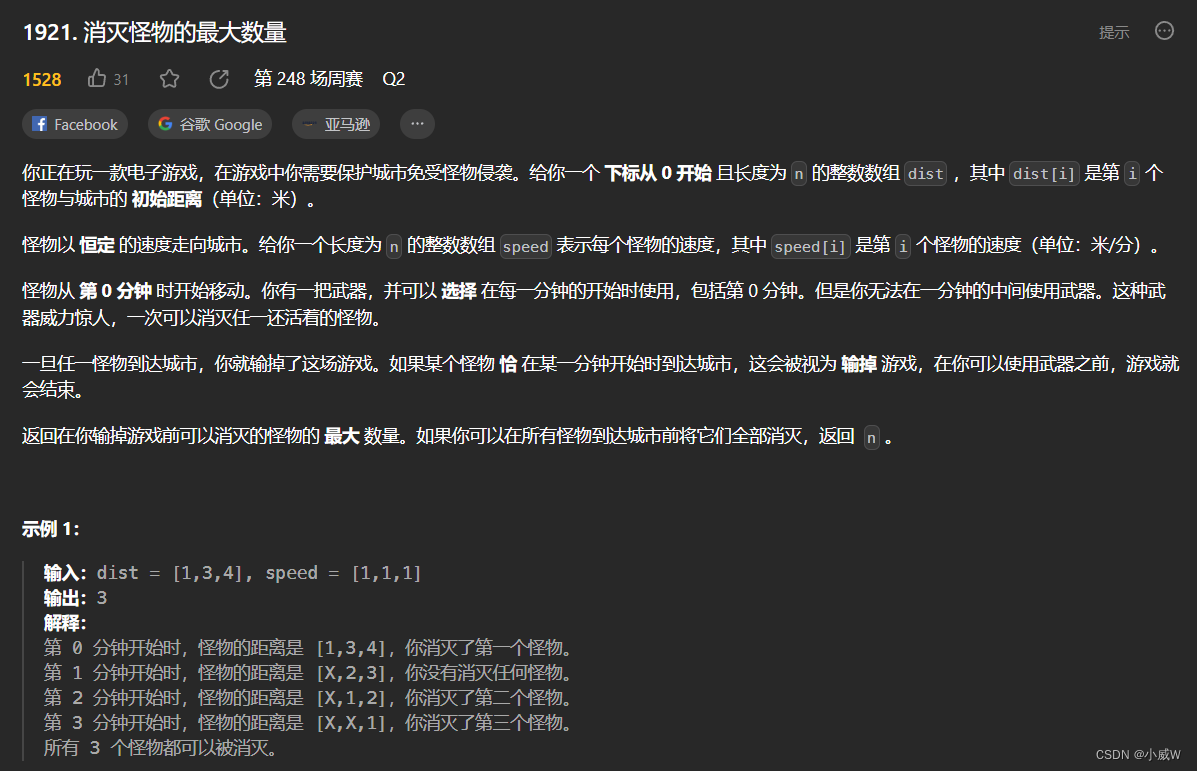
提示:
n == dist.length == speed.length
1 <= n <= 10^5
1 <= dist[i], speed[i] <= 10^5
先计算时间,再按时间排序。
贪心的先消灭快要到达城市的怪兽。
class Solution {public int eliminateMaximum(int[] dist, int[] speed) {int n = dist.length;int[] t = new int[n];for (int i = 0; i < n; ++i) {t[i] = (dist[i] + speed[i] - 1) / speed[i]; // 向上取整}Arrays.sort(t);for (int i = 0; i < n; ++i) {if (t[i] <= i) return i;}return n;}
}