CodeTON Round #7 (Div. 1 + Div. 2) A~E

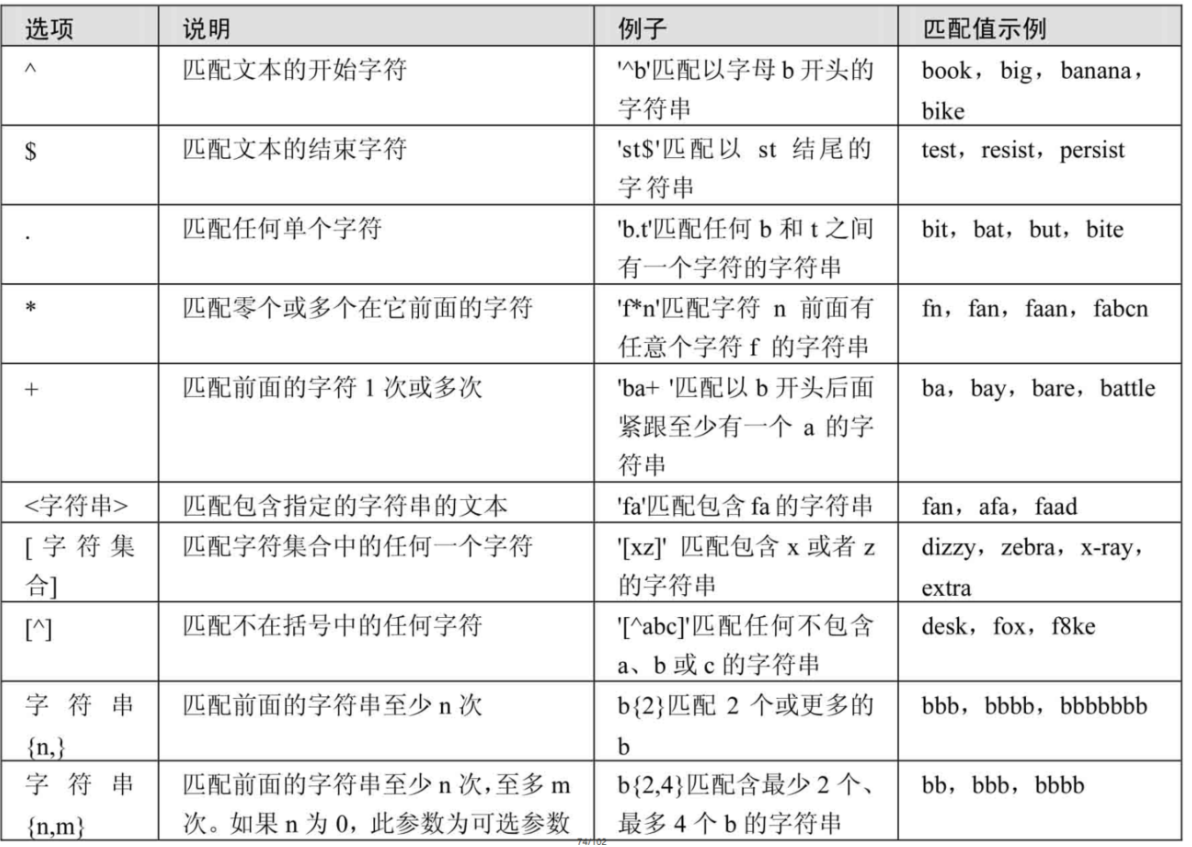
A.jagged Swaps（思维）

题意：

给出一个包含 $n$ 个数字的序列，每次可以选择一个同时大于左右两边相邻的数字，将这个数字与它右边的数字交换，问能否在经过若干次操作后使序列变为升序。

分析：

由于交换只能向后进行，且第一个元素无法向后交换（不存在左边的数字），而其他大的数字均可以通过交换到达自己的位置，因此只需要考虑开始时序列的第一个数字是否为1，如果是1，就是"YES"，否则，就是"NO"。

hint：包含 $n$ 个数字的序列恰好包含 $\sim n$ 中每一个数字。

代码：

#include <bits/stdc++.h>
using namespace std;int a[15];void solve() {int n;cin >> n;for (int i = 1; i <= n; i++) {cin >> a[i];}if (a[1] != 1) {cout << "NO" << endl;} else {cout << "YES" << endl;}
}int main() {int Case;cin >> Case;while (Case--) {solve();}return 0;
}

B.AB Flipping（思维）

题意：

给出一个长度为 $n$ 且仅包含"AB"两种字符的字符串，每次可以选择一个下标 $i$ ,当字符串中第 $i$ 个字符为'A'，且第 $i + 1$ 个字符为'B'，那么可以让第 $i$ 个字符和第 $i + 1$ 个字符交换。

要求每个下标 $i$ 均只能选择一次，问最多可以进行多少次交换。

分析：

当出现连续的'B'前面有一个'A'时，这段连续区间上的'B'均可以向前交换一次，交换次数为这段连续的'B'的长度，而经过一次交换之后，由于每个下标只能被选择一次，那么仅有第一个'B'还能向前交换，可以认为这段连续的'B'交换完成后就只剩下开头这一个'B'了。使用变量维护还能向前交换的'B'的个数，从后往前遍历模拟即可。

代码：

#include <bits/stdc++.h>
using namespace std;string s;void solve() {int n;cin >> n >> s;int cnt = 0, ans = 0;for (int i = n - 1; i >= 0; i--) {if (s[i] == 'B') {cnt++;} else {ans += cnt;cnt = min(cnt, 1);//如果当前没有遇到过B，就让cnt保持在0}}cout << ans << endl;
}int main() {solve();return 0;
}

C.Matching Arrays（贪心）

题意：

给出两个包含 $n$ 个数字的数组 $a$ 和 $b$ ，这两个数组的美丽值为满足 $a_i > b_i$ 的下标 $i$ 的个数。

题目会给出一个数字 $x$ ，问能否对 $b$ 重新排列，使得这两个数组的美丽值等于 $x$ 。

分析：

虽然题目要求只能对 $b$ 重排，但为了便于处理，两个数组都需要排序，同时为了记录数组 $a$ 原本的数字位置，需使用结构体存储数据。

贪心：将 $a$ 中最大的 $x$ 个元素与 $b$ 中最小的 $x$ 个元素按大小次序进行比较，如果这部分元素无法构成 $x$ 的美丽值，由于 $a$ 中剩余元素更小， $b$ 中剩余元素更大，那么无论怎么交换元素，都无法使美丽值增加，此时本题无解。

检查：比较完 $a$ 中最大的 $x$ 个元素与 $b$ 中最小的 $x$ 个元素后，还需要考虑剩余的元素是否还会产生美丽值，同样采用按大小次序依次比较，如果产生美丽值那么同样表示本题无解。

输出：如果可以构造，那么需要根据记录的 $a$ 中每个数字排序前的位置将对应的 $b$ 数组元素输出。

代码：

#include <bits/stdc++.h>
using namespace std;struct Node{int val, id;bool operator < (const Node &o) const {return val < o.val;}
}a[200005], b[200005];int ans[200005];void solve() {int n, x;cin >> n >> x;for (int i = 1; i <= n; i++) {cin >> a[i].val;a[i].id = i;}sort(a + 1, a + 1 + n);for (int i = 1; i <= n; i++) {cin >> b[i].val;b[i].id = i;}sort(b + 1, b + 1 + n);for (int i = 1; i <= x; i++) {if (a[i + n - x].val <= b[i].val) {//a中最大的x个与b中最小的x个对位比较cout << "NO" << endl;return;}ans[a[i + n - x].id] = b[i].val;//将当前b中元素放入对应的a中元素原本所在下标对应的位置上}for (int i = 1; i <= n - x; i++) {if (a[i].val > b[i + x].val) {//剩余的n-x个元素对位比较cout << "NO" << endl;return;}ans[a[i].id] = b[i + x].val;}cout << "YES" << endl;for (int i = 1; i <= n; i++) {cout << ans[i] << ' ';}cout << endl;
}int main() {int Case;cin >> Case;while (Case--) {solve();}return 0;
}

D.Ones and Twos(思维，数据结构)

题意：

给出一个仅包含 $1, 2$ 的数组。

有 $q$ 个询问，询问分以下两种情况：

"1 s"，询问数组中能否找出一个子段和为 $s$
"2 i v"，将数组中第 $i$ 个数字修改为 $v$

分析：

通过分析样例，可以发现以下规律：如果子段的左右端点数字均为1，那么可以组成任意值在 $\sim$ （子段数字之和）以内的数字。

根据以上规律，想要组成尽可能多的数字，那么选择的一定是最左和最右的两个 $1$ 中间的子段（包含端点）。

然后需要根据以下情况进行分类讨论：

数组中存在 $1$ ，这两个 $1$ 之间的子段总和为 $s u m$
- $\le sum$ ,则可以组成
- $\gt sum$ ，分成以下两种情况：
  - $x$ 与 $s u m$ 奇偶性相同，为了尽可能使总和最大，一定会选择将选择的子段向左右扩散，且此时左右元素一定均为2，只要整个数组的数字总和可以达到 $x$ ，那么就能组成 $x$ 。
  - 奇偶性不同时，可以删去子段一侧的 $1$ ，再加上另一侧的 $2$ ，看组成的子段数字总和能否到达 $x$ 。
数组中不存在 $1$ ，那么能组成的只有偶数，且能组成的偶数 $x$ 的值要在数组中数字总和的范围内。

hint：可以通过 $se t$ 对所有 $1$ 所在的位置（下标）进行维护，通过 $* (b e g in ())$ 和 $* (- - e n d ())$ （end()函数返回的是最后一个元素的下一个迭代器，需要通过前自减得到最后一个元素的迭代器）来获得集合中最小和最大的元素。

代码：

#include <bits/stdc++.h>using namespace std;
int n, q, a[100005], cnt;set<int> S;bool check(int x) {if (S.empty()) {//数组中没有1if (x % 2 == 1) return false;if (n * 2 < x) return false;return true;}int first = *S.begin(), last = *(--S.end());//获得最前和最后的1所在的下标int sum = (last - first + 1) * 2 - S.size();//将区间内所有的数视为2，计算出总和，减去1的数量，就是该子段的数字总和if (sum >= x) return true;//被1包围的子段已经能组成x了if (x % 2 == sum % 2) {int add = n - (last - first + 1);//计算出未被加上的2的数量if (sum + add * 2 >= x) return true;} else {int add = max(n - last, first - 1);//计算左右两边最多有多少个2if (sum - 1 + add * 2 >= x) return true;}return false;
}void solve() {S.clear();cin >> n >> q;for (int i = 1; i <= n; i++) {cin >> a[i];if (a[i] == 1) {S.insert(i);cnt++;}}while (q--) {int op;cin >> op;if (op == 1) {int x;cin >> x;if (check(x)) {cout << "YES" << endl;} else {cout << "NO" << endl;}} else {int i, v;cin >> i >> v;if (a[i] == 1) S.erase(i);a[i] = v;if (a[i] == 1) S.insert(i);}}
}int main() {int Case;cin >> Case;while (Case--) {solve();}return 0;
}

E. Permutation Sorting(数据结构)

题意：

给一个大小为 $n$ 的排列 $a$ ，如果满足 $a_i=i$ ，那么称下标 $i$ 是良好的，每一秒，将所有不好的下标 $i$ 取出来作为一个子序列，保证下标 $i$ 是升序的。
假设这个序列为 $s_1-s_k$ ，那么对 $1 - k$ 的每个 $i$ ，使得 $s_{i\%k+1}=s_i$ 。现在对于每一个下标 $i$ ，询问下标 $i$ 第一次变好的时间。

分析：

我们假设 $i$ 与 $a_i$ 之间存在一条边，并且将每组 $i,a_i]$ 看作一个区间，那么对于每一个下标 $i$ ，它的答案为:区间长度 $- 1 -$ 在它之前的并且被它完全包含的区间。
我们可以用二维数点来维护有哪些区间被当前处理的区间包含，将左端点当作 $x$ 坐标，右端点当作 $y$ 坐标，类似于求二维前缀和。考虑到这里是环形数组，可以倍长简化操作。

代码：

#include <bits/stdc++.h>typedef long long LL;
using namespace std;
const int N = 1e6 + 5;
int n;
int a[N];
int ans[N];
int id[N];
int tr[2 * N];
int vis[N];void add(int x, int k) {for (; x <= n; x += (x & -x))tr[x] += k;
}int ask(int x, int res = 0) {for (; x; x -= (x & -x))res += tr[x];return res;
}int qry(int l, int r) {if (l <= r)return ask(r) - ask(l - 1);elsereturn ask(n) - qry(r, l - 1);
}void Init() {for (int i = 1; i <= n; i++)tr[i] = 0;for (int i = 1; i <= n; i++)ans[i] = 0, vis[i] = 0;
}int main() {int t = 1;cin >> t;while (t--) {cin >> n;Init();for (int i = 1; i <= n; i++) {cin >> a[i];id[a[i]] = i;}for (int i = 1; i <= 2 * n; i++) {int x = (i - 1) % n + 1;if (vis[x]) {ans[x] = qry(id[x] + 1, x) + 1;add(id[x], -1);vis[x] = 0;}if (a[x] != x) {add(x, 1);vis[a[x]] = 1;}}for (int i = 1; i <= n; i++) {cout << ans[i] << " ";}cout << endl;}return 0;
}