---

前言

首先给出莱斯衰落信道仿真模型，该模型由直射路径分量和反射路径分量组成，其中反射路径分量由瑞利衰落信道模型构成，该模型可以很方便地建立瑞利衰落信道的仿真。给出莱斯衰落信道的MATLAB仿真代码，并对比了莱斯衰落信道和瑞利衰落信道，揭示了两者之间的内在关系。

---

一、莱斯衰落信道仿真模型

根据《（10）MATLAB莱斯（Rician）衰落信道仿真1》知道，在莱斯衰落信道中，接收信号为直射路径信号和反射路径信号之和，而莱斯因子K正是直射路径与反射路径信号的相对功率。

若K>>1，则信道趋近于直射路径（高斯信道）；若K<<1，则信道趋近于瑞利衰落。

在莱斯衰落信道仿真模型中，一般使用直射路径部分和瑞利衰落部分是分开的方法，仿真模型如下图所示：

其中，

由图1可写出莱斯衰落信道模型的表示式：

下面使用该仿真模型，给出莱斯衰落信道的MATLAB仿真代码。

二、仿真代码与结果

1.仿真代码

首先，建立瑞利衰落信道仿真函数：

function Rayleigh_ch = Rayleigh_channel(nSamples)
% Rayleigh Channel Model
% Input : nSamples    - number of channel samples
% Output: Rayleigh_ch - complex channel vectorsigma = sqrt(0.5);Rayleigh_ch = sigma*(randn(1,nSamples) + 1j*randn(1,nSamples));
end

注：该代码详细说明请参考：（9）MATLAB瑞利衰落信道仿真2

其次，使用瑞利衰落信道函数，建立莱斯衰落信道仿真函数：

function Rician_ch = Rician_channel(K_dB, nSamples)
% Rician Channel Model
% Input : K_dB      - K factor in dB
%         nSamples  - number of channel  samples
% Output: Rician_ch - complex channel vector
K = 10^(K_dB/10);
Rician_ch = sqrt(K/(K+1)) + sqrt(1/(K+1))*Rayleigh_channel(nSamples);

最后，给出莱斯衰落信道仿真模型：

close all
clear all
clcsamples_number = 1e5;
bins_number = 50;                                      % number of bins in the histogram% Rayleigh channel model
Rayleigh_ch = Rayleigh_channel(samples_number);[elements_number,x] = hist(abs(Rayleigh_ch),bins_number);
px = elements_number/samples_number/mean(diff(x));
figure()
plot(x,px,'r-','LineWidth',1.5)
hold on
grid on% Rician channel model
K_dB = [-50, 15];                                       % K in dB
Rician_ch = zeros(2,samples_number);
color = ['b','m'];
line = ['-','--'];
marker = ['o','*'];
for i=1:length(K_dB)Rician_ch(i,:) = Rician_channel(K_dB(i),samples_number);[elements_number,x] = hist(abs(Rician_ch(i,:)),bins_number);px = elements_number/samples_number/mean(diff(x));plot(x, px, [color(i),line(i),marker(i)],'LineWidth',1.5);
endtitle('The PDF of Rayleigh fading channel and Rician fading channel')
xlabel('x')
ylabel('Occurance of x: px')                            % Occurance rate
legend('Rayleigh','Rician, K=-50dB','Rician, K=15dB')

2.仿真结果画图

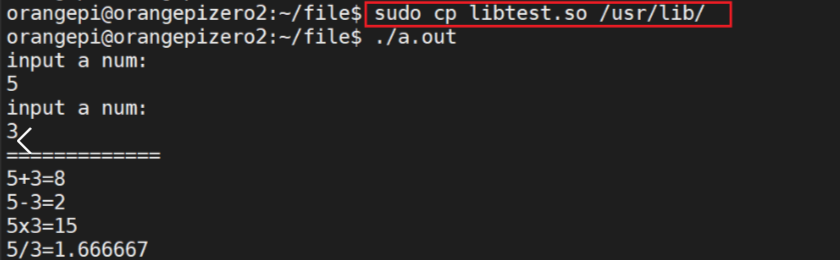
仿真代码运行结果画图如下：

从仿真图中可以看到，若K>>1，则莱斯衰落信道趋近于高斯信道；若K<<1，则信道趋近于瑞利衰落信道。该仿真结果与理论分析一致。

三、后续：

下一篇文章将会给出莱斯随机变量的概率密度函数的MATLAB代码。

四、参考文献：

Michel C. Jeruchim, Philip Balaban, and K. Sam Shanmugan , Simulation of Communication Systems, Second Edition : Methodology, Modeling, and Techniques

---